Nikiya Anton Bettey 2021: 09-18-2021-0414 - Psuedoforce Notes Drafting

Saturday, September 18, 2021

09-18-2021-0414 - Psuedoforce Notes Drafting

A fictitious force (also called a pseudo force,^[1] d'Alembert force,^[2]^[3] or inertial force^[4]^[5]) is a force that appears to act on a mass whose motion is described using a non-inertial frame of reference, such as an accelerating or rotating reference frame. An example is seen in a passenger vehicle that is accelerating in the forward direction – passengers perceive that they are acted upon by a force in the rearward direction pushing them back into their seats. An example in a rotating reference frame is the force that appears to push objects outwards towards the rim of a centrifuge. These apparent forces are examples of fictitious forces.

The fictitious force F is due to an object's inertia when the reference frame does not move inertially, and thus begins to accelerate relative to the free object. The fictitious force thus does not arise from any physical interaction between two objects, such as electromagnetism or contact forces, but rather from the acceleration a of the non-inertial reference frame itself, which from the viewpoint of the frame now appears to be an acceleration of the object instead, requiring a "force" to make this happen. As stated by Iro:^[6]^[7]

Such an additional force due to nonuniform relative motion of two reference frames is called a pseudo-force.
— H. Iro in A Modern Approach to Classical Mechanics p. 180

Assuming Newton's second law in the form F = ma, fictitious forces are always proportional to the mass m.

The fictitious force on an object arises as an imaginary influence, when the frame of reference used to describe the object's motion is accelerating compared to a non-accelerating frame. The fictitious force "explains," using Newton's mechanics, why an object does not follow Newton's laws and "floats freely" as if weightless. As a frame can accelerate in any arbitrary way, so can fictitious forces be as arbitrary (but only in direct response to the acceleration of the frame). However, four fictitious forces are defined for frames accelerated in commonly occurring ways: one caused by any relative acceleration of the origin in a straight line (rectilinear acceleration);^[8] two involving rotation: centrifugal force and Coriolis force; and a fourth, called the Euler force, caused by a variable rate of rotation, should that occur.

Gravitational force would also be a fictitious force based upon a field model in which particles distort spacetime due to their mass, such as general relativity.

Figure 5: Crossing a rotating carousel walking at constant speed from the center of the carousel to its edge, a spiral is traced out in the inertial frame, while a simple straight radial path is seen in the frame of the carousel.

https://en.wikipedia.org/wiki/Fictitious_force

In classical mechanics, the Euler force is the fictitious tangential force^[1] that appears when a non-uniformly rotating reference frame is used for analysis of motion and there is variation in the angular velocity of the reference frame's axes. The Euler acceleration (named for Leonhard Euler), also known as azimuthal acceleration^[2] or transverse acceleration^[3] is that part of the absolute acceleration that is caused by the variation in the angular velocity of the reference frame.^[4]

https://en.wikipedia.org/wiki/Euler_force

In physics, a gravitational field is a model used to explain the influences that a massive body extends into the space around itself, producing a force on another massive body.^[1] Thus, a gravitational field is used to explain gravitational phenomena, and is measured in newtons per kilogram (N/kg). In its original concept, gravity was a force between point masses. Following Isaac Newton, Pierre-Simon Laplace attempted to model gravity as some kind of radiation field or fluid, and since the 19th century, explanations for gravity have usually been taught in terms of a field model, rather than a point attraction.

In a field model, rather than two particles attracting each other, the particles distort spacetime via their mass, and this distortion is what is perceived and measured as a "force".^{[citation needed]} In such a model one states that matter moves in certain ways in response to the curvature of spacetime,^[2] and that there is either no gravitational force,^[3] or that gravity is a fictitious force.^[4]

Gravity is distinguished from other forces by its obedience to the equivalence principle.

https://en.wikipedia.org/wiki/Gravitational_field

In Newtonian mechanics, the centrifugal force is an inertial force (also called a "fictitious" or "pseudo" force) that appears to act on all objects when viewed in a rotating frame of reference. It is directed away from an axis which is parallel to the axis of rotation and passing through the coordinate system's origin. If the axis of rotation passes through the coordinate system's origin, the centrifugal force is directed radially outwards from that axis. The magnitude of centrifugal force F on an object of mass m at the distance r from the origin of a frame of reference rotating with angular velocity ω is:

F=m\omega ^{2}r

The concept of centrifugal force can be applied in rotating devices, such as centrifuges, centrifugal pumps, centrifugal governors, and centrifugal clutches, and in centrifugal railways, planetary orbits and banked curves, when they are analyzed in a rotating coordinate system.

Confusingly, the term has sometimes also been used for the reactive centrifugal force, a real inertial-frame-independent Newtonian force that exists as a reaction to a centripetal force.

https://en.wikipedia.org/wiki/Centrifugal_force

In classical mechanics, a reactive centrifugal force forms part of an action–reaction pair with a centripetal force.

In accordance with Newton's first law of motion, an object moves in a straight line in the absence of any external forces acting on the object. A curved path may however ensue when a force acts on it; this force is often called a centripetal force, as it is directed toward the center of curvature of the path. Then in accordance with Newton's third law of motion, there will also be an equal and opposite force exerted by the object on some other object,^[1]^[2]such as a constraint that forces the path to be curved, and this reaction force, the subject of this article, is sometimes called a reactive centrifugal force, as it is directed in the opposite direction of the centripetal force.

Unlike the inertial force or fictitious force known as centrifugal force, which always exists in addition to the reactive force in the rotating frame of reference, the reactive force is a real Newtonian force that is observed in any reference frame. The two forces will only have the same magnitude in the special cases where circular motion arises and where the axis of rotation is the origin of the rotating frame of reference. It is the reactive force that is the subject of this article.^[3]^[4]^[5]^[6]

https://en.wikipedia.org/wiki/Reactive_centrifugal_force

In physics, the Coriolis force is an inertial or fictitious force^[1] that acts on objects that are in motion within a frame of reference that rotates with respect to an inertial frame. In a reference frame with clockwise rotation, the force acts to the left of the motion of the object. In one with anticlockwise (or counterclockwise) rotation, the force acts to the right. Deflection of an object due to the Coriolis force is called the Coriolis effect. Though recognized previously by others, the mathematical expression for the Coriolis force appeared in an 1835 paper by French scientist Gaspard-Gustave de Coriolis, in connection with the theory of water wheels.^[2] Early in the 20th century, the term Coriolis force began to be used in connection with meteorology.

https://en.wikipedia.org/wiki/Coriolis_force#Tossed_ball_on_a_rotating_carousel

https://en.wikipedia.org/wiki/Ultracentrifuge

https://en.wikipedia.org/wiki/Lamm_equation

https://en.wikipedia.org/wiki/Geodesics_in_general_relativity

https://en.wikipedia.org/wiki/Absolute_space_and_time

The Foucault pendulum or Foucault's pendulum is a simple device named after French physicist Léon Foucault and conceived as an experiment to demonstrate the Earth's rotation. The pendulum was introduced in 1851 and was the first experiment to give simple, direct evidence of the Earth's rotation. Foucault pendulums today are popular displays in science museums and universities.^[1]

https://en.wikipedia.org/wiki/Foucault_pendulum

Isaac Newton's rotating spheres argument attempts to demonstrate that true rotational motion can be defined by observing the tension in the string joining two identical spheres. The basis of the argument is that all observers make two observations: the tension in the string joining the bodies (which is the same for all observers) and the rate of rotation of the spheres (which is different for observers with differing rates of rotation). Only for the truly non-rotating observer will the tension in the string be explained using only the observed rate of rotation. For all other observers a "correction" is required (a centrifugal force) that accounts for the tension calculated being different from the one expected using the observed rate of rotation.^[1] It is one of five arguments from the "properties, causes, and effects" of true motion and rest that support his contention that, in general, true motion and rest cannot be defined as special instances of motion or rest relative to other bodies, but instead can be defined only by reference to absolute space. Alternatively, these experiments provide an operational definition of what is meant by "absolute rotation", and do not pretend to address the question of "rotation relative to what?"^[2] General relativity dispenses with absolute space and with physics whose cause is external to the system, with the concept of geodesics of spacetime.^[3]

Newton was concerned to address the problem of how it is that we can experimentally determine the true motions of bodies in light of the fact that absolute space is not something that can be perceived. Such determination, he says, can be accomplished by observing the causes of motion (that is, forces) and not simply the apparent motions of bodies relative to one another (as in the bucket argument).

https://en.wikipedia.org/wiki/Rotating_spheres

In physics, circular motion is a movement of an object along the circumference of a circle or rotation along a circular path. It can be uniform, with constant angular rate of rotation and constant speed, or non-uniform with a changing rate of rotation. The rotation around a fixed axis of a three-dimensional body involves circular motion of its parts. The equations of motion describe the movement of the center of mass of a body. In circular motion, the distance between the body and a fixed point on the surface remains the same.

Examples of circular motion include: an artificial satellite orbiting the Earth at a constant height, a ceiling fan's blades rotating around a hub, a stone which is tied to a rope and is being swung in circles, a car turning through a curve in a race track, an electron moving perpendicular to a uniform magnetic field, and a gear turning inside a mechanism.

Since the object's velocity vector is constantly changing direction, the moving object is undergoing acceleration by a centripetal force in the direction of the center of rotation. Without this acceleration, the object would move in a straight line, according to Newton's laws of motion.

In physics, uniform circular motion describes the motion of a body traversing a circular path at constant speed. Since the body describes circular motion, its distance from the axis of rotation remains constant at all times. Though the body's speed is constant, its velocity is not constant: velocity, a vector quantity, depends on both the body's speed and its direction of travel. This changing velocity indicates the presence of an acceleration; this centripetal acceleration is of constant magnitude and directed at all times towards the axis of rotation. This acceleration is, in turn, produced by a centripetal force which is also constant in magnitude and directed towards the axis of rotation.

In the case of rotation around a fixed axis of a rigid body that is not negligibly small compared to the radius of the path, each particle of the body describes a uniform circular motion with the same angular velocity, but with velocity and acceleration varying with the position with respect to the axis.

https://en.wikipedia.org/wiki/Circular_motion

A centripetal force (from Latin centrum, "center" and petere, "to seek"^[1]) is a force that makes a body follow a curved path. Its direction is always orthogonal to the motion of the body and towards the fixed point of the instantaneous center of curvature of the path. Isaac Newton described it as "a force by which bodies are drawn or impelled, or in any way tend, towards a point as to a centre".^[2] In Newtonian mechanics, gravity provides the centripetal force causing astronomical orbits.

One common example involving centripetal force is the case in which a body moves with uniform speed along a circular path. The centripetal force is directed at right angles to the motion and also along the radius towards the centre of the circular path.^[3]^[4] The mathematical description was derived in 1659 by the Dutch physicist Christiaan Huygens.^[5]

https://en.wikipedia.org/wiki/Centripetal_force

https://en.wikipedia.org/wiki/Torque

https://en.wikipedia.org/wiki/Acceleration

https://en.wikipedia.org/wiki/Velocity

https://en.wikipedia.org/wiki/Force

https://en.wikipedia.org/wiki/Energy

https://en.wikipedia.org/wiki/Time

https://en.wikipedia.org/wiki/Speed

https://en.wikipedia.org/wiki/Space

https://en.wikipedia.org/wiki/Distance

https://en.wikipedia.org/wiki/Orientation_(geometry)

https://en.wikipedia.org/wiki/Scalar_(physics)

https://en.wikipedia.org/wiki/Scalar_field

https://en.wikipedia.org/wiki/Spinor_field

https://en.wikipedia.org/wiki/Gradient

https://en.wikipedia.org/wiki/Spinor_field

https://en.wikipedia.org/wiki/Spin_structure#Spin_structures_on_vector_bundles

https://en.wikipedia.org/wiki/Magnitude

https://en.wikipedia.org/wiki/Direction

https://en.wikipedia.org/wiki/Vector_(mathematics_and_physics)

https://en.wikipedia.org/wiki/Synthetic_geometry

https://en.wikipedia.org/wiki/Spin_geometry

https://en.wikipedia.org/wiki/Frame_bundle#Orthonormal_frame_bundle

https://en.wikipedia.org/wiki/Trigonometry

https://en.wikipedia.org/wiki/Calculus

https://en.wikipedia.org/wiki/Linear_algebra

https://en.wikipedia.org/wiki/Topological_space

https://en.wikipedia.org/wiki/Set_theory

https://en.wikipedia.org/wiki/Number_theory

https://en.wikipedia.org/wiki/Bayesian_statistics

https://en.wikipedia.org/wiki/Probability_distribution

https://en.wikipedia.org/wiki/Probability_theory

https://en.wikipedia.org/wiki/Statistics

https://en.wikipedia.org/wiki/List_of_equations

https://en.wikipedia.org/wiki/List_of_common_physics_notations

https://en.wikipedia.org/wiki/Greek_letters_used_in_mathematics,_science,_and_engineering

https://en.wikipedia.org/wiki/Zero-dimensional_space

https://en.wikipedia.org/wiki/N-sphere

https://en.wikipedia.org/wiki/Tesseract

https://en.wikipedia.org/wiki/Fractal

https://en.wikipedia.org/wiki/Dimension

https://en.wikipedia.org/wiki/Glossary_of_topology

https://en.wikipedia.org/wiki/Hausdorff_dimension

https://en.wikipedia.org/wiki/Point-finite_collection

https://en.wikipedia.org/wiki/Fluid_dynamics

https://en.wikipedia.org/wiki/Elasticity_(physics)

https://en.wikipedia.org/wiki/Surface_tension

https://en.wikipedia.org/wiki/Shear_stress

https://en.wikipedia.org/wiki/Thermodynamic_free_energy

https://en.wikipedia.org/wiki/Wulff_construction

https://en.wikipedia.org/wiki/Momentum

https://en.wikipedia.org/wiki/Angular_momentum

https://en.wikipedia.org/wiki/Hydrostatics

https://en.wikipedia.org/wiki/Supercritical_fluid

https://en.wikipedia.org/wiki/Hydrostatics

https://en.wikipedia.org/wiki/Pressure

https://en.wikipedia.org/wiki/Adhesion

https://en.wikipedia.org/wiki/Plasma_(physics)

https://en.wikipedia.org/wiki/Rheology

https://en.wikipedia.org/wiki/Continuum_mechanics

https://en.wikipedia.org/wiki/Shear_force

https://en.wikipedia.org/wiki/Solid_mechanics

https://en.wikipedia.org/wiki/Cauchy_stress_tensor#Stress_deviator_tensor

https://en.wikipedia.org/wiki/Critical_plane_analysis

https://en.wikipedia.org/wiki/Deformation_(physics)

https://en.wikipedia.org/wiki/Category:Fracture_mechanics

https://en.wikipedia.org/wiki/Category:Materials_degradation

https://en.wikipedia.org/wiki/Category:Mechanical_failure

https://en.wikipedia.org/wiki/Christensen_failure_criterion

https://en.wikipedia.org/wiki/Fluid

https://en.wikipedia.org/wiki/Scientific_law

https://en.wikipedia.org/wiki/Aerodynamics

https://en.wikipedia.org/wiki/Compressible_flow

https://en.wikipedia.org/wiki/Incompressible_flow

https://en.wikipedia.org/wiki/Isentropic_process

https://en.wikipedia.org/wiki/Thermodynamics

https://en.wikipedia.org/wiki/Hygroscopic_cycle

https://en.wikipedia.org/wiki/Hygroscopy

https://en.wikipedia.org/wiki/Desiccant

https://en.wikipedia.org/wiki/Wax_fire

https://en.wikipedia.org/wiki/Cyclic_ozone

https://en.wikipedia.org/wiki/Cyaphide

https://en.wikipedia.org/wiki/Cyanogen

https://en.wikipedia.org/wiki/Copper(II)_sulfate

https://en.wikipedia.org/wiki/Dicyanoacetylene

https://en.wikipedia.org/wiki/Oxamide

https://en.wikipedia.org/wiki/Graphite

https://en.wikipedia.org/wiki/Beryllium

https://en.wikipedia.org/wiki/Barium

https://en.wikipedia.org/wiki/Caesium

https://en.wikipedia.org/wiki/Rubidium

https://en.wikipedia.org/wiki/Cobalt

https://en.wikipedia.org/wiki/Cobalt_blue

https://en.wikipedia.org/wiki/Prussian_blue

https://en.wikipedia.org/wiki/Cyanide

https://en.wikipedia.org/wiki/Colloid

https://en.wikipedia.org/wiki/Ferrocyanide

https://en.wikipedia.org/wiki/Phosphorus

https://en.wikipedia.org/wiki/Phosphorous

https://en.wikipedia.org/wiki/Thallium#Thallium(I)

https://en.wikipedia.org/wiki/Protium

https://en.wikipedia.org/wiki/Neutronium

https://en.wikipedia.org/wiki/Strange_matter

https://en.wikipedia.org/wiki/Neutron_magnetic_moment

https://en.wikipedia.org/wiki/R-process

https://en.wikipedia.org/wiki/Neutron_microscope

https://en.wikipedia.org/wiki/Mirror_nuclei

https://en.wikipedia.org/wiki/Neutron_generator

https://en.wikipedia.org/wiki/Mirror_matter

https://en.wikipedia.org/wiki/Neutron_supermirror

https://en.wikipedia.org/wiki/Neutron_emission

https://en.wikipedia.org/wiki/Weak_interaction

https://en.wikipedia.org/wiki/Neutron–proton_ratio

https://en.wikipedia.org/wiki/Fusion_power#Magnetic_Mirror

https://en.wikipedia.org/wiki/Nuclear_drip_line

https://en.wikipedia.org/wiki/Table_of_nuclides

https://en.wikipedia.org/wiki/Valley_of_stability

https://en.wikipedia.org/wiki/Beta-decay_stable_isobars

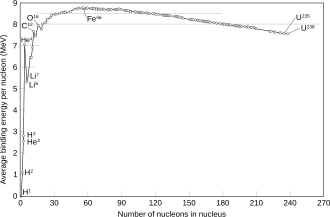
https://en.wikipedia.org/wiki/Nuclear_binding_energy

https://en.wikipedia.org/wiki/Neutron_magnetic_moment

https://en.wikipedia.org/wiki/Positron_emission

https://en.wikipedia.org/wiki/Nuclear_fission_product

https://en.wikipedia.org/wiki/Radioactive_waste

https://en.wikipedia.org/wiki/Compact_toroid

Fusion power is a proposed form of power generation that would generate electricity by using heat from nuclear fusion reactions. In a fusion process, two lighter atomic nuclei combine to form a heavier nucleus, while releasing energy. Devices designed to harness this energy are known as fusion reactors.

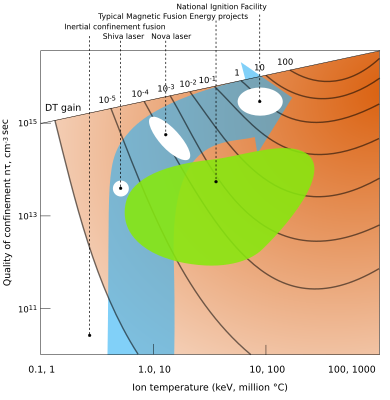
Fusion processes require fuel and a confined environment with sufficient temperature, pressure, and confinement time to create a plasma in which fusion can occur. The combination of these figures that results in a power-producing system is known as the Lawson criterion. In stars, the most common fuel is hydrogen, and gravity provides extremely long confinement times that reach the conditions needed for fusion energy production. Proposed fusion reactors generally use hydrogen isotopes such as deuterium and tritium (and especially a mixture of the two), which react more easily than hydrogen to allow them to reach the Lawson criterion requirements with less extreme conditions. Most designs aim to heat their fuel to around 100 million degrees, which presents a major challenge in producing a successful design.

As a source of power, nuclear fusion is expected to have many advantages over fission. These include reduced radioactivity in operation and little high-level nuclear waste, ample fuel supplies, and increased safety. However, the necessary combination of temperature, pressure, and duration has proven to be difficult to produce in a practical and economical manner. Research into fusion reactors began in the 1940s, but to date, no design has produced more fusion power output than the electrical power input.^[1] A second issue that affects common reactions is managing neutrons that are released during the reaction, which over time degrade many common materials used within the reaction chamber.

Fusion researchers have investigated various confinement concepts. The early emphasis was on three main systems: z-pinch, stellarator, and magnetic mirror. The current leading designs are the tokamak and inertial confinement (ICF) by laser. Both designs are under research at very large scales, most notably the ITER tokamak in France, and the National Ignition Facility (NIF) laser in the United States. Researchers are also studying other designs that may offer cheaper approaches. Among these alternatives, there is increasing interest in magnetized target fusion and inertial electrostatic confinement, and new variations of the stellarator.

Background[edit]

The Sun, like other stars, is a natural fusion reactor, where stellar nucleosynthesis transforms lighter elements into heavier elements with the release of energy.

Binding energy for different atomic nuclei. Iron-56 has the highest, making it the most stable. Nuclei to the left are likely to release energy when they fuse (fusion); those to the far right are likely to be unstable and release energy when they split (fission).

Mechanism[edit]

Fusion reactions occur when two or more atomic nuclei come close enough for long enough that the nuclear force pulling them together exceeds the electrostatic force pushing them apart, fusing them into heavier nuclei. For nuclei heavier than iron-56, the reaction is endothermic, requiring an input of energy.^[2] The heavy nuclei bigger than iron have many more protons resulting in a greater repulsive force. For nuclei lighter than iron-56, the reaction is exothermic, releasing energy when they fuse. Since hydrogen has a single proton in its nucleus, it requires the least effort to attain fusion, and yields the most net energy output. Also since it has one electron, hydrogen is the easiest fuel to fully ionize.

The strong force acts only over short distances (at most one femtometer, the diameter of one proton or neutron), while the repulsive electrostatic force between nuclei acts over longer distances. In order to undergo fusion, the fuel atoms need to be given enough kinetic energy to approach each other closely enough for the strong force to overcome the electrostatic repulsion. The amount of kinetic energyneeded to bring the fuel atoms close enough is known as the "Coulomb barrier". Ways of providing this energy include speeding up atoms in a particle accelerator, or heating them to high temperatures.

Once an atom is heated above its ionization energy, its electrons are stripped away, leaving just the bare nucleus. This process is known as ionization, and the resulting nucleus is known as an ion. The result is a hot cloud of ions and free electrons formerly attached to them known as plasma. Because the charges are separated, plasmas are electrically conductive and magnetically controllable. Many fusion devices take advantage of this to confine the particles as they are heated.

Cross section[edit]

The fusion reaction rate increases rapidly with temperature until it maximizes and then gradually drops off. The deuterium-tritium fusion rate peaks at a lower temperature (about 70 keV, or 800 million kelvin) and at a higher value than other reactions commonly considered for fusion energy.

A reaction's cross section, denoted σ, measures the probability that a fusion reaction will happen. This depends on the relative velocity of the two nuclei. Higher relative velocities generally increase the probability, but the probability begins to decrease again at very high energies.^[3]

In a plasma, particle velocity can be characterized using a probability distribution. If the plasma is thermalized, the distribution looks like a Gaussian curve, or Maxwell–Boltzmann distribution. In this case, it is useful to use the average particle cross section over the velocity distribution. This is entered into the volumetric fusion rate:^[4]

P_{\text{fusion}}=n_{A}n_{B}\langle \sigma v_{A,B}\rangle E_{\text{fusion}}

where:

$P_{\text{fusion}}$ is the energy made by fusion, per time and volume
n is the number density of species A or B, of the particles in the volume
$\langle \sigma v_{A,B}\rangle$ is the cross section of that reaction, average over all the velocities of the two species v
$E_{\text{fusion}}$ is the energy released by that fusion reaction.

Lawson criterion[edit]

The Lawson criterion shows how energy output varies with temperature, density, speed of collision for any given fuel. This equation was central to John Lawson's analysis of fusion working with a hot plasma. Lawson assumed an energy balance, shown below.^[4]

P_\text{out} = \eta_\text{capture}\left(P_\text{fusion} - P_\text{conduction} - P_\text{radiation}\right)

η, efficiency
$P_{\text{conduction}}$ , conduction losses as energetic mass leaves the plasma
$P_{\text{radiation}}$ , radiation losses as energy leaves as light
$P_{{\text{out}}}$ , net power from fusion
$P_{\text{fusion}}$ , is rate of energy generated by the fusion reactions.

Plasma clouds lose energy through conduction and radiation.^[4] Conduction occurs when ions, electrons, or neutrals impact other substances, typically a surface of the device, and transfer a portion of their kinetic energy to the other atoms. Radiation is energy that leaves the cloud as light. Radiation increases with temperature. Fusion power technologies must overcome these losses.

Triple product: density, temperature, time[edit]

The Lawson criterion argues that a machine holding a thermalized and quasi-neutral plasma has to generate enough energy to overcome its energy losses. The amount of energy released in a given volume is a function of the temperature, and thus the reaction rate on a per-particle basis, the density of particles within that volume, and finally the confinement time, the length of time that energy stays within the volume.^[4]^[5] This is known as the "triple product": the plasma density, temperature, and confinement time.^[6]

In magnetic confinement, the density is low, on the order of a "good vacuum". For instance, in the ITER device the fuel density is about 10 x 10¹⁹, which is about one-millionth atmospheric density.^[7] This means that the temperature and/or confinement time must increase. Fusion-relevant temperatures have been achieved using a variety of heating methods that were developed in the early 1970s. In modern machines, as of 2019, the major remaining issue was the confinement time. Plasmas in strong magnetic fields are subject to a number of inherent instabilities, which must be suppressed to reach useful durations. One way to do this is to simply make the reactor volume larger, which reduces the rate of leakage due to classical diffusion. This is why ITER is so large.

In contrast, inertial confinement systems approach useful triple product values via higher density, and have short confinement intervals. In NIF, the initial frozen hydrogen fuel load has a density less than water that is increased to about 100 times the density of lead. In these conditions, the rate of fusion is so high that the fuel fuses in the microseconds it takes for the heat generated by the reactions to blow the fuel apart. Although NIF is also large, this is a function of its "driver" design, not inherent to the fusion process.

Energy capture[edit]

Multiple approaches have been proposed to capture the energy that fusion produces. The simplest is to heat a fluid. The commonly targeted D-T reaction releases much of its energy as fast-moving neutrons. Electrically neutral, the neutron is unaffected by the confinement scheme. In most such designs, it is captured in a thick "blanket" of lithium surrounding the reactor core. When struck by a high-energy neutron, the blanket heats up. It is then actively cooled with a working fluid that drives a turbine to produce power.

Another design proposed to use the neutrons to breed fission fuel in a blanket of nuclear waste, a concept known as a fission-fusion hybrid. In these systems, the power output is enhanced by the fission events, and power is extracted using systems like those in conventional fission reactors.^[8]

Designs that use other fuels, notably the proton-boron aneutronic fusion reaction, release much more of their energy in the form of charged particles. In these cases, power extraction systems based on the movement of these charges are possible. Direct energy conversion was developed at Lawrence Livermore National Laboratory (LLNL) in the 1980s as a method to maintain a voltage directly using fusion reaction products. This has demonstrated energy capture efficiency of 48 percent.^[9]

Methods[edit]

Plasma behavior[edit]

Plasma is an ionized gas that conducts electricity.^[10] In bulk, it is modeled using magnetohydrodynamics, which is a combination of the Navier–Stokes equations governing fluids and Maxwell's equations governing how magnetic and electric fields behave.^[11] Fusion exploits several plasma properties, including:

Self-organizing plasma conducts electric and magnetic fields. Its motions generate fields that can in turn contain it.^[12]
Diamagnetic plasma can generate its own internal magnetic field. This can reject an externally applied magnetic field, making it diamagnetic.^[13]
Magnetic mirrors can reflect plasma when it moves from a low to high density field.^[14]^:24

Magnetic confinement[edit]

Tokamak: the most well-developed and well-funded approach. This method drives hot plasma around in a magnetically confined torus, with an internal current. When completed, ITER will become the world's largest tokamak. As of April 2012 an estimated 215 experimental tokamaks were either planned, decommissioned or operating (35) worldwide.^[15]
Spherical tokamak: also known as spherical torus. A variation on the tokamak with a spherical shape.
Stellarator: Twisted rings of hot plasma. The stellarator attempts to create a natural twisted plasma path, using external magnets. Stellarators were developed by Lyman Spitzer in 1950 and evolved into four designs: Torsatron, Heliotron, Heliac and Helias. One example is Wendelstein 7-X, a German device. It is the world's largest stellarator.^[16]
Internal rings: Stellarators create a twisted plasma using external magnets, while tokamaks do so using a current induced in the plasma. Several classes of designs provide this twist using conductors inside the plasma. Early calculations showed that collisions between the plasma and the supports for the conductors would remove energy faster than fusion reactions could replace it. Modern variations, including the Levitated Dipole Experiment (LDX), use a solid superconducting torus that is magnetically levitated inside the reactor chamber.^[17]
Magnetic mirror: Developed by Richard F. Post and teams at LLNL in the 1960s.^[18] Magnetic mirrors reflect plasma back and forth in a line. Variations included the Tandem Mirror, magnetic bottle and the biconic cusp.^[19] A series of mirror machines were built by the US government in the 1970s and 1980s, principally at LLNL.^[20] However, calculations in the 1970s estimated it was unlikely these would ever be commercially useful.
Bumpy torus: A number of magnetic mirrors are arranged end-to-end in a toroidal ring. Any fuel ions that leak out of one are confined in a neighboring mirror, permitting the plasma pressure to be raised arbitrarily high without loss. An experimental facility, the ELMO Bumpy Torus or EBT was built and tested at Oak Ridge National Laboratory (ORNL) in the 1970s.
Field-reversed configuration: This device traps plasma in a self-organized quasi-stable structure; where the particle motion makes an internal magnetic field which then traps itself.^[21]
Spheromak: Similar to a field-reversed configuration, a semi-stable plasma structure made by using the plasmas' self-generated magnetic field. A spheromak has both toroidal and poloidal fields, while a field-reversed configuration has no toroidal field.^[22]
Reversed field pinch: Here the plasma moves inside a ring. It has an internal magnetic field. Moving out from the center of this ring, the magnetic field reverses direction.

Inertial confinement[edit]

Indirect drive: Lasers heat a structure known as a Hohlraum that becomes so hot it begins to radiate x-ray light. These x-rays heat a fuel pellet, causing it to collapse inward to compress the fuel. The largest system using this method is the National Ignition Facility, followed closely by Laser Mégajoule.^[23]
Direct drive: Lasers directly heat the fuel pellet. Notable direct drive experiments have been conducted at the Laboratory for Laser Energetics (LLE) and the GEKKO XII facilities. Good implosions require fuel pellets with close to a perfect shape in order to generate a symmetrical inward shock wave that produces the high-density plasma.
Fast ignition: This method uses two laser blasts. The first blast compresses the fusion fuel, while the second ignites it. As of 2019 this technique had lost favor for energy production.^[24]
Magneto-inertial fusion or Magnetized Liner Inertial Fusion: This combines a laser pulse with a magnetic pinch. The pinch community refers to it as magnetized liner inertial fusion while the ICF community refers to it as magneto-inertial fusion.^[25]
Ion Beams: Ion beams replace laser beams to heat the fuel.^[26] The main difference is that the beam has momentum due to mass, whereas lasers do not. As of 2019 it appears unlikely that ion beams can be sufficiently focused spatially and in time.
Z-machine: Sends an electrical current through thin tungsten wires, heating them sufficiently to generate x-rays. Like the indirect drive approach, these x-rays then compress a fuel capsule.

Magnetic or electric pinches[edit]

Z-Pinch: A current travels in the z-direction through the plasma. The current generates a magnetic field that compresses the plasma. Pinches were the first method for man-made controlled fusion.^[27]^[28] The z-pinch has inherent instabilities that limit its compression and heating to values too low for practical fusion. The largest such machine, the UK's ZETA, was the last major experiment of the sort. The problems in z-pinch led to the tokamak design. The dense plasma focus is a possibly superior variation.
Theta-Pinch: A current circles around the outside of a plasma column, in the theta direction. This induces a magnetic field running down the center of the plasma, as opposed to around it. The early theta-pinch device Scylla was the first to conclusively demonstrate fusion, but later work demonstrated it had inherent limits that made it uninteresting for power production.
Sheared Flow Stabilized Z-Pinch: Research at the University of Washington under Uri Shumlak investigated the use of sheared-flow stabilization to smooth out the instabilities of Z-pinch reactors. This involves accelerating neutral gas along the axis of the pinch. Experimental machines included the FuZE and Zap Flow Z-Pinch experimental reactors.^[29] In 2017, Conway, Nelson and Shumlak co-founded Zap Energy to attempt to commercialize the technology for power production.^[30]^[31]^[32]
Screw Pinch: This method combines a theta and z-pinch for improved stabilization.^[33]

Inertial electrostatic confinement[edit]

Fusor: An electric field heats ions to fusion conditions. The machine typically uses two spherical cages, a cathode inside the anode, inside a vacuum. These machines are not considered a viable approach to net power because of their high conduction and radiation losses.^[34] They are simple enough to build that amateurs have fused atoms using them.^[35]
Polywell: Attempts to combine magnetic confinement with electrostatic fields, to avoid the conduction losses generated by the cage.^[36]

Other[edit]

Magnetized target fusion: Confines hot plasma using a magnetic field and squeezes it using inertia. Examples include LANL FRX-L machine,^[37] General Fusion (piston compression with liquid metal liner), HyperJet Fusion (plasma jet compression with plasma liner).^[38]^[39]
Uncontrolled: Fusion has been initiated by man, using uncontrolled fission explosions to stimulate fusion. Early proposals for fusion power included using bombs to initiate reactions. See Project PACER.
Beam fusion: A beam of high energy particles fired at another beam or target can initiate fusion. This was used in the 1970s and 1980s to study the cross sections of fusion reactions.^[3]However beam systems cannot be used for power because keeping a beam coherent takes more energy than comes from fusion.
Muon-catalyzed fusion: This approach replaces electrons in diatomic molecules of isotopes of hydrogen with muons - more massive particles with the same electric charge. Their greater mass compresses the nuclei enough such that the strong interaction can cause fusion.^[40] As of 2007 producing muons required more energy than can be obtained from muon-catalyzed fusion.^[41]

Common tools[edit]

Many approaches, equipment, and mechanisms are employed across multiple projects to address fusion heating, measurement, and power production.^[42]

Heating[edit]

Electrostatic heating: an electric field can do work on charged ions or electrons, heating them.^[43]
Neutral beam injection: hydrogen is ionized and accelerated by an electric field to form a charged beam that is shone through a source of neutral hydrogen gas towards the plasma which itself is ionized and contained by a magnetic field. Some of the intermediate hydrogen gas is accelerated towards the plasma by collisions with the charged beam while remaining neutral: this neutral beam is thus unaffected by the magnetic field and so reaches the plasma. Once inside the plasma the neutral beam transmits energy to the plasma by collisions which ionize it and allow it to be contained by the magnetic field, thereby both heating and refueling the reactor in one operation. The remainder of the charged beam is diverted by magnetic fields onto cooled beam dumps.^[44]
Radio frequency heating: a radio wave causes the plasma to oscillate (i.e., microwave oven). This is also known as electron cyclotron resonance heating or dielectric heating.^[45]
Magnetic reconnection: when plasma gets dense, its electromagnetic properties can change, which can lead to magnetic reconnection. Reconnection helps fusion because it instantly dumps energy into a plasma, heating it quickly. Up to 45% of the magnetic field energy can heat the ions.^[46]^[47]
Magnetic oscillations: varying electrical currents can be supplied to magnetic coils that heat plasma confined within a magnetic wall.^[48]
Antiproton annihilation: antiprotons injected into a mass of fusion fuel can induce thermonuclear reactions. This possibility as a method of spacecraft propulsion, known as antimatter-catalyzed nuclear pulse propulsion, was investigated at Pennsylvania State University in connection with the proposed AIMStar project.^{[citation needed]}

Measurement[edit]

Flux loop: a loop of wire is inserted into the magnetic field. As the field passes through the loop, a current is made. The current measures the total magnetic flux through that loop. This has been used on the National Compact Stellarator Experiment,^[49] the polywell,^[50] and the LDX machines. A Langmuir probe, a metal object placed in a plasma, can be employed. A potential is applied to it, giving it a voltage against the surrounding plasma. The metal collects charged particles, drawing a current. As the voltage changes, the current changes. This makes an IV Curve. The IV-curve can be used to determine the local plasma density, potential and temperature.^[51]
Thomson scattering: Light scatters from plasma that can be used to reconstruct plasma behavior, including density and temperature. It is common in Inertial confinement fusion,^[52]Tokamaks,^[53] and fusors. In ICF systems, firing a second beam into a gold foil adjacent to the target makes x-rays that traverse the plasma. In tokamaks, this can be done using mirrors and detectors to reflect light.
Neutron detectors: Several types of neutron detectors can record the rate at which neutrons are produced.^[54]^[55]
X-ray detectors Visible, IR, UV, and X-rays are emitted anytime a particle changes velocity.^[56] If the reason is deflection by a magnetic field, the radiation is cyclotron radiation at low speeds and synchrotron radiation at high speeds. If the reason is deflection by another particle, plasma radiates X-rays, known as Bremsstrahlung radiation.^[57]

Power production[edit]

Neutron blankets absorb neutrons, which heats the blanket. Power can be extracted from the blanket in various ways:

Steam turbines can be driven by heat transferred into a working fluid that turns into steam, driving electric generators.^[58]
Neutron blankets: These neutrons can regenerate spent fission fuel.^[59] Tritium can be produced using a breeder blanket comprised of liquid lithium or a helium cooled pebble bed made of lithium-bearing ceramic pebbles.^[60]
Direct conversion: The kinetic energy of a particle can be converted into voltage.^[18] It was first suggested by Richard F. Post in conjunction with magnetic mirrors, in the late 1960s. It has been proposed for Field-Reversed Configurations as well as Dense Plasma Focus devices. The process converts a large fraction of the random energy of the fusion products into directed motion. The particles are then collected on electrodes at various large electrical potentials. This method has demonstrated an experimental efficiency of 48 percent.^[61]

Confinement[edit]

Parameter space occupied by inertial fusion energy and magnetic fusion energy devices as of the mid 1990s. The regime allowing thermonuclear ignition with high gain lies near the upper right corner of the plot.

Confinement refers to all the conditions necessary to keep a plasma dense and hot long enough to undergo fusion. General principles:

Equilibrium: The forces acting on the plasma must be balanced. One exception is inertial confinement, where the fusion must occur faster than the dispersal time.
Stability: The plasma must be constructed so that disturbances will not lead to the plasma dispersing.
Transport or conduction: The loss of material must be sufficiently slow.^[4] The plasma carries energy off with it, so rapid loss of material will disrupt fusion. Material can be lost by transport into different regions or conduction through a solid or liquid.

To produce self-sustaining fusion, part of the energy released by the reaction must be used to heat new reactants and maintain the conditions for fusion.

Unconfined[edit]

The first human-made, large-scale fusion reaction was the test of the hydrogen bomb, Ivy Mike, in 1952.

Magnetic confinement[edit]

Magnetic Mirror[edit]

Magnetic mirror effect. If a particle follows the field line and enters a region of higher field strength, the particles can be reflected. Several devices apply this effect. The most famous was the magnetic mirror machines, a series of devices built at LLNL from the 1960s to the 1980s.^[62] Other examples include magnetic bottles and Biconic cusp.^[63] Because the mirror machines were straight, they had some advantages over ring-shaped designs. The mirrors were easier to construct and maintain and direct conversion energy capture was easier to implement.^[9] Poor confinement led this approach to be abandoned, except in the polywell design.^[64]

Magnetic Loops[edit]

Magnetic loops bend the field lines back on themselves, either in circles or more commonly in nested toroidal surfaces. The most highly developed systems of this type are the tokamak, the stellarator, and the reversed field pinch. Compact toroids, especially the field-reversed configuration and the spheromak, attempt to combine the advantages of toroidal magnetic surfaces with those of a simply connected (non-toroidal) machine, resulting in a mechanically simpler and smaller confinement area.

Inertial confinement[edit]

Inertial confinement is the use of rapid implosion to heat and confine plasma. A shell surrounding the fuel is imploded using a direct laser blast (direct drive), a secondary x-ray blast (indirect drive), or heavy beams. The fuel must be compressed to about 30 times solid density with energetic beams. Direct drive can in principle be efficient, but insufficient uniformity has prevented success.^[65]^:19-20 Indirect drive uses beams to heat a shell, driving the shell to radiate x-rays, which then implode the pellet. The beams are commonly laser beams, but ion and electron beams have been investigated.^[65]^:182-193

Electrostatic confinement[edit]

Electrostatic confinement fusion devices use electrostatic fields. The best known is the fusor. This device has a cathode inside an anode wire cage. Positive ions fly towards the negative inner cage, and are heated by the electric field in the process. If they miss the inner cage they can collide and fuse. Ions typically hit the cathode, however, creating prohibitory high conduction losses. Fusion rates in fusors are low because of competing physical effects, such as energy loss in the form of light radiation.^[66] Designs have been proposed to avoid the problems associated with the cage, by generating the field using a non-neutral cloud. These include a plasma oscillating device,^[67] a magnetically-shielded-grid,^[68] a penning trap, the polywell,^[69] and the F1 cathode driver concept.^[70]

https://en.wikipedia.org/wiki/Fusion_power#Magnetic_Mirror

https://en.wikipedia.org/wiki/Torus

https://en.wikipedia.org/wiki/Fusion_power#Magnetic_Mirror

https://en.wikipedia.org/wiki/Simply_connected_space

https://en.wikipedia.org/wiki/X-ray

https://en.wikipedia.org/wiki/Inertial_electrostatic_confinement

https://en.wikipedia.org/wiki/Penning_trap

https://en.wikipedia.org/wiki/Polywell

https://en.wikipedia.org/wiki/Lockheed_Martin_Compact_Fusion_Reactor

https://en.wikipedia.org/wiki/Deuterium–tritium_fusion

https://en.wikipedia.org/wiki/Magnetized_target_fusion

The reaction vessel will be a fast-rotating cylinder of liquid metal (lead, incorporating lithium to harvest the tritium formed through neutron activation) which is formed into a sphere by the action of synchronised pistons driven by steam. Magnetized fusion fuel as plasma is injected into the sphere as it contracts, producing sufficient temperature and pressure for the fusion reaction to take place. The liquid metal is circulated through heat exchangers to provide steam.

https://en.wikipedia.org/wiki/Magnetized_target_fusion

https://en.wikipedia.org/wiki/Heat_exchanger

https://en.wikipedia.org/wiki/Field-reversed_configuration

https://en.wikipedia.org/wiki/Superconductivity

https://en.wikipedia.org/wiki/Magneto-inertial_fusion

https://en.wikipedia.org/wiki/Neutronium

https://en.wikipedia.org/wiki/Preon

https://en.wikipedia.org/wiki/Meson_bomb

https://en.wikipedia.org/wiki/Pressuron

https://en.wikipedia.org/wiki/Vertical_pressure_variation

https://en.wikipedia.org/wiki/Hypsometric_equation

https://en.wikipedia.org/wiki/Seabed#Depth

https://en.wikipedia.org/wiki/Marine_sediment

https://en.wikipedia.org/wiki/Trihydrogen_cation

https://en.wikipedia.org/wiki/Three-center_two-electron_bond

https://en.wikipedia.org/wiki/Dark_matter

https://en.wikipedia.org/wiki/Dark_energy

https://en.wikipedia.org/wiki/Lambda-CDM_model

https://en.wikipedia.org/wiki/Inhomogeneous_cosmology

https://en.wikipedia.org/wiki/Prussian_blue

https://en.wikipedia.org/wiki/Hydride

https://en.wikipedia.org/wiki/Hydrogen_ion

https://en.wikipedia.org/wiki/Weakly_interacting_massive_particles

https://en.wikipedia.org/wiki/Inflation_(cosmology)

https://en.wikipedia.org/wiki/Cosmic_microwave_background

https://en.wikipedia.org/wiki/Nitroglycerin

https://en.wikipedia.org/wiki/Glycerol

https://en.wikipedia.org/wiki/Propylene_oxide

https://en.wikipedia.org/wiki/Oxetane

https://en.wikipedia.org/wiki/Diol_cyclization

cyclicization ringulants

https://en.wikipedia.org/wiki/Magnetic_dipole–dipole_interaction

https://en.wikipedia.org/wiki/J-coupling

https://en.wikipedia.org/wiki/Electron_electric_dipole_moment

https://en.wikipedia.org/wiki/Zero-point_energy

https://en.wikipedia.org/wiki/Van_der_Waals_force

https://en.wikipedia.org/wiki/Zero_field_splitting

https://en.wikipedia.org/wiki/Quadrupole

https://en.wikipedia.org/wiki/Quadrupole#Gravitational_quadrupole

https://en.wikipedia.org/wiki/Trace_(linear_algebra)

https://en.wikipedia.org/wiki/Overtone_band

https://en.wikipedia.org/wiki/Near-infrared_spectroscopy

https://en.wikipedia.org/wiki/Doppler_effect

https://en.wikipedia.org/wiki/Inflation_(cosmology)

https://en.wikipedia.org/wiki/Quintessence_(physics)

https://en.wikipedia.org/wiki/Lambda-CDM_model

https://en.wikipedia.org/wiki/Heliosphere#Heliopause

https://en.wikipedia.org/wiki/Entropic_gravity

https://en.wikipedia.org/wiki/Fundamental_interaction

https://en.wikipedia.org/wiki/List_of_particles

https://en.wikipedia.org/wiki/Chameleon_particle

https://en.wikipedia.org/wiki/Axion

https://en.wikipedia.org/wiki/Graviphoton

https://en.wikipedia.org/wiki/Particle_physics

https://en.wikipedia.org/wiki/Condensed_matter_physics

https://en.wikipedia.org/wiki/Particle

https://en.wikipedia.org/wiki/Preon

https://en.wikipedia.org/wiki/Weak_hypercharge

https://en.wikipedia.org/wiki/X_(charge)

https://en.wikipedia.org/wiki/SO(10)

https://en.wikipedia.org/wiki/Grand_Unified_Theory

https://en.wikipedia.org/wiki/Supersymmetry_breaking

https://en.wikipedia.org/wiki/Stochastic_differential_equation

https://en.wikipedia.org/wiki/Gauge_theory

https://en.wikipedia.org/wiki/Eigenvalues_and_eigenvectors

https://en.wikipedia.org/wiki/Zero_element#Additive_identities

https://en.wikipedia.org/wiki/Category:Isotopes_of_neutronium

https://en.wikipedia.org/wiki/Category:Neutron

https://en.wikipedia.org/wiki/Isotopes_of_hydrogen#Hydrogen-1_(Protium)

https://en.wikipedia.org/wiki/Hydrogen

https://en.wikipedia.org/wiki/Proton

https://en.wikipedia.org/wiki/Extended_periodic_table

https://en.wikipedia.org/wiki/Periodic_table

https://en.wikipedia.org/wiki/Alternative_periodic_tables

https://en.wikipedia.org/wiki/Subatomic_particle

https://en.wikipedia.org/wiki/Hypernucleus

https://en.wikipedia.org/wiki/Category:Exotic_matter

https://en.wikipedia.org/wiki/Spinor_condensate

https://en.wikipedia.org/wiki/Spiral

https://en.wikipedia.org/wiki/Circle

https://en.wikipedia.org/wiki/Triangle

https://en.wikipedia.org/wiki/Square

https://en.wikipedia.org/wiki/Point_(geometry)

https://en.wikipedia.org/wiki/Point

https://en.wikipedia.org/wiki/Line

https://en.wikipedia.org/wiki/Tractrix

https://en.wikipedia.org/wiki/Logarithmic_spiral

https://en.wikipedia.org/wiki/Loop_(graph_theory)

https://en.wikipedia.org/wiki/Loop_(topology)

https://en.wikipedia.org/wiki/Free_loop

https://en.wikipedia.org/wiki/Category:Knot_theory

https://en.wikipedia.org/wiki/Infinity

https://en.wikipedia.org/wiki/Mathematical_fallacy

https://en.wikipedia.org/wiki/Infinite_divisibility

https://en.wikipedia.org/wiki/Division_by_zero

https://en.wikipedia.org/wiki/Indeterminate_form#Indeterminate_form_0/0

https://en.wikipedia.org/wiki/Undefined_(mathematics)

https://en.wikipedia.org/wiki/Zero_to_the_power_of_zero

https://en.wikipedia.org/wiki/Category:0_(number)

https://en.wikipedia.org/wiki/Nilpotent

https://en.wikipedia.org/wiki/Zero_of_a_function

https://en.wikipedia.org/wiki/Algebraically_closed_field#Other_properties

https://en.wikipedia.org/wiki/Category:Field_(mathematics)

https://en.wikipedia.org/wiki/Ground_field

https://en.wikipedia.org/wiki/Galois_cohomology

https://en.wikipedia.org/wiki/Profinite_group

https://en.wikipedia.org/wiki/Hilbert%27s_Theorem_90

https://en.wikipedia.org/wiki/Principal_homogeneous_space

https://en.wikipedia.org/wiki/Differentiable_manifold#Definition

https://en.wikipedia.org/wiki/Chain_rule

https://en.wikipedia.org/wiki/Malliavin_calculus

https://en.wikipedia.org/wiki/Stochastic_process

https://en.wikipedia.org/wiki/Gaussian_process

https://en.wikipedia.org/wiki/Gaussian_process_approximations

https://en.wikipedia.org/wiki/Linear_combination

https://en.wikipedia.org/wiki/Euclidean_space

https://en.wikipedia.org/wiki/Dot_product

https://en.wikipedia.org/wiki/Scalar_multiplication

https://en.wikipedia.org/wiki/Empty_set

https://en.wikipedia.org/wiki/Axiom_of_extensionality

https://en.wikipedia.org/wiki/Axiom_of_extensionality#In_set_theory_with_ur-elements

https://en.wikipedia.org/wiki/Axiom_of_regularity

https://en.wikipedia.org/wiki/Axiom_of_pairing

https://en.wikipedia.org/wiki/Gödel%27s_incompleteness_theorems#Second_incompleteness_theorem

https://en.wikipedia.org/wiki/Axiom_schema_of_replacement

https://en.wikipedia.org/wiki/Zermelo_set_theory

https://en.wikipedia.org/wiki/Type_theory

https://en.wikipedia.org/wiki/Ordinal_number

https://en.wikipedia.org/wiki/Limit_ordinal

https://en.wikipedia.org/wiki/Isolated_point

https://en.wikipedia.org/wiki/Topological_space

https://en.wikipedia.org/wiki/Kuratowski_closure_axioms

https://en.wikipedia.org/wiki/Unary_operation

https://en.wikipedia.org/wiki/Arity

https://en.wikipedia.org/wiki/Valency_(linguistics)

https://en.wikipedia.org/wiki/Lambda_calculus

https://en.wikipedia.org/wiki/Absolute_value

https://en.wikipedia.org/wiki/Floor_and_ceiling_functions

https://en.wikipedia.org/wiki/Arity

https://en.wikipedia.org/wiki/Earnshaw%27s_theorem#Proofs_for_magnetic_dipoles

https://en.wikipedia.org/wiki/Electrostatic_levitation

https://en.wikipedia.org/wiki/Magnetic_levitation

https://en.wikipedia.org/wiki/Magnetic_pressure

https://en.wikipedia.org/wiki/Dipole_magnet

https://en.wikipedia.org/wiki/Permeability_(electromagnetism)

https://en.wikipedia.org/wiki/Nonlinear_optics

In a nonlinear medium, the permeability can depend on the strength of the magnetic field. (space)

https://en.wikipedia.org/wiki/Permeability_(electromagnetism)

https://en.wikipedia.org/wiki/Vacuum_permeability

https://en.wikipedia.org/wiki/Centimetre–gram–second_system_of_units

https://en.wikipedia.org/wiki/Magnetostatics

https://en.wikipedia.org/wiki/Servomechanism

https://en.wikipedia.org/wiki/Eddy_current

https://en.wikipedia.org/wiki/Anisotropy

https://en.wikipedia.org/wiki/Magnetic_levitation

https://en.wikipedia.org/wiki/Dimensionless_quantity

https://en.wikipedia.org/wiki/Linear_stability

https://en.wikipedia.org/wiki/Electromagnetic_suspension

https://en.wikipedia.org/wiki/Zippe-type_centrifuge

https://en.wikipedia.org/wiki/Maglev

https://en.wikipedia.org/wiki/Drag_(physics)

https://en.wikipedia.org/wiki/Electrodynamic_suspension

https://en.wikipedia.org/wiki/Lenz%27s_law

https://en.wikipedia.org/wiki/Gyroscope

https://en.wikipedia.org/wiki/Linear_induction_motor

https://en.wikipedia.org/wiki/Pyrolytic_carbon

https://en.wikipedia.org/wiki/Bismuth

https://en.wikipedia.org/wiki/Vacuum_permeability

https://en.wikipedia.org/wiki/Ampère%27s_force_law

https://en.wikipedia.org/wiki/Biot–Savart_law

https://en.wikipedia.org/wiki/Line_integral

https://en.wikipedia.org/wiki/Parametric_equation

https://en.wikipedia.org/wiki/Linear_form

https://en.wikipedia.org/wiki/Flux

https://en.wikipedia.org/wiki/Transport_phenomena

UNIT OPERATIONS

In chemical engineering and related fields, a unit operation is a basic step in a process. Unit operations involve a physical change or chemical transformation such as separation, crystallization, evaporation, filtration, polymerization, isomerization, and other reactions. For example, in milk processing, the following unit operations are involved: homogenization, pasteurization, and packaging. These unit operations are connected to create the overall process. A process may require many unit operations to obtain the desired product from the starting materials, or feedstocks.

Chemical Engineering[edit]

Chemical engineering unit operations consist of five classes:

Fluid flow processes, including fluids transportation, filtration, and solids fluidization.
Heat transfer processes, including evaporation and heat exchange.
Mass transfer processes, including gas absorption, distillation, extraction, adsorption, and drying.
Thermodynamic processes, including gas liquefaction, and refrigeration.
Mechanical processes, including solids transportation, crushing and pulverization, and screening and sieving.

Chemical engineering unit operations also fall in the following categories which involve elements from more than one class:

Combination (mixing)
Separation (distillation, crystallization)
Reaction (chemical reaction)

Furthermore, there are some unit operations which combine even these categories, such as reactive distillation and stirred tank reactors. A "pure" unit operation is a physical transport process, while a mixed chemical/physical process requires modeling both the physical transport, such as diffusion, and the chemical reaction. This is usually necessary for designing catalytic reactions, and is considered a separate discipline, termed chemical reaction engineering.

Chemical engineering unit operations and chemical engineering unit processing form the main principles of all kinds of chemical industries and are the foundation of designs of chemical plants, factories, and equipment used.

https://en.wikipedia.org/wiki/Unit_operation

In physics, transport phenomena are all irreversible processes of statistical nature stemming from the random continuous motion of molecules, mostly observed in fluids. Every aspect of transport phenomena is grounded in two primary concepts : the conservation laws, and the constitutive equations. The conservation laws, which in the context of transport phenomena are formulated as continuity equations, describe how the quantity being studied must be conserved. The constitutive equations describe how the quantity in question responds to various stimuli via transport. Prominent examples include Fourier's law of heat conduction and the Navier–Stokes equations, which describe, respectively, the response of heat flux to temperature gradients and the relationship between fluid flux and the forces applied to the fluid. These equations also demonstrate the deep connection between transport phenomena and thermodynamics, a connection that explains why transport phenomena are irreversible. Almost all of these physical phenomena ultimately involve systems seeking their lowest energy state in keeping with the principle of minimum energy. As they approach this state, they tend to achieve true thermodynamic equilibrium, at which point there are no longer any driving forces in the system and transport ceases. The various aspects of such equilibrium are directly connected to a specific transport: heat transfer is the system's attempt to achieve thermal equilibrium with its environment, just as mass and momentum transport move the system towards chemical and mechanical equilibrium.

Examples of transport processes include heat conduction (energy transfer), fluid flow (momentum transfer), molecular diffusion (mass transfer), radiation and electric charge transfer in semiconductors.^[3]^[4]^[5]^[6]

Transport phenomena have wide application. For example, in solid state physics, the motion and interaction of electrons, holes and phonons are studied under "transport phenomena". Another example is in biomedical engineering, where some transport phenomena of interest are thermoregulation, perfusion, and microfluidics. In chemical engineering, transport phenomena are studied in reactor design, analysis of molecular or diffusive transport mechanisms, and metallurgy.

The transport of mass, energy, and momentum can be affected by the presence of external sources:

An odor dissipates more slowly (and may intensify) when the source of the odor remains present.
The rate of cooling of a solid that is conducting heat depends on whether a heat source is applied.
The gravitational force acting on a rain drop counteracts the resistance or drag imparted by the surrounding air.

Diffusion[edit]

There are some notable similarities in equations for momentum, energy, and mass transfer^[7] which can all be transported by diffusion, as illustrated by the following examples:

Mass: the spreading and dissipation of odors in air is an example of mass diffusion.
Energy: the conduction of heat in a solid material is an example of heat diffusion.
Momentum: the drag experienced by a rain drop as it falls in the atmosphere is an example of momentum diffusion (the rain drop loses momentum to the surrounding air through viscous stresses and decelerates).

The molecular transfer equations of Newton's law for fluid momentum, Fourier's law for heat, and Fick's law for mass are very similar. One can convert from one transport coefficient to another in order to compare all three different transport phenomena.^[8]

Comparison of diffusion phenomena
Transported quantity	Physical phenomenon	Equation
Momentum	Viscosity (Newtonian fluid)	$\tau =-\nu {\frac {\partial \rho \upsilon }{\partial x}}$
Energy	Heat conduction (Fourier's law)	${\frac {q}{A}}=-k{\frac {dT}{dx}}$
Mass	Molecular diffusion (Fick's law)	$J=-D{\frac {\partial C}{\partial x}}$

(Definitions of these formulas are given below).

A great deal of effort has been devoted in the literature to developing analogies among these three transport processes for turbulent transfer so as to allow prediction of one from any of the others. The Reynolds analogy assumes that the turbulent diffusivities are all equal and that the molecular diffusivities of momentum (μ/ρ) and mass (D_AB) are negligible compared to the turbulent diffusivities. When liquids are present and/or drag is present, the analogy is not valid. Other analogies, such as von Karman's and Prandtl's, usually result in poor relations.

The most successful and most widely used analogy is the Chilton and Colburn J-factor analogy.^[9] This analogy is based on experimental data for gases and liquids in both the laminar and turbulent regimes. Although it is based on experimental data, it can be shown to satisfy the exact solution derived from laminar flow over a flat plate. All of this information is used to predict transfer of mass.

Onsager reciprocal relations[edit]

In fluid systems described in terms of temperature, matter density, and pressure, it is known that temperature differences lead to heat flows from the warmer to the colder parts of the system; similarly, pressure differences will lead to matter flow from high-pressure to low-pressure regions (a "reciprocal relation"). What is remarkable is the observation that, when both pressure and temperature vary, temperature differences at constant pressure can cause matter flow (as in convection) and pressure differences at constant temperature can cause heat flow. Perhaps surprisingly, the heat flow per unit of pressure difference and the density (matter) flow per unit of temperature difference are equal.

This equality was shown to be necessary by Lars Onsager using statistical mechanics as a consequence of the time reversibility of microscopic dynamics. The theory developed by Onsager is much more general than this example and capable of treating more than two thermodynamic forces at once.^[10]

Momentum transfer[edit]

In momentum transfer, the fluid is treated as a continuous distribution of matter. The study of momentum transfer, or fluid mechanics can be divided into two branches: fluid statics (fluids at rest), and fluid dynamics (fluids in motion). When a fluid is flowing in the x-direction parallel to a solid surface, the fluid has x-directed momentum, and its concentration is υ_xρ. By random diffusion of molecules there is an exchange of molecules in the z-direction. Hence the x-directed momentum has been transferred in the z-direction from the faster- to the slower-moving layer. The equation for momentum transfer is Newton's law of viscosity written as follows:

\tau _{{zx}}=-\nu {\frac {\partial \rho \upsilon _{x}}{\partial z}}

where τ_zx is the flux of x-directed momentum in the z-direction, ν is μ/ρ, the momentum diffusivity, z is the distance of transport or diffusion, ρ is the density, and μ is the dynamic viscosity. Newton's law of viscosity is the simplest relationship between the flux of momentum and the velocity gradient.

Mass transfer[edit]

When a system contains two or more components whose concentration vary from point to point, there is a natural tendency for mass to be transferred, minimizing any concentration difference within the system. Mass Transfer in a system is governed by Fick's First Law: 'Diffusion flux from higher concentration to lower concentration is proportional to the gradient of the concentration of the substance and the diffusivity of the substance in the medium.' Mass transfer can take place due to different driving forces. Some of them are:^[11]

Mass can be transferred by the action of a pressure gradient (pressure diffusion)
Forced diffusion occurs because of the action of some external force
Diffusion can be caused by temperature gradients (thermal diffusion)
Diffusion can be caused by differences in chemical potential

This can be compared to Fick's Law of Diffusion, for a species A in a binary mixture consisting of A and B:

J_{{Ay}}=-D_{{AB}}{\frac {\partial Ca}{\partial y}}

where D is the diffusivity constant.

Energy transfer[edit]

All processes in engineering involve the transfer of energy. Some examples are the heating and cooling of process streams, phase changes, distillations, etc. The basic principle is the first law of thermodynamics which is expressed as follows for a static system:

q=-k{\frac {dT}{dx}}

The net flux of energy through a system equals the conductivity times the rate of change of temperature with respect to position.

For other systems that involve either turbulent flow, complex geometries or difficult boundary conditions another equation would be easier to use:

Q=h\cdot A\cdot {\Delta T}

where A is the surface area, : ${\Delta T}$ is the temperature driving force, Q is the heat flow per unit time, and h is the heat transfer coefficient.

Within heat transfer, two types of convection can occur:

Forced convection can occur in both laminar and turbulent flow. In the situation of laminar flow in circular tubes, several dimensionless numbers are used such as Nusselt number, Reynolds number, and Prandtl number. The commonly used equation is $Nu_{{a}}={\frac {h_{{a}}D}{k}}$ .

Natural or free convection is a function of Grashof and Prandtl numbers. The complexities of free convection heat transfer make it necessary to mainly use empirical relations from experimental data.^[11]

Heat transfer is analyzed in packed beds, nuclear reactors and heat exchangers.

Applications[edit]

Pollution[edit]

The study of transport processes is relevant for understanding the release and distribution of pollutants into the environment. In particular, accurate modeling can inform mitigation strategies. Examples include the control of surface water pollution from urban runoff, and policies intended to reduce the copper content of vehicle brake pads in the U.S.^[12]^[13]

Nikiya Anton Bettey 2021

Blog Archive

Saturday, September 18, 2021

09-18-2021-0414 - Psuedoforce Notes Drafting

Contents

Background[edit]

Mechanism[edit]

Cross section[edit]

Lawson criterion[edit]

Triple product: density, temperature, time[edit]

Energy capture[edit]

Methods[edit]

Plasma behavior[edit]

Magnetic confinement[edit]

Inertial confinement[edit]

Magnetic or electric pinches[edit]

Inertial electrostatic confinement[edit]

Other[edit]

Common tools[edit]

Heating[edit]

Measurement[edit]

Power production[edit]

Confinement[edit]

Unconfined[edit]

Magnetic confinement[edit]

Magnetic Mirror[edit]

Magnetic Loops[edit]

Inertial confinement[edit]

Electrostatic confinement[edit]

Chemical Engineering[edit]

Diffusion[edit]

Onsager reciprocal relations[edit]

Momentum transfer[edit]

Mass transfer[edit]

Energy transfer[edit]

Applications[edit]

Pollution[edit]

See also[edit]

No comments:

Post a Comment