The pressure referred to is the partial pressure of the substance, not the total (e.g. atmospheric) pressure of the entire system. So, all solids that possess an appreciable vapour pressure at a certain temperature usually can sublime in air (e.g. water ice just below 0 °C).
The term sublimation refers to a physical change of state (imp. indep chemical/element or standard mixture non reactive ; nested systems in testing) and is not used to describe the transformation of a solid to a gas in a chemical reaction.
https://en.wikipedia.org/wiki/Sublimation_(phase_transition)
In a mixture of gases, each constituent gas has a partial pressure which is the notional pressure of that constituent gas if it alone occupied the entire volume of the original mixture at the same temperature.[1] The total pressure of an ideal gas mixture is the sum of the partial pressures of the gases in the mixture (Dalton's Law).
The partial pressure of a gas is a measure of thermodynamic activity of the gas's molecules.
Gases dissolve, diffuse, and react according to their partial pressures, and not according to their concentrations in gas mixtures or liquids.
https://en.wikipedia.org/wiki/Partial_pressure
Pressure (symbol: p or P) is the force applied perpendicular to the surface of an object per unit area over which that force is distributed.: 445 [1] Gauge pressure(also spelled gage pressure)[a] is the pressure relative to the ambient pressure.
Various units are used to express pressure. Some of these derive from a unit of force divided by a unit of area; the SI unit of pressure, the pascal (Pa), for example, is one newton per square metre (N/m2); similarly, the pound-force per square inch (psi) is the traditional unit of pressure in the imperial and U.S. customary systems. Pressure may also be expressed in terms of standard atmospheric pressure; the atmosphere (atm) is equal to this pressure, and the torr is defined as 1⁄760 of this. Manometric units such as the centimetre of water, millimetre of mercury, and inch of mercury are used to express pressures in terms of the height of column of a particular fluid in a manometer.
Mathematically:
where:
- is the pressure,
- is the magnitude of the normal force,
- is the area of the surface on contact.
Pressure is a scalar quantity. It relates the vector area element (a vector normal to the surface) with the normal force acting on it. The pressure is the scalar proportionality constant that relates the two normal vectors:
The minus sign comes from the fact that the force is considered towards the surface element, while the normal vector points outward. The equation has meaning in that, for any surface S in contact with the fluid, the total force exerted by the fluid on that surface is the surface integral over S of the right-hand side of the above equation.
It is incorrect (although rather usual) to say "the pressure is directed in such or such direction". The pressure, as a scalar, has no direction. The force given by the previous relationship to the quantity has a direction, but the pressure does not. If we change the orientation of the surface element, the direction of the normal force changes accordingly, but the pressure remains the same.
Pressure is distributed to solid boundaries or across arbitrary sections of fluid normal to these boundaries or sections at every point. It is a fundamental parameter in thermodynamics, and it is conjugate to volume.
https://en.wikipedia.org/wiki/Pressure
https://en.wikipedia.org/wiki/Proportionality_(mathematics)#Direct_proportionality
Pressure measurement is the analysis of an applied force by a fluid (liquid or gas) on a surface. Pressure is typically measured in units of force per unit of surface area. Many techniques have been developed for the measurement of pressure and vacuum. Instruments used to measure and display pressure in an integral unit are called pressure meters or pressure gauges or vacuum gauges. A manometer is a good example, as it uses the surface area and weight of a column of liquid to both measure and indicate pressure. Likewise the widely used Bourdon gauge is a mechanical device, which both measures and indicates and is probably the best known type of gauge.
A vacuum gauge is a pressure gauge used to measure pressures lower than the ambient atmospheric pressure, which is set as the zero point, in negative values (e.g.: −15 psig or −760 mmHg equals total vacuum). Most gauges measure pressure relative to atmospheric pressure as the zero point, so this form of reading is simply referred to as "gauge pressure". However, anything greater than total vacuum is technically a form of pressure. For very accurate readings, especially at very low pressures, a gauge that uses total vacuum as the zero point may be used, giving pressure readings in an absolute scale.
Other methods of pressure measurement involve sensors that can transmit the pressure reading to a remote indicator or control system (telemetry).
Absolute, gauge and differential pressures — zero reference[edit]
Everyday pressure measurements, such as for vehicle tire pressure, are usually made relative to ambient air pressure. In other cases measurements are made relative to a vacuum or to some other specific reference. When distinguishing between these zero references, the following terms are used:
Absolute pressure is zero-referenced against a perfect vacuum, using an absolute scale, so it is equal to gauge pressure plus atmospheric pressure.
Gauge pressure is zero-referenced against ambient air pressure, so it is equal to absolute pressure minus atmospheric pressure. Negative signs are usually omitted.[citation needed] To distinguish a negative pressure, the value may be appended with the word "vacuum" or the gauge may be labeled a "vacuum gauge". These are further divided into two subcategories: high and low vacuum (and sometimes ultra-high vacuum). The applicable pressure ranges of many of the techniques used to measure vacuums overlap. Hence, by combining several different types of gauge, it is possible to measure system pressure continuously from 10 mbar down to 10−11 mbar.
Differential pressure is the difference in pressure between two points.
The zero reference in use is usually implied by context, and these words are added only when clarification is needed. Tire pressure and blood pressure are gauge pressures by convention, while atmospheric pressures, deep vacuum pressures, and altimeter pressures must be absolute.
For most working fluids where a fluid exists in a closed system, gauge pressure measurement prevails. Pressure instruments connected to the system will indicate pressures relative to the current atmospheric pressure. The situation changes when extreme vacuum pressures are measured, then absolute pressures are typically used instead.
Differential pressures are commonly used in industrial process systems. Differential pressure gauges have two inlet ports, each connected to one of the volumes whose pressure is to be monitored. In effect, such a gauge performs the mathematical operation of subtraction through mechanical means, obviating the need for an operator or control system to watch two separate gauges and determine the difference in readings.
Moderate vacuum pressure readings can be ambiguous without the proper context, as they may represent absolute pressure or gauge pressure without a negative sign. Thus a vacuum of 26 inHg gauge is equivalent to an absolute pressure of 4 inHg, calculated as 30 inHg (typical atmospheric pressure) − 26 inHg (gauge pressure).
Atmospheric pressure is typically about 100 kPa at sea level, but is variable with altitude and weather. If the absolute pressure of a fluid stays constant, the gauge pressure of the same fluid will vary as atmospheric pressure changes. For example, when a car drives up a mountain, the (gauge) tire pressure goes up because atmospheric pressure goes down. The absolute pressure in the tire is essentially unchanged.
Using atmospheric pressure as reference is usually signified by a "g" for gauge after the pressure unit, e.g. 70 psig, which means that the pressure measured is the total pressure minus atmospheric pressure. There are two types of gauge reference pressure: vented gauge (vg) and sealed gauge (sg).
A vented-gauge pressure transmitter, for example, allows the outside air pressure to be exposed to the negative side of the pressure-sensing diaphragm, through a vented cable or a hole on the side of the device, so that it always measures the pressure referred to ambient barometric pressure. Thus a vented-gauge reference pressure sensor should always read zero pressure when the process pressure connection is held open to the air.
A sealed gauge reference is very similar, except that atmospheric pressure is sealed on the negative side of the diaphragm. This is usually adopted on high pressure ranges, such as hydraulics, where atmospheric pressure changes will have a negligible effect on the accuracy of the reading, so venting is not necessary. This also allows some manufacturers to provide secondary pressure containment as an extra precaution for pressure equipment safety if the burst pressure of the primary pressure sensing diaphragm is exceeded.
There is another way of creating a sealed gauge reference, and this is to seal a high vacuum on the reverse side of the sensing diaphragm. Then the output signal is offset, so the pressure sensor reads close to zero when measuring atmospheric pressure.
A sealed gauge reference pressure transducer will never read exactly zero because atmospheric pressure is always changing and the reference in this case is fixed at 1 bar.
To produce an absolute pressure sensor, the manufacturer seals a high vacuum behind the sensing diaphragm. If the process-pressure connection of an absolute-pressure transmitter is open to the air, it will read the actual barometric pressure.
https://en.wikipedia.org/wiki/Pressure_measurement#Gauge
Atmospheric pressure, also known as barometric pressure (after the barometer), is the pressure within the atmosphere of Earth. The standard atmosphere (symbol: atm) is a unit of pressure defined as 101,325 Pa (1,013.25 hPa; 1,013.25 mbar), which is equivalent to 760 mm Hg, 29.9212 inches Hg, or 14.696 psi.[1] The atm unit is roughly equivalent to the mean sea-level atmospheric pressure on Earth; that is, the Earth's atmospheric pressure at sea level is approximately 1 atm.
In most circumstances, atmospheric pressure is closely approximated by the hydrostatic pressure caused by the weight of air above the measurement point. As elevation increases, there is less overlying atmospheric mass, so that atmospheric pressure decreases with increasing elevation. Because the atmosphere is thin relative to the Earth's radius—especially the dense atmospheric layer at low altitudes—the Earth's gravitational acceleration as a function of altitude can be approximated as constant and contributes little to this fall off. Pressure measures force per unit area, with SI units of pascals (1 pascal = 1 newton per square metre, 1 N/m2). On average, a column of air with a cross-sectional area of 1 square centimetre (cm2), measured from mean (average) sea level to the top of Earth's atmosphere, has a mass of about 1.03 kilogram and exerts a force or "weight" of about 10.1 newtons, resulting in a pressure of 10.1 N/cm2 or 101 kN/m2 (101 kilopascals, kPa). A column of air with a cross-sectional area of 1 in2 would have a weight of about 14.7 lbf, resulting in a pressure of 14.7 lbf/in2.
https://en.wikipedia.org/wiki/Atmospheric_pressure
Standard temperature and pressure (STP) are standard sets of conditions for experimental measurements to be established to allow comparisons to be made between different sets of data. The most used standards are those of the International Union of Pure and Applied Chemistry (IUPAC) and the National Institute of Standards and Technology (NIST), although these are not universally accepted standards. Other organizations have established a variety of alternative definitions for their standard reference conditions.
In chemistry, IUPAC changed the definition of standard temperature and pressure in 1982:[1][2]
- Until 1982, STP was defined as a temperature of 273.15 K (0 °C, 32 °F) and an absolute pressure of exactly 1 atm (101.325 kPa).
- Since 1982, STP is defined as a temperature of 273.15 K (0 °C, 32 °F) and an absolute pressure of exactly 105 Pa (100 kPa, 1 bar).
STP should not be confused with the standard state commonly used in thermodynamic evaluations of the Gibbs energy of a reaction.
NIST uses a temperature of 20 °C (293.15 K, 68 °F) and an absolute pressure of 1 atm (14.696 psi, 101.325 kPa). This standard is also called normal temperature and pressure (abbreviated as NTP). These stated values of STP used by NIST have not been verified and require a source. However, values cited in Modern Thermodynamics with Statistical Mechanics by Carl S. Helrich and A Guide to the NIST Chemistry WebBook by Peter J.[vague] Linstrom suggest a common STP in use by NIST for thermodynamic experiments is 298.15 K (25°C, 77°F) and 1 bar (14.5038 psi, 100 kPa).[3][4]
The International Standard Metric Conditions for natural gas and similar fluids are 288.15 K (15.00 °C; 59.00 °F) and 101.325 kPa.[5]
In industry and commerce, standard conditions for temperature and pressure are often necessary to define the standard reference conditions to express the volumes of gases and liquids and related quantities such as the rate of volumetric flow (the volumes of gases vary significantly with temperature and pressure): standard cubic meters per second (Sm3/s), and normal cubic meters per second (Nm3/s).
However, many technical publications (books, journals, advertisements for equipment and machinery) simply state "standard conditions" without specifying them; often substituting the term with older "normal conditions", or "NC". In special cases this can lead to confusion and errors. Good practice always incorporates the reference conditions of temperature and pressure. If not stated, some room environment conditions are supposed, close to 1 atm pressure, 293 K (20 °C), and 0% humidity.
https://en.wikipedia.org/wiki/Standard_conditions_for_temperature_and_pressure
In chemistry, the standard state of a material (pure substance, mixture or solution) is a reference point used to calculate its properties under different conditions. A superscript circle is used to designate a thermodynamic quantity in the standard state, such as change in enthalpy (ΔH°), change in entropy (ΔS°), or change in Gibbs free energy (ΔG°).[1][2] (See discussion about typesetting below.)
In principle, the choice of standard state is arbitrary, although the International Union of Pure and Applied Chemistry (IUPAC) recommends a conventional set of standard states for general use.[3] IUPAC recommends using a standard pressure p⦵ = 105 Pa.[4] Strictly speaking, temperature is not part of the definition of a standard state. For example, as discussed below, the standard state of a gas is conventionally chosen to be unit pressure (usually in bar) ideal gas, regardless of the temperature. However, most tables of thermodynamic quantities are compiled at specific temperatures, most commonly 298.15 K (25.00 °C; 77.00 °F) or, somewhat less commonly, 273.15 K (0.00 °C; 32.00 °F).[5]
The standard state should not be confused with standard temperature and pressure (STP) for gases,[6] nor with the standard solutions used in analytical chemistry.[7] STP is commonly used for calculations involving gases that approximate an ideal gas, whereas standard state conditions are used for thermodynamic calculations.[5]
For a given material or substance, the standard state is the reference state for the material's thermodynamic state properties such as enthalpy, entropy, Gibbs free energy, and for many other material standards. The standard enthalpy change of formation for an element in its standard state is zero, and this convention allows a wide range of other thermodynamic quantities to be calculated and tabulated. The standard state of a substance does not have to exist in nature: for example, it is possible to calculate values for steam at 298.15 K and 105 Pa, although steam does not exist (as a gas) under these conditions. The advantage of this practice is that tables of thermodynamic properties prepared in this way are self-consistent.
Conventional standard states[edit]
Many standard states are non-physical states, often referred to as "hypothetical states". Nevertheless, their thermodynamic properties are well-defined, usually by an extrapolation from some limiting condition, such as zero pressure or zero concentration, to a specified condition (usually unit concentration or pressure) using an ideal extrapolating function, such as ideal solution or ideal gas behavior, or by empirical measurements.
Gases[edit]
The standard state for a gas is the hypothetical state it would have as a pure substance obeying the ideal gas equation at standard pressure (105 Pa, or 1 bar). No real gas has perfectly ideal behavior, but this definition of the standard state allows corrections for non-ideality to be made consistently for all the different gases.
Liquids and solids[edit]
The standard state for liquids and solids is simply the state of the pure substance subjected to a total pressure of 105 Pa. For most elements, the reference point of ΔHf⦵ = 0 is defined for the most stable allotrope of the element, such as graphite in the case of carbon, and the β-phase (white tin) in the case of tin. An exception is white phosphorus, the most common allotrope of phosphorus, which is defined as the standard state despite the fact that it is only metastable.[8]
Solutes[edit]
For a substance in solution (solute), the standard state is usually chosen as the hypothetical state it would have at the standard state molality or amount concentration but exhibiting infinite-dilution behavior (where there are no solute-solute interactions, but solute-solvent interactions are present). The reason for this unusual definition is that the behavior of a solute at the limit of infinite dilution is described by equations which are very similar to the equations for ideal gases. Hence taking infinite-dilution behavior to be the standard state allows corrections for non-ideality to be made consistently for all the different solutes. The standard state molality is 1 mol kg−1, while the standard state molarity is 1 mol dm−3.
Other choices are possible. For example, the use of a standard state concentration of 
Adsorbates[edit]
For molecules adsorbed on surfaces there have been various conventions proposed based on hypothetical standard states. For adsorption that occurs on specific sites (Langmuir adsorption) the most common standard state is a relative coverage of θ°=0.5, as this choice results in a cancellation of the configurational entropy term and is also consistent with neglecting to include the standard state (which is a common error).[12] The advantage of using θ°=0.5 is that the configurational term cancels and the entropy extracted from thermodynamic analyses is thus reflective of intra-molecular changes between the bulk phase (such as gas or liquid) and the adsorbed state. There may be benefit to tabulating values based on both a relative coverage based standard state and in additional column an absolute coverage based standard state. For 2D gas states, the complication of discrete states does not arise and an absolute density base standard state has been proposed, similar for the 3D gas phase.[12]
Typesetting[edit]
At the time of development in the nineteenth century, the superscript Plimsoll symbol (⦵) was adopted to indicate the non-zero nature of the standard state.[13] IUPAC recommends in the 3rd edition of Quantities, Units and Symbols in Physical Chemistry a symbol which seems to be a degree sign (°) as a substitute for the plimsoll mark. In the very same publication the plimsoll mark appears to be constructed by combining a horizontal stroke with a degree sign.[14] A range of similar symbols are used in the literature: a stroked lowercase letter O (o),[15] a superscript zero (0)[16] or a circle with a horizontal bar either where the bar extends beyond the boundaries of the circle (U+29B5 ⦵ CIRCLE WITH HORIZONTAL BAR) or is enclosed by the circle, dividing the circle in half (U+2296 ⊖ CIRCLED MINUS).[17][18] When compared to the plimsoll symbol used on vessels, the horizontal bar should extend beyond the boundaries of the circle; care should be taken not to confuse the symbol with the Greek letter theta (uppercase Θ or ϴ, lowercase θ ).
The use of a degree symbol (°) or superscript zero (
See also[edit]
Standard conditions for temperature and pressure
Standard molar entropy
References[edit]
International Union of Pure and Applied Chemistry (1982). "Notation for states and processes, significance of the word standard in chemical thermodynamics, and remarks on commonly tabulated forms of thermodynamic functions" (PDF). Pure Appl. Chem. 54 (6): 1239–50. doi:10.1351/pac198254061239.
IUPAC–IUB–IUPAB Interunion Commission of Biothermodynamics (1976). "Recommendations for measurement and presentation of biochemical equilibrium data" (PDF). J. Biol. Chem. 251 (22): 6879–85.
In chemistry, the standard molar entropy is the entropy content of one mole of pure substance at a standard state of pressure and any temperature of interest. These are often (but not necessarily) chosen to be the standard temperature and pressure.
The standard molar entropy at pressure = is usually given the symbol S°, and has units of joules per mole kelvin (J⋅mol−1⋅K−1). Unlike standard enthalpies of formation, the value of S° is absolute. That is, an element in its standard state has a definite, nonzero value of S at room temperature. The entropy of a pure crystalline structure can be 0 J⋅mol−1⋅K−1 only at 0 K, according to the third law of thermodynamics. However, this assumes that the material forms a 'perfect crystal' without any residual entropy. This can be due to crystallographic defects, dislocations, and/or incomplete rotational quenching within the solid, as originally pointed out by Linus Pauling.[1]) These contributions to the entropy are always present, because crystals always grow at a finite rate and at temperature. However, the residual entropy is often quite negligible and can be accounted for when it occurs using statistical mechanics.
Thermodynamics[edit]
If a mole of a solid substance is a perfectly ordered solid at 0 K, then if the solid warmed by its surroundings to 298.15 K without melting, its absolute molar entropy would be the sum of a series of N stepwise and reversible entropy changes. The limit of this sum as becomes an integral:
In this example, and is the molar heat capacity at a constant pressure of the substance in the reversible process k. The molar heat capacity is not constant during the experiment because it changes depending on the (increasing) temperature of the substance. Therefore, a table of values for is required to find the total molar entropy. The quantity represents the ratio of a very small exchange of heat energy to the temperature T. The total molar entropy is the sum of many small changes in molar entropy, where each small change can be considered a reversible process.
Chemistry[edit]
The standard molar entropy of a gas at STP includes contributions from:[2]
- The heat capacity of one mole of the solid from 0 K to the melting point (including heat absorbed in any changes between different crystal structures).
- The latent heat of fusion of the solid.
- The heat capacity of the liquid from the melting point to the boiling point.
- The latent heat of vaporization of the liquid.
- The heat capacity of the gas from the boiling point to room temperature.
Changes in entropy are associated with phase transitions and chemical reactions. Chemical equations make use of the standard molar entropy of reactants and products to find the standard entropy of reaction:[3]
The standard entropy of reaction helps determine whether the reaction will take place spontaneously. According to the second law of thermodynamics, a spontaneous reaction always results in an increase in total entropy of the system and its surroundings:
Molar entropy is not same for all gases. Under identical conditions, it is greater for a heavier gas.
See also[edit]
Entropy is a scientific concept, as well as a measurable physical property that is most commonly associated with a state of disorder, randomness, or uncertainty. The term and the concept are used in diverse fields, from classical thermodynamics, where it was first recognized, to the microscopic description of nature in statistical physics, and to the principles of information theory. It has found far-ranging applications in chemistry and physics, in biological systems and their relation to life, in cosmology, economics, sociology, weather science, climate change, and information systems including the transmission of information in telecommunication.[1]
The thermodynamic concept was referred to by Scottish scientist and engineer Macquorn Rankine in 1850 with the names thermodynamic function and heat-potential.[2] In 1865, German physicist Rudolph Clausius, one of the leading founders of the field of thermodynamics, defined it as the quotient of an infinitesimal amount of heat to the instantaneous temperature. He initially described it as transformation-content, in German Verwandlungsinhalt, and later coined the term entropy from a Greek word for transformation. Referring to microscopic constitution and structure, in 1862, Clausius interpreted the concept as meaning disgregation.[3]
A consequence of entropy is that certain processes are irreversible or impossible, aside from the requirement of not violating the conservation of energy, the latter being expressed in the first law of thermodynamics. Entropy is central to the second law of thermodynamics, which states that the entropy of isolated systems left to spontaneous evolution cannot decrease with time, as they always arrive at a state of thermodynamic equilibrium, where the entropy is highest.
Austrian physicist Ludwig Boltzmann explained entropy as the measure of the number of possible microscopic arrangements or states of individual atoms and molecules of a system that comply with the macroscopic condition of the system. He thereby introduced the concept of statistical disorder and probability distributions into a new field of thermodynamics, called statistical mechanics, and found the link between the microscopic interactions, which fluctuate about an average configuration, to the macroscopically observable behavior, in form of a simple logarithmic law, with a proportionality constant, the Boltzmann constant, that has become one of the defining universal constants for the modern International System of Units (SI).
In 1948, Bell Labs scientist Claude Shannon developed similar statistical concepts of measuring microscopic uncertainty and multiplicity to the problem of random losses of information in telecommunication signals. Upon John von Neumann's suggestion, Shannon named this entity of missing information in analogous manner to its use in statistical mechanics as entropy, and gave birth to the field of information theory. This description has been proposed as a universal definition of the concept of entropy.[4]
https://en.wikipedia.org/wiki/Entropy
Thermodynamics is a branch of physics that deals with heat, work, and temperature, and their relation to energy, radiation, and physical properties of matter. The behavior of these quantities is governed by the four laws of thermodynamics which convey a quantitative description using measurable macroscopic physical quantities, but may be explained in terms of microscopic constituents by statistical mechanics. Thermodynamics applies to a wide variety of topics in science and engineering, especially physical chemistry, biochemistry, chemical engineering and mechanical engineering, but also in other complex fields such as meteorology.
Historically, thermodynamics developed out of a desire to increase the efficiency of early steam engines, particularly through the work of French physicist Nicolas Léonard Sadi Carnot (1824) who believed that engine efficiency was the key that could help France win the Napoleonic Wars.[1] Scots-Irish physicist Lord Kelvin was the first to formulate a concise definition of thermodynamics in 1854[2] which stated, "Thermo-dynamics is the subject of the relation of heat to forces acting between contiguous parts of bodies, and the relation of heat to electrical agency."
The initial application of thermodynamics to mechanical heat engines was quickly extended to the study of chemical compounds and chemical reactions. Chemical thermodynamics studies the nature of the role of entropy in the process of chemical reactions and has provided the bulk of expansion and knowledge of the field.[3][4][5][6][7][8][9][10][11] Other formulations of thermodynamics emerged. Statistical thermodynamics, or statistical mechanics, concerns itself with statistical predictions of the collective motion of particles from their microscopic behavior. In 1909, Constantin Carathéodory presented a purely mathematical approach in an axiomatic formulation, a description often referred to as geometrical thermodynamics.
Introduction[edit]
A description of any thermodynamic system employs the four laws of thermodynamics that form an axiomatic basis. The first law specifies that energy can be transferred between physical systems as heat, as work, and with transfer of matter.[12] The second law defines the existence of a quantity called entropy, that describes the direction, thermodynamically, that a system can evolve and quantifies the state of order of a system and that can be used to quantify the useful work that can be extracted from the system.[13]
In thermodynamics, interactions between large ensembles of objects are studied and categorized. Central to this are the concepts of the thermodynamic system and its surroundings. A system is composed of particles, whose average motions define its properties, and those properties are in turn related to one another through equations of state. Properties can be combined to express internal energy and thermodynamic potentials, which are useful for determining conditions for equilibrium and spontaneous processes.
With these tools, thermodynamics can be used to describe how systems respond to changes in their environment. This can be applied to a wide variety of topics in science and engineering, such as engines, phase transitions, chemical reactions, transport phenomena, and even black holes. The results of thermodynamics are essential for other fields of physics and for chemistry, chemical engineering, corrosion engineering, aerospace engineering, mechanical engineering, cell biology, biomedical engineering, materials science, and economics, to name a few.[14][15]
This article is focused mainly on classical thermodynamics which primarily studies systems in thermodynamic equilibrium. Non-equilibrium thermodynamics is often treated as an extension of the classical treatment, but statistical mechanics has brought many advances to that field.
The history of thermodynamics as a scientific discipline generally begins with Otto von Guericke who, in 1650, built and designed the world's first vacuum pump and demonstrated a vacuum using his Magdeburg hemispheres. Guericke was driven to make a vacuum in order to disprove Aristotle's long-held supposition that 'nature abhors a vacuum'. Shortly after Guericke, the Anglo-Irish physicist and chemist Robert Boyle had learned of Guericke's designs and, in 1656, in coordination with English scientist Robert Hooke, built an air pump.[17] Using this pump, Boyle and Hooke noticed a correlation between pressure, temperature, and volume. In time, Boyle's Law was formulated, which states that pressure and volume are inversely proportional. Then, in 1679, based on these concepts, an associate of Boyle's named Denis Papin built a steam digester, which was a closed vessel with a tightly fitting lid that confined steam until a high pressure was generated.
Later designs implemented a steam release valve that kept the machine from exploding. By watching the valve rhythmically move up and down, Papin conceived of the idea of a piston and a cylinder engine. He did not, however, follow through with his design. Nevertheless, in 1697, based on Papin's designs, engineer Thomas Savery built the first engine, followed by Thomas Newcomen in 1712. Although these early engines were crude and inefficient, they attracted the attention of the leading scientists of the time.
https://en.wikipedia.org/wiki/Thermodynamics
The laws of thermodynamics define a group of physical quantities, such as temperature, energy, and entropy, that characterize thermodynamic systems in thermodynamic equilibrium. The laws also use various parameters for thermodynamic processes, such as thermodynamic work and heat, and establish relationships between them. They state empirical facts that form a basis of precluding the possibility of certain phenomena, such as perpetual motion. In addition to their use in thermodynamics, they are important fundamental laws of physics in general, and are applicable in other natural sciences.
Traditionally, thermodynamics has recognized three fundamental laws, simply named by an ordinal identification, the first law, the second law, and the third law.[1][2][3] A more fundamental statement was later labelled as the zeroth law, after the first three laws had been established.
The zeroth law of thermodynamics defines thermal equilibrium and forms a basis for the definition of temperature: If two systems are each in thermal equilibrium with a third system, then they are in thermal equilibrium with each other.
The first law of thermodynamics states that, when energy passes into or out of a system (as work, heat, or matter), the system's internal energy changes in accord with the law of conservation of energy.
The second law of thermodynamics states that in a natural thermodynamic process, the sum of the entropies of the interacting thermodynamic systems never decreases. Another form of the statement is that heat does not spontaneously pass from a colder body to a warmer body.
The third law of thermodynamics states that a system's entropy approaches a constant value as the temperature approaches absolute zero. With the exception of non-crystalline solids (glasses) the entropy of a system at absolute zero is typically close to zero.[2]
The first and second law prohibit two kinds of perpetual motion machines, respectively: the perpetual motion machine of the first kind which produces work with no energy input, and the perpetual motion machine of the second kind which spontaneously converts thermal energy into mechanical work.
https://en.wikipedia.org/wiki/Laws_of_thermodynamics
In thermodynamics, the internal energy of a system is expressed in terms of pairs of conjugate variables such as temperature and entropy or pressure and volume. In fact, all thermodynamic potentials are expressed in terms of conjugate pairs. The product of two quantities that are conjugate has units of energy or sometimes power.
For a mechanical system, a small increment of energy is the product of a force times a small displacement. A similar situation exists in thermodynamics. An increment in the energy of a thermodynamic system can be expressed as the sum of the products of certain generalized "forces" that, when unbalanced, cause certain generalized "displacements", and the product of the two is the energy transferred as a result. These forces and their associated displacements are called conjugate variables. The thermodynamic force is always an intensive variable and the displacement is always an extensive variable, yielding an extensive energy transfer. The intensive (force) variable is the derivative of the internal energy with respect to the extensive (displacement) variable, while all other extensive variables are held constant.
The thermodynamic square can be used as a tool to recall and derive some of the thermodynamic potentials based on conjugate variables.
In the above description, the product of two conjugate variables yields an energy. In other words, the conjugate pairs are conjugate with respect to energy. In general, conjugate pairs can be defined with respect to any thermodynamic state function. Conjugate pairs with respect to entropy are often used, in which the product of the conjugate pairs yields an entropy. Such conjugate pairs are particularly useful in the analysis of irreversible processes, as exemplified in the derivation of the Onsager reciprocal relations.
https://en.wikipedia.org/wiki/Conjugate_variables_(thermodynamics)
The thermodynamic square (also known as the thermodynamic wheel, Guggenheim scheme or Born square) is a mnemonic diagram attributed to Max Born and used to help determine thermodynamic relations. Born presented the thermodynamic square in a 1929 lecture.[1] The symmetry of thermodynamics appears in a paper by F.O. Koenig.[2] The corners represent common conjugate variables while the sides represent thermodynamic potentials. The placement and relation among the variables serves as a key to recall the relations they constitute.
A mnemonic used by students to remember the Maxwell relations (in thermodynamics) is "Good Physicists Have Studied Under Very Fine Teachers", which helps them remember the order of the variables in the square, in clockwise direction. Another mnemonic used here is "Valid Facts and Theoretical Understanding Generate Solutions to Hard Problems", which gives the letter in the normal left-to-right writing direction. Both times A has to be identified with F, another common symbol for Helmholtz' Free Energy. To prevent the need for this switch the following mnemonic is also widely used:"Good Physicists Have Studied Under Very Ambitious Teachers"; another one is Good Physicists Have SUVAT, in reference to the equations of motion. One other useful variation of the mnemonic when the symbol E is used for internal energy instead of U is the following: "Some Hard Problems Go To Finish Very Easy".[3]
https://en.wikipedia.org/wiki/Thermodynamic_square
The atmosphere of Earth, commonly known as air, is the layer of gases retained by Earth's gravity that surrounds the planet and forms its planetary atmosphere. The atmosphere of Earth protects life on Earth by creating pressure allowing for liquid water to exist on the Earth's surface, absorbing ultraviolet solar radiation, warming the surface through heat retention (greenhouse effect), and reducing temperature extremes between day and night (the diurnal temperature variation).
By mole fraction (i.e., by number of molecules), dry air contains 78.08% nitrogen, 20.95% oxygen, 0.93% argon, 0.04% carbon dioxide, and small amounts of other gases.[8] Air also contains a variable amount of water vapor, on average around 1% at sea level, and 0.4% over the entire atmosphere. Air composition, temperature, and atmospheric pressure vary with altitude. Within the atmosphere, air suitable for use in photosynthesis by terrestrial plants and breathing of terrestrial animals is found only in Earth's troposphere.[citation needed]
Earth's early atmosphere consisted of gases in the solar nebula, primarily hydrogen. The atmosphere changed significantly over time, affected by many factors such as volcanism, life, and weathering. Recently, human activity has also contributed to atmospheric changes, such as global warming, ozone depletion and acid deposition.
The atmosphere has a mass of about 5.15×1018 kg,[9] three quarters of which is within about 11 km (6.8 mi; 36,000 ft) of the surface. The atmosphere becomes thinner with increasing altitude, with no definite boundary between the atmosphere and outer space. The Kármán line, at 100 km (62 mi) or 1.57% of Earth's radius, is often used as the border between the atmosphere and outer space. Atmospheric effects become noticeable during atmospheric reentry of spacecraft at an altitude of around 120 km (75 mi). Several layers can be distinguished in the atmosphere, based on characteristics such as temperature and composition.
The study of Earth's atmosphere and its processes is called atmospheric science (aerology), and includes multiple subfields, such as climatology and atmospheric physics. Early pioneers in the field include Léon Teisserenc de Bort and Richard Assmann.[10] The study of historic atmosphere is called paleoclimatology.
https://en.wikipedia.org/wiki/Atmosphere_of_Earth
In astrophysics, accretion is the accumulation of particles into a massive object by gravitationally attracting more matter, typically gaseous matter, in an accretion disk.[1][2] Most astronomical objects, such as galaxies, stars, and planets, are formed by accretion processes.
https://en.wikipedia.org/wiki/Accretion_(astrophysics)
Nuclear transmutation is the conversion of one chemical element or an isotope into another chemical element.[1] Nuclear transmutation occurs in any process where the number of protons or neutrons in the nucleus of an atom is changed.
A transmutation can be achieved either by nuclear reactions (in which an outside particle reacts with a nucleus) or by radioactive decay, where no outside cause is needed.
Natural transmutation by stellar nucleosynthesis in the past created most of the heavier chemical elements in the known existing universe, and continues to take place to this day, creating the vast majority of the most common elements in the universe, including helium, oxygen and carbon. Most stars carry out transmutation through fusion reactions involving hydrogen and helium, while much larger stars are also capable of fusing heavier elements up to iron late in their evolution.
Elements heavier than iron, such as gold or lead, are created through elemental transmutations that can only naturally occur in supernovae. As stars begin to fuse heavier elements, substantially less energy is released from each fusion reaction. This continues until it reaches iron which is produced by an endothermic reaction consuming energy. No heavier element can be produced in such conditions.
One type of natural transmutation observable in the present occurs when certain radioactive elements present in nature spontaneously decay by a process that causes transmutation, such as alpha or beta decay. An example is the natural decay of potassium-40 to argon-40, which forms most of the argon in the air. Also on Earth, natural transmutations from the different mechanisms of natural nuclear reactions occur, due to cosmic ray bombardment of elements (for example, to form carbon-14), and also occasionally from natural neutron bombardment (for example, see natural nuclear fission reactor).
Artificial transmutation may occur in machinery that has enough energy to cause changes in the nuclear structure of the elements. Such machines include particle accelerators and tokamak reactors. Conventional fission power reactors also cause artificial transmutation, not from the power of the machine, but by exposing elements to neutrons produced by fission from an artificially produced nuclear chain reaction. For instance, when a uranium atom is bombarded with slow neutrons, fission takes place. This releases, on average, 3 neutrons and a large amount of energy. The released neutrons then cause fission of other uranium atoms, until all of the available uranium is exhausted. This is called a chain reaction.
Artificial nuclear transmutation has been considered as a possible mechanism for reducing the volume and hazard of radioactive waste.[2]
https://en.wikipedia.org/wiki/Nuclear_transmutation
Stellar nucleosynthesis is the creation (nucleosynthesis) of chemical elements by nuclear fusion reactions within stars. Stellar nucleosynthesis has occurred since the original creation of hydrogen, helium and lithium during the Big Bang. As a predictive theory, it yields accurate estimates of the observed abundances of the elements. It explains why the observed abundances of elements change over time and why some elements and their isotopes are much more abundant than others. The theory was initially proposed by Fred Hoyle in 1946,[1] who later refined it in 1954.[2] Further advances were made, especially to nucleosynthesis by neutron capture of the elements heavier than iron, by Margaret and Geoffrey Burbidge, William Alfred Fowler and Hoyle in their famous 1957 B2FH paper,[3] which became one of the most heavily cited papers in astrophysics history.
Stars evolve because of changes in their composition (the abundance of their constituent elements) over their lifespans, first by burning hydrogen(main sequence star), then helium (horizontal branch star), and progressively burning higher elements. However, this does not by itself significantly alter the abundances of elements in the universe as the elements are contained within the star. Later in its life, a low-mass star will slowly eject its atmosphere via stellar wind, forming a planetary nebula, while a higher–mass star will eject mass via a sudden catastrophic event called a supernova. The term supernova nucleosynthesis is used to describe the creation of elements during the explosion of a massive star or white dwarf.
The advanced sequence of burning fuels is driven by gravitational collapse and its associated heating, resulting in the subsequent burning of carbon, oxygen and silicon. However, most of the nucleosynthesis in the mass range A = 28–56 (from silicon to nickel) is actually caused by the upper layers of the star collapsing onto the core, creating a compressional shock wave rebounding outward. The shock front briefly raises temperatures by roughly 50%, thereby causing furious burning for about a second. This final burning in massive stars, called explosive nucleosynthesis or supernova nucleosynthesis, is the final epoch of stellar nucleosynthesis.
A stimulus to the development of the theory of nucleosynthesis was the discovery of variations in the abundances of elements found in the universe. The need for a physical description was already inspired by the relative abundances of the chemical elements in the solar system. Those abundances, when plotted on a graph as a function of the atomic number of the element, have a jagged sawtooth shape that varies by factors of tens of millions (see history of nucleosynthesis theory).[4] This suggested a natural process that is not random. A second stimulus to understanding the processes of stellar nucleosynthesis occurred during the 20th century, when it was realized that the energy released from nuclear fusion reactions accounted for the longevity of the Sun as a source of heat and light.[5]
https://en.wikipedia.org/wiki/Stellar_nucleosynthesis
In 1980, he transmuted several thousand atoms of bismuth into gold at the Lawrence Berkeley Laboratory. His experimental technique, using nuclear physics, was able to remove protons and neutrons from the bismuth atoms. Seaborg's technique would have been far too expensive to enable routine manufacturing of gold, but his work was close to the mythical Philosopher's Stone.[45][46]
https://en.wikipedia.org/wiki/Glenn_T._Seaborg#Return_to_California
In physics, gravitational acceleration is the acceleration of an object in free fall within a vacuum (and thus without experiencing drag). This is the steady gain in speed caused exclusively by the force of gravitational attraction. At a fixed point on the Earth's surface, all bodies accelerate in vacuum at the same rate, regardless of the masses or compositions of the bodies;[1] the measurement and analysis of these rates is known as gravimetry.
At different points on Earth's surface, the free fall acceleration ranges from 9.764 m/s2 to 9.834 m/s2[2] depending on altitude, latitude, and longitude. A conventional standard value is defined exactly as 9.80665 m/s2 (approximately 32.17405 ft/s2). Locations of significant variation from this value are known as gravity anomalies. This does not take into account other effects, such as buoyancy or drag.
https://en.wikipedia.org/wiki/Gravitational_acceleration
In the thermodynamics of equilibrium, a state function, function of state, or point function is a function defined for a system relating several state variables or state quantities that depends only on the current equilibrium thermodynamic state of the system[1] (e.g. gas, liquid, solid, crystal, or emulsion), not the path which the system took to reach its present state. A state function describes the equilibrium state of a system, thus also describing the type of system. For example, a state function could describe an atom or molecule in a gaseous, liquid, or solid form; a heterogeneous or homogeneous mixture; and the amounts of energy required to create such systems or change them into a different equilibrium state.
Heat, enthalpy, and entropy are examples of state quantities because they quantitatively describe an equilibrium state of a thermodynamic system, regardless of how the system arrived in that state. In contrast, mechanical work and heat are process quantities or path functions because their values depend on the specific "transition" (or "path") between two equilibrium states. Heat (in certain discrete amounts) can describe a state function such as enthalpy, but in general, does not truly describe the system unless it is defined as the state function of a certain system, and thus enthalpy is described by an amount of heat. This can also apply to entropy when heat is compared to temperature. The description breaks down for quantities exhibiting hysteresis.[2]
https://en.wikipedia.org/wiki/State_function
Statics is the branch of mechanics that is concerned with the analysis of (force and torque, or "moment") acting on physical systems that do not experience an acceleration (a=0), but rather, are in static equilibrium with their environment. The application of Newton's second law to a system gives:
Where bold font indicates a vector that has magnitude and direction. is the total of the forces acting on the system, is the mass of the system and is the acceleration of the system. The summation of forces will give the direction and the magnitude of the acceleration and will be inversely proportional to the mass. The assumption of static equilibrium of = 0 leads to:
The summation of forces, one of which might be unknown, allows that unknown to be found. So when in static equilibrium, the acceleration of the system is zero and the system is either at rest, or its center of mass moves at constant velocity. Likewise the application of the assumption of zero acceleration to the summation of moments acting on the system leads to:
Here, is the summation of all moments acting on the system, is the moment of inertia of the mass and = 0 the angular acceleration of the system, which when assumed to be zero leads to:
The summation of moments, one of which might be unknown, allows that unknown to be found. These two equations together, can be applied to solve for as many as two loads (forces and moments) acting on the system.
From Newton's first law, this implies that the net force and net torque on every part of the system is zero. The net forces equaling zero is known as the first condition for equilibrium, and the net torque equaling zero is known as the second condition for equilibrium. See statically indeterminate.
https://en.wikipedia.org/wiki/Statics
https://en.wikipedia.org/wiki/Component
https://en.wikipedia.org/wiki/Exergy_efficiency
https://en.wikipedia.org/wiki/Absolute_zero
https://en.wikipedia.org/wiki/Absolute_zero#Thermodynamics_near_absolute_zero
https://en.wikipedia.org/wiki/Adiabatic_process
https://en.wikipedia.org/wiki/Environment_(systems)
https://en.wikipedia.org/wiki/Thermodynamic_system
https://en.wikipedia.org/wiki/Steam-electric_power_station
https://en.wikipedia.org/wiki/Enthalpy–entropy_chart
https://en.wikipedia.org/wiki/Deposition_(phase_transition)
https://en.wikipedia.org/wiki/Sublimation_(phase_transition)
https://en.wikipedia.org/wiki/Endothermic_process
https://en.wikipedia.org/wiki/Exothermic_process
https://en.wikipedia.org/wiki/Enthalpy
https://en.wikipedia.org/wiki/Bond_energy
https://en.wikipedia.org/wiki/Thermal_energy
https://en.wikipedia.org/wiki/Potential_energy
https://en.wikipedia.org/wiki/Kinetic_energy
https://en.wikipedia.org/wiki/Molecule
https://en.wikipedia.org/wiki/Heat
https://en.wikipedia.org/wiki/Energy
https://en.wikipedia.org/wiki/Work_(thermodynamics)
https://en.wikipedia.org/wiki/State_function
https://en.wikipedia.org/wiki/Process_function
https://en.wikipedia.org/wiki/List_of_thermodynamic_properties
https://en.wikipedia.org/wiki/Partial_pressure
https://en.wikipedia.org/wiki/Equilibrium_constant
https://en.wikipedia.org/wiki/Determination_of_equilibrium_constants
https://en.wikipedia.org/wiki/State_of_matter
https://en.wikipedia.org/wiki/Physical_property
https://en.wikipedia.org/wiki/Phase_transition
https://en.wikipedia.org/wiki/Sublimation_(phase_transition)
https://en.wikipedia.org/wiki/Physical_change
https://en.wikipedia.org/wiki/Enthalpy_of_fusion
https://en.wikipedia.org/wiki/Specific_heat_capacity
https://en.wikipedia.org/wiki/Chemistry
https://en.wikipedia.org/wiki/Thermodynamics
https://en.wikipedia.org/wiki/Category:Equilibrium_chemistry
https://en.wikipedia.org/wiki/Non-equilibrium_thermodynamics
https://en.wikipedia.org/wiki/Equilibrium_thermodynamics
https://en.wikipedia.org/wiki/Thermodynamic_system
https://en.wikipedia.org/wiki/Environment_(systems)
https://en.wikipedia.org/wiki/Intensive_and_extensive_properties
https://en.wikipedia.org/wiki/Fundamental_thermodynamic_relation
https://en.wikipedia.org/wiki/Energy_system
https://en.wikipedia.org/wiki/Chemical_potential
https://en.wikipedia.org/wiki/Stochastic
https://en.wikipedia.org/wiki/Entropy_(energy_dispersal)
https://en.wikipedia.org/wiki/Thermodynamic_equilibrium
https://en.wikipedia.org/wiki/Thermodynamic_operation
https://en.wikipedia.org/wiki/Thermodynamic_process
https://en.wikipedia.org/wiki/Thermodynamic_cycle
https://en.wikipedia.org/wiki/First_law_of_thermodynamics_(fluid_mechanics)
https://en.wikipedia.org/wiki/Combustion_chemical_vapor_deposition
https://en.wikipedia.org/wiki/Atomic_layer_deposition
https://en.wikipedia.org/wiki/Plasma-enhanced_chemical_vapor_deposition
https://en.wikipedia.org/wiki/Deposition_(phase_transition)
https://en.wikipedia.org/wiki/Laws_of_thermodynamics
https://en.wikipedia.org/wiki/Thermodynamic_system
https://en.wikipedia.org/wiki/Material_properties_(thermodynamics)
https://en.wikipedia.org/wiki/List_of_thermodynamic_properties
https://en.wikipedia.org/wiki/Thermodynamic_state
https://en.wikipedia.org/wiki/Thermodynamic_diagrams
https://en.wikipedia.org/wiki/Entropy
https://en.wikipedia.org/wiki/SI_base_unit
https://en.wikipedia.org/wiki/Thermodynamic_instruments
https://en.wikipedia.org/wiki/State_function
https://en.wikipedia.org/wiki/State_space
https://en.wikipedia.org/wiki/Monatomic_gas
https://en.wikipedia.org/wiki/State_postulate
https://en.wikipedia.org/wiki/Process_function
A thermodynamic instrument is any device which facilitates the quantitative measurement of thermodynamic systems. In order for a thermodynamic parameter to be truly defined, a technique for its measurement must be specified. For example, the ultimate definition of temperature is "what a thermometer reads". The question follows – what is a thermometer?
There are two types of thermodynamic instruments, the meter and the reservoir. A thermodynamic meter is any device which measures any parameter of a thermodynamic system. A thermodynamic reservoir is a system which is so large that it does not appreciably alter its state parameters when brought into contact with the test system.
Thermodynamic meters[edit]
A meter is a thermodynamic system which displays some aspect of its thermodynamic state to the observer. The nature of its contact with the system it is measuring can be controlled, and it is sufficiently small that it does not appreciably affect the state of the system being measured. The theoretical thermometer described below is just such a meter.
In some cases, the thermodynamic parameter is actually defined in terms of an idealized measuring instrument. For example, the zeroth law of thermodynamics states that if two bodies are in thermal equilibrium with a third body, they are also in thermal equilibrium with each other. This principle, as noted by James Maxwell in 1872, asserts that it is possible to measure temperature. An idealized thermometer is a sample of an ideal gas at constant pressure. From the ideal gas law, the volume of such a sample can be used as an indicator of temperature; in this manner it defines temperature. Although pressure is defined mechanically, a pressure-measuring device called a barometer may also be constructed from a sample of an ideal gas held at a constant temperature. A calorimeter is a device which is used to measure and define the internal energy of a system.
Some common thermodynamic meters are:
- Thermometer - a device which measures temperature as described above
- Barometer - a device which measures pressure. An ideal gas barometer may be constructed by mechanically connecting an ideal gas to the system being measured, while thermally insulating it. The volume will then measure pressure, by the ideal gas equation P=NkT/V .
- Calorimeter - a device which measures the heat energy added to a system. A simple calorimeter is simply a thermometer connected to a thermally isolated system.
Thermodynamic reservoirs[edit]
A reservoir is a thermodynamic system which controls the state of a system, usually by "imposing" itself upon the system being controlled. This means that the nature of its contact with the system can be controlled. A reservoir is so large that its thermodynamic state is not appreciably affected by the state of the system being controlled. The term "atmospheric pressure" in the below description of a theoretical thermometer is essentially a "pressure reservoir" which imposes atmospheric pressure upon the thermometer.
Some common reservoirs are:
- Pressure reservoir - by far the most common pressure reservoir is the Earth's atmosphere.
- Temperature reservoir - A large quantity of water at its triple point forms an effective temperature reservoir.
Theory[edit]
Let's assume that we understand mechanics well enough to understand and measure volume, area, mass, and force. These may be combined to understand the concept of pressure, which is force per unit area and density, which is mass per unit volume. It has been experimentally determined that, at low enough pressures and densities, all gases behave as ideal gases. The behavior of an ideal gas is given by the ideal gas law:
where P is pressure, V is volume, N is the number of particles (total mass divided by mass per particle), k is Boltzmann's constant, and T is temperature. In fact, this equation is more than a phenomenological equation, it gives an operational, or experimental, definition of temperature. A thermometer is a tool that measures temperature - a primitive thermometer would simply be a small container of an ideal gas, that was allowed to expand against atmospheric pressure. If we bring it into thermal contact with the system whose temperature we wish to measure, wait until it equilibrates, and then measure the volume of the thermometer, we will be able to calculate the temperature of the system in question via T=PV/Nk. Hopefully, the thermometer will be small enough that it does not appreciably alter the temperature of the system it is measuring, and also that the atmospheric pressure is not affected by the expansion of the thermometer.
The ideal gas thermometer can be defined more precisely by saying it is a system containing an ideal gas, which is thermally connected to the system it is measuring, while being dynamically and materially insulated from it. It is simultaneously dynamically connected to an external pressure reservoir, from which it is materially and thermally insulated. Other thermometers (e.g. mercury thermometers, which display the volume of mercury to the observer) may now be constructed, and calibrated against the ideal gas thermometer.
https://en.wikipedia.org/wiki/Thermodynamic_instruments
A gas thermometer is a thermometer that measures temperature by the variation in volume or pressure of a gas.[1]
Volume Thermometer[edit]
This thermometer functions by Charles's Law. Charles's Law states that when the temperature of a gas increases, so does the volume. [2]
Using Charles's Law, the temperature can be measured by knowing the volume of gas at a certain temperature by using the formula, written below. Translating it to the correct levels of the device that is holding the gas. This works on the same principle as mercury thermometers.
or
is the volume,
is the thermodynamic temperature,
is the constant for the system.
is not a fixed constant across all systems and therefore needs to be found experimentally for a given system through testing with known temperature values.
Pressure Thermometer and Absolute Zero
The constant volume gas thermometer plays a crucial role in understanding how absolute zero could be discovered long before the advent of cryogenics. Consider a graph of pressure versus temperature made not far from standard conditions (well above absolute zero) for three different samples of any ideal gas (a, b, c). To the extent that the gas is ideal, the pressure depends linearly on temperature, and the extrapolation to zero pressure occurs at absolute zero.[3] Note that data could have been collected with three different amounts of the same gas, which would have rendered this experiment easy to do in the eighteenth century.
https://en.wikipedia.org/wiki/Gas_thermometer
https://en.wikipedia.org/wiki/Category:Thermometers
https://en.wikipedia.org/wiki/Category:Gases
https://en.wikipedia.org/wiki/Temperature
https://en.wikipedia.org/wiki/Pressure
https://en.wikipedia.org/wiki/Volume
https://en.wikipedia.org/wiki/International_System_of_Units
https://en.wikipedia.org/wiki/Periodic_table
https://en.wikipedia.org/wiki/Algebra
https://en.wikipedia.org/wiki/Trigonometry
https://en.wikipedia.org/wiki/Geometry
https://en.wikipedia.org/wiki/Calculus
https://en.wikipedia.org/wiki/Integral
https://en.wikipedia.org/wiki/Vector_calculus
https://en.wikipedia.org/wiki/Arithmetic
https://en.wikipedia.org/wiki/Algebraic_expression
https://en.wikipedia.org/wiki/Quadratic_equation
https://en.wikipedia.org/wiki/Order_of_operations
https://en.wikipedia.org/wiki/Algebraic_function
https://en.wikipedia.org/wiki/Elementary_algebra
https://en.wikipedia.org/wiki/Category:Elementary_mathematics
https://en.wikipedia.org/wiki/Logarithm
https://en.wikipedia.org/wiki/Trigonometric_functions
https://en.wikipedia.org/wiki/Exponentiation
https://en.wikipedia.org/wiki/Fundamental_theorem_of_arithmetic
https://en.wikipedia.org/wiki/Arithmetic#Arithmetic_operations
https://en.wikipedia.org/wiki/Glossary_of_mathematical_symbols
https://en.wikipedia.org/wiki/List_of_mathematical_constants
https://en.wikipedia.org/wiki/Operator_(mathematics)
https://en.wikipedia.org/wiki/Operation_(mathematics)
https://en.wikipedia.org/wiki/Boyle%27s_law
https://en.wikipedia.org/wiki/Ideal_gas_law#Combined_gas_law
https://en.wikipedia.org/wiki/Avogadro%27s_law
https://en.wikipedia.org/wiki/Ideal_gas_law
https://en.wikipedia.org/wiki/Ideal_gas_law#Combined_gas_law
History[edit]
Origin of the concept[edit]
The Avogadro constant is named after the Italian scientist Amedeo Avogadro (1776–1856), who, in 1811, first proposed that the volume of a gas (at a given pressure and temperature) is proportional to the number of atoms or molecules regardless of the nature of the gas.[16]
The name Avogadro's number was coined in 1909 by the physicist Jean Perrin, who defined it as the number of molecules in exactly 32 grams of oxygen.[7] The goal of this definition was to make the mass of a mole of a substance, in grams, be numerically equal to the mass of one molecule relative to the mass of the hydrogen atom; which, because of the law of definite proportions, was the natural unit of atomic mass, and was assumed to be 1/16 of the atomic mass of oxygen.
First measurements[edit]
The value of Avogadro's number (not yet known by that name) was first obtained indirectly by Josef Loschmidt in 1865, by estimating the number of particles in a given volume of gas.[14] This value, the number density n0 of particles in an ideal gas, is now called the Loschmidt constant in his honor, and is related to the Avogadro constant, NA, by
where p0 is the pressure, R is the gas constant, and T0 is the absolute temperature. Because of this work, the symbol L is sometimes used for the Avogadro constant,[17] and, in German literature, that name may be used for both constants, distinguished only by the units of measurement.[18] (However, NA should not be confused with the entirely different Loschmidt constant in English-language literature.)
Perrin himself determined Avogadro's number by several different experimental methods. He was awarded the 1926 Nobel Prize in Physics, largely for this work.[19]
The electric charge per mole of electrons is a constant called the Faraday constant and has been known since 1834, when Michael Faraday published his works on electrolysis. In 1910, Robert Millikan obtained the first measurement of the charge on an electron. Dividing the charge on a mole of electrons by the charge on a single electron provided a more accurate estimate of the Avogadro number.[20]
SI definition of 1971[edit]
In 1971 the International Bureau of Weights and Measures (BIPM) decided to regard the amount of substance as an independent dimension of measurement, with the mole as its base unit in the International System of Units (SI).[17] Specifically, the mole was defined as an amount of a substance that contains as many elementary entities as there are atoms in 0.012 kilograms of carbon-12.
By this definition, the common rule of thumb that "one gram of matter contains N0 nucleons" was exact for carbon-12, but slightly inexact for other elements and isotopes. On the other hand, one mole of any substance contained exactly as many molecules as one mole of any other substance.
As a consequence of this definition, in the SI system the Avogadro constant NA had the dimensionality of reciprocal of amount of substance rather than of a pure number, and had the approximate value 6.02×1023 with units of mol−1.[17] By this definition, the value of NA inherently had to be determined experimentally.
The BIPM also named NA the "Avogadro constant", but the term "Avogadro number" continued to be used especially in introductory works.[21]
SI redefinition of 2019[edit]
In 2017, the BIPM decided to change the definitions of mole and amount of substance.[22][4] The mole was redefined as being the amount of substance containing exactly 6.02214076×1023 elementary entities. One consequence of this change is that the mass of a mole of 12C atoms is no longer exactly 0.012 kg. On the other hand, the dalton (a.k.a. universal atomic mass unit) remains unchanged as 1/12 of the mass of 12C.[23][24] Thus, the molar mass constant is no longer exactly 1 g/mol, although the difference (4.5×10−10 in relative terms, as of March 2019) is insignificant for practical purposes.[4][1]
Connection to other constants[edit]
The Avogadro constant, NA is related to other physical constants and properties.
- It relates the molar gas constant R and the Boltzmann constant kB, which in the SI (since 20 May 2019) is defined to be exactly 1.380649×10−23 J/K:[4]
- = 8.31446261815324 J⋅K−1⋅mol−1
- It relates the Faraday constant F and the elementary charge e, which in the SI (since 20 May 2019) is defined as exactly 1.602176634×10−19 coulombs:[4]
- = 96485.3321233100184 C/mol
- It relates the molar mass constant, Mu and the atomic mass constant mu, currently 1.66053906660(50)×10−27 kg:[25]
- = 0.99999999965(30)×10−3 kg⋅mol−1[26]
A thermodynamic temperature reading of zero denotes the point at which the fundamental physical property that imbues matter with a temperature, transferable kinetic energy due to atomic motion, begins. In science, thermodynamic temperature is measured on the Kelvin scale and the unit of measure is the kelvin (unit symbol: K). For comparison, a temperature of 295 K is a comfortable one, equal to 21.85 °C and 71.33 °F.
At the zero point of thermodynamic temperature, absolute zero, the particle constituents of matter have minimal motion and can become no colder.[1][2] Absolute zero, which is a temperature of zero kelvin (0 K), is precisely equal to −273.15 °C and −459.67 °F. Matter at absolute zero has no remaining transferable average kinetic energy and the only remaining particle motion is due to an ever-pervasive quantum mechanical phenomenon called zero-point energy.[3]
https://en.wikipedia.org/wiki/Thermodynamic_temperature
Zero-point energy (ZPE) is the lowest possible energy that a quantum mechanical system may have. Unlike in classical mechanics, quantum systems constantly fluctuate in their lowest energy state as described by the Heisenberg uncertainty principle.[1] As well as atoms and molecules, the empty space of the vacuum has these properties. According to quantum field theory, the universe can be thought of not as isolated particles but continuous fluctuating fields: matter fields, whose quanta are fermions (i.e., leptons and quarks), and force fields, whose quanta are bosons (e.g., photons and gluons). All these fields have zero-point energy.[2] These fluctuating zero-point fields lead to a kind of reintroduction of an aether in physics[1][3] since some systems can detect the existence of this energy. However, this aether cannot be thought of as a physical medium if it is to be Lorentz invariant such that there is no contradiction with Einstein's theory of special relativity.[1]
Physics currently lacks a full theoretical model for understanding zero-point energy; in particular, the discrepancy between theorized and observed vacuum energy is a source of major contention.[4] Physicists Richard Feynman and John Wheeler calculated the zero-point radiation of the vacuum to be an order of magnitude greater than nuclear energy, with a single light bulb containing enough energy to boil all the world's oceans.[5] Yet according to Einstein's theory of general relativity, any such energy would gravitate and the experimental evidence from both the expansion of the universe, dark energy and the Casimir effect shows any such energy to be exceptionally weak. A popular proposal that attempts to address this issue is to say that the fermion field has a negative zero-point energy, while the boson field has positive zero-point energy and thus these energies somehow cancel each other out.[6][7] This idea would be true if supersymmetry were an exact symmetry of nature; however, the LHC at CERN has so far found no evidence to support it. Moreover, it is known that if supersymmetry is valid at all, it is at most a broken symmetry, only true at very high energies, and no one has been able to show a theory where zero-point cancellations occur in the low energy universe we observe today.[7] This discrepancy is known as the cosmological constant problem and it is one of the greatest unsolved mysteries in physics. Many physicists believe that "the vacuum holds the key to a full understanding of nature".[8]
https://en.wikipedia.org/wiki/Zero-point_energy
The quantum vacuum state or simply quantum vacuum refers to the quantum state with the lowest possible energy.
Quantum vacuum may also refer to:
- Quantum chromodynamic vacuum (QCD vacuum), a non-perturbative vacuum
- Quantum electrodynamic vacuum (QED vacuum), a field-theoretic vacuum
- Quantum vacuum (ground state), the state of lowest energy of a quantum system
- Quantum vacuum collapse, a hypothetical vacuum metastability event
- Quantum vacuum expectation value, an operator's average, expected value in a quantum vacuum
- Quantum vacuum energy, an underlying background energy that exists in space throughout the entire Universe
- The Quantum Vacuum, a physics textbook by Peter W. Milonni
See also[edit]
Formation[edit]
Formation of monatomic ions[edit]
Monatomic ions are formed by the gain or loss of electrons to the valence shell (the outer-most electron shell) in an atom. The inner shells of an atom are filled with electrons that are tightly bound to the positively charged atomic nucleus, and so do not participate in this kind of chemical interaction. The process of gaining or losing electrons from a neutral atom or molecule is called ionization.
Atoms can be ionized by bombardment with radiation, but the more usual process of ionization encountered in chemistry is the transfer of electrons between atoms or molecules. This transfer is usually driven by the attaining of stable ("closed shell") electronic configurations. Atoms will gain or lose electrons depending on which action takes the least energy.
For example, a sodium atom, Na, has a single electron in its valence shell, surrounding 2 stable, filled inner shells of 2 and 8 electrons. Since these filled shells are very stable, a sodium atom tends to lose its extra electron and attain this stable configuration, becoming a sodium cation in the process
- Na → Na+
+
e−
On the other hand, a chlorine atom, Cl, has 7 electrons in its valence shell, which is one short of the stable, filled shell with 8 electrons. Thus, a chlorine atom tends to gain an extra electron and attain a stable 8-electron configuration, becoming a chloride anion in the process:
- Cl +
e−
→ Cl−
This driving force is what causes sodium and chlorine to undergo a chemical reaction, wherein the "extra" electron is transferred from sodium to chlorine, forming sodium cations and chloride anions. Being oppositely charged, these cations and anions form ionic bonds and combine to form sodium chloride, NaCl, more commonly known as table salt.
- Na+
+ Cl−
→ NaCl
Hyperfine Structure
Qubit in ion-trap quantum computing[edit]
The hyperfine states of a trapped ion are commonly used for storing qubits in ion-trap quantum computing. They have the advantage of having very long lifetimes, experimentally exceeding ~10 minutes (compared to ~1 s for metastable electronic levels).
The frequency associated with the states' energy separation is in the microwave region, making it possible to drive hyperfine transitions using microwave radiation. However, at present no emitter is available that can be focused to address a particular ion from a sequence. Instead, a pair of laser pulses can be used to drive the transition, by having their frequency difference (detuning) equal to the required transition's frequency. This is essentially a stimulated Raman transition. In addition, near-field gradients have been exploited to individually address two ions separated by approximately 4.3 micrometers directly with microwave radiation.[16]
See also[edit]
https://en.wikipedia.org/wiki/Electron_gun
https://en.wikipedia.org/wiki/Electron_emission
https://en.wikipedia.org/wiki/Fusor
https://en.wikipedia.org/wiki/Hydrogen_maser
https://en.wikipedia.org/wiki/Hydrogen_narcosis
https://en.wikipedia.org/wiki/Quadrupole
https://en.wikipedia.org/wiki/Quadrupole_ion_trap
https://en.wikipedia.org/wiki/Nuclear_quadrupole_resonance
https://en.wikipedia.org/wiki/Pi-Stacking_(chemistry)
https://en.wikipedia.org/wiki/Quadrupole_splitting
https://en.wikipedia.org/wiki/Trihydrogen_cation
https://en.wikipedia.org/wiki/Ozone
https://en.wikipedia.org/wiki/Cyclic_ozone
https://en.wikipedia.org/wiki/Dimer_(chemistry)
https://en.wikipedia.org/wiki/Protein_dimer
https://en.wikipedia.org/wiki/Supramolecular_chemistry
https://en.wikipedia.org/wiki/Supramolecular_catalysis
https://en.wikipedia.org/wiki/Cryptand
https://en.wikipedia.org/wiki/Pnictogen_hydride
https://en.wikipedia.org/wiki/Phosphine
https://en.wikipedia.org/wiki/Dendrimer
https://en.wikipedia.org/wiki/Catenane
https://en.wikipedia.org/wiki/Spiro_compound
https://en.wikipedia.org/wiki/Linear_motor#Rapid_transits_using_linear_motor_propulsion
https://en.wikipedia.org/wiki/Linear_motor
https://en.wikipedia.org/wiki/Magnetic-core_memory
https://en.wikipedia.org/wiki/Ferrite_core
https://en.wikipedia.org/wiki/Prussian_blue
https://en.wikipedia.org/wiki/Sodium_ferrocyanide
https://en.wikipedia.org/wiki/Launch_loop
https://en.wikipedia.org/wiki/Accelerator_physics
https://en.wikipedia.org/wiki/Pyrolytic_carbon
https://en.wikipedia.org/wiki/Bismuth
https://en.wikipedia.org/wiki/Gamma_spectroscopy
https://en.wikipedia.org/wiki/Radical_cyclization
https://en.wikipedia.org/wiki/Redox
https://en.wikipedia.org/wiki/Explosive
https://en.wikipedia.org/wiki/Nitroglycerin
https://en.wikipedia.org/wiki/Cyanide
https://en.wikipedia.org/wiki/Graphene
https://en.wikipedia.org/wiki/Pressure#Negative_pressures
https://en.wikipedia.org/wiki/Cavitation
https://en.wikipedia.org/wiki/Observable_universe#Large-scale_structure
https://en.wikipedia.org/wiki/Carbon_disulfide
https://en.wikipedia.org/wiki/Negative_mass
https://en.wikipedia.org/wiki/Antimatter
https://en.wikipedia.org/wiki/Mirror_matter
https://en.wikipedia.org/wiki/Effective_mass_(spring–mass_system)
https://en.wikipedia.org/wiki/Lambda-CDM_model
https://en.wikipedia.org/wiki/Negative_mass
https://en.wikipedia.org/wiki/Linear_induction_accelerator
https://en.wikipedia.org/wiki/Angular_acceleration
https://en.wikipedia.org/wiki/0
https://en.wikipedia.org/wiki/Magnetic_scalar_potential
https://en.wikipedia.org/wiki/Accelerator_physics
https://en.wikipedia.org/wiki/Pyrolytic_carbon
https://en.wikipedia.org/wiki/Bismuth
https://en.wikipedia.org/wiki/Hyperloop
https://en.wikipedia.org/wiki/Electrodynamic_suspension#Levitation_melting
https://en.wikipedia.org/wiki/Aerodynamic_levitation
https://en.wikipedia.org/wiki/Electrostatic_levitation
Magnetic levitation
https://en.wikipedia.org/wiki/Launch_loop
https://en.wikipedia.org/wiki/Synchronous_motor
https://en.wikipedia.org/wiki/Linear_stage
https://en.wikipedia.org/wiki/Skew-symmetric_matrix
Supramolecular chemistry
09-18-2021-0806 - Spiro compounds
https://en.wikipedia.org/wiki/Compressible_flow
https://en.wikipedia.org/wiki/Shear_rate
https://en.wikipedia.org/wiki/Hyperfine_structure
https://en.wikipedia.org/wiki/Void_(astronomy)
https://en.wikipedia.org/wiki/Quintessence_(physics)
https://en.wikipedia.org/wiki/Scalar_field
https://en.wikipedia.org/wiki/Symmetric_matrix
https://en.wikipedia.org/wiki/Quadrupole
https://en.wikipedia.org/wiki/Vector_field
https://en.wikipedia.org/wiki/Preon
https://en.wikipedia.org/wiki/Pressuron
https://en.wikipedia.org/wiki/Spinor
https://en.wikipedia.org/wiki/Astrophysical_jet
https://en.wikipedia.org/wiki/Accretion_(astrophysics)
https://en.wikipedia.org/wiki/Gravitational_lens
https://en.wikipedia.org/wiki/Escape_velocity
https://en.wikipedia.org/wiki/Compressible_flow
https://en.wikipedia.org/wiki/Nuclear_magnetic_resonance
https://en.wikipedia.org/wiki/Molecular_laser_isotope_separation
https://en.wikipedia.org/wiki/Cascade_(chemical_engineering)
https://en.wikipedia.org/wiki/Nuclide
https://en.wikipedia.org/wiki/Hydroformylation
https://en.wikipedia.org/wiki/Tolman_electronic_parameter
https://en.wikipedia.org/wiki/Radioligand
https://en.wikipedia.org/wiki/Synchronous_motor
https://en.wikipedia.org/wiki/Electrodynamic_suspension#Levitation_melting
https://en.wikipedia.org/wiki/Magnetic_scalar_potential
https://en.wikipedia.org/wiki/Sublimation_(phase_transition)
reactive centrifugal force, a real inertial-frame-independent Newtonian force that exists as a reaction to a centripetal force.
https://en.wikipedia.org/wiki/Centrifugal_force
https://en.wikipedia.org/wiki/Torque
https://en.wikipedia.org/wiki/Acceleration
https://en.wikipedia.org/wiki/Velocity
https://en.wikipedia.org/wiki/Force
https://en.wikipedia.org/wiki/Energy
https://en.wikipedia.org/wiki/Time
https://en.wikipedia.org/wiki/Speed
https://en.wikipedia.org/wiki/Space
https://en.wikipedia.org/wiki/Distance
https://en.wikipedia.org/wiki/Orientation_(geometry)
https://en.wikipedia.org/wiki/Scalar_(physics)
https://en.wikipedia.org/wiki/Scalar_field
https://en.wikipedia.org/wiki/Spinor_field
https://en.wikipedia.org/wiki/Gradient
https://en.wikipedia.org/wiki/Spinor_field
https://en.wikipedia.org/wiki/Spin_structure#Spin_structures_on_vector_bundles
https://en.wikipedia.org/wiki/Magnitude
https://en.wikipedia.org/wiki/Direction
https://en.wikipedia.org/wiki/Vector_(mathematics_and_physics)
https://en.wikipedia.org/wiki/Synthetic_geometry
https://en.wikipedia.org/wiki/Spin_geometry
https://en.wikipedia.org/wiki/Frame_bundle#Orthonormal_frame_bundle
https://en.wikipedia.org/wiki/Trigonometry
https://en.wikipedia.org/wiki/Calculus
https://en.wikipedia.org/wiki/Linear_algebra
https://en.wikipedia.org/wiki/Topological_space
https://en.wikipedia.org/wiki/Set_theory
https://en.wikipedia.org/wiki/Number_theory
https://en.wikipedia.org/wiki/Bayesian_statistics
https://en.wikipedia.org/wiki/Probability_distribution
https://en.wikipedia.org/wiki/Probability_theory
https://en.wikipedia.org/wiki/Statistics
https://en.wikipedia.org/wiki/List_of_equations
https://en.wikipedia.org/wiki/List_of_common_physics_notations
https://en.wikipedia.org/wiki/Greek_letters_used_in_mathematics,_science,_and_engineering
https://en.wikipedia.org/wiki/Zero-dimensional_space
https://en.wikipedia.org/wiki/N-sphere
https://en.wikipedia.org/wiki/Tesseract
https://en.wikipedia.org/wiki/Fractal
https://en.wikipedia.org/wiki/Dimension
https://en.wikipedia.org/wiki/Glossary_of_topology
https://en.wikipedia.org/wiki/Hausdorff_dimension
https://en.wikipedia.org/wiki/Point-finite_collection
https://en.wikipedia.org/wiki/Fluid_dynamics
https://en.wikipedia.org/wiki/Elasticity_(physics)
https://en.wikipedia.org/wiki/Surface_tension
https://en.wikipedia.org/wiki/Shear_stress
https://en.wikipedia.org/wiki/Thermodynamic_free_energy
https://en.wikipedia.org/wiki/Wulff_construction
https://en.wikipedia.org/wiki/Momentum
https://en.wikipedia.org/wiki/Angular_momentum
https://en.wikipedia.org/wiki/Hydrostatics
https://en.wikipedia.org/wiki/Supercritical_fluid
https://en.wikipedia.org/wiki/Hydrostatics
https://en.wikipedia.org/wiki/Pressure
https://en.wikipedia.org/wiki/Adhesion
https://en.wikipedia.org/wiki/Plasma_(physics)
https://en.wikipedia.org/wiki/Rheology
https://en.wikipedia.org/wiki/Continuum_mechanics
https://en.wikipedia.org/wiki/Shear_force
https://en.wikipedia.org/wiki/Solid_mechanics
https://en.wikipedia.org/wiki/Cauchy_stress_tensor#Stress_deviator_tensor
https://en.wikipedia.org/wiki/Critical_plane_analysis
https://en.wikipedia.org/wiki/Deformation_(physics)
https://en.wikipedia.org/wiki/Category:Fracture_mechanics
https://en.wikipedia.org/wiki/Category:Materials_degradation
https://en.wikipedia.org/wiki/Category:Mechanical_failure
https://en.wikipedia.org/wiki/Christensen_failure_criterion
https://en.wikipedia.org/wiki/Fluid
https://en.wikipedia.org/wiki/Scientific_law
https://en.wikipedia.org/wiki/Aerodynamics
https://en.wikipedia.org/wiki/Compressible_flow
https://en.wikipedia.org/wiki/Incompressible_flow
https://en.wikipedia.org/wiki/Isentropic_process
https://en.wikipedia.org/wiki/Thermodynamics
https://en.wikipedia.org/wiki/Hygroscopic_cycle
https://en.wikipedia.org/wiki/Hygroscopy
https://en.wikipedia.org/wiki/Desiccant
https://en.wikipedia.org/wiki/Wax_fire
https://en.wikipedia.org/wiki/Cyclic_ozone
https://en.wikipedia.org/wiki/Cyaphide
https://en.wikipedia.org/wiki/Cyanogen
https://en.wikipedia.org/wiki/Copper(II)_sulfate
https://en.wikipedia.org/wiki/Dicyanoacetylene
https://en.wikipedia.org/wiki/Oxamide
https://en.wikipedia.org/wiki/Graphite
https://en.wikipedia.org/wiki/Beryllium
https://en.wikipedia.org/wiki/Barium
https://en.wikipedia.org/wiki/Caesium
https://en.wikipedia.org/wiki/Rubidium
https://en.wikipedia.org/wiki/Cobalt
https://en.wikipedia.org/wiki/Cobalt_blue
https://en.wikipedia.org/wiki/Prussian_blue
https://en.wikipedia.org/wiki/Cyanide
https://en.wikipedia.org/wiki/Colloid
https://en.wikipedia.org/wiki/Ferrocyanide
https://en.wikipedia.org/wiki/Phosphorus
https://en.wikipedia.org/wiki/Phosphorous
https://en.wikipedia.org/wiki/Thallium#Thallium(I)
https://en.wikipedia.org/wiki/Protium
https://en.wikipedia.org/wiki/Neutronium
https://en.wikipedia.org/wiki/Strange_matter
https://en.wikipedia.org/wiki/Neutron_magnetic_moment
https://en.wikipedia.org/wiki/R-process
https://en.wikipedia.org/wiki/Neutron_microscope
https://en.wikipedia.org/wiki/Mirror_nuclei
https://en.wikipedia.org/wiki/Neutron_generator
https://en.wikipedia.org/wiki/Mirror_matter
https://en.wikipedia.org/wiki/Neutron_supermirror
https://en.wikipedia.org/wiki/Neutron_emission
https://en.wikipedia.org/wiki/Weak_interaction
https://en.wikipedia.org/wiki/Neutron–proton_ratio
https://en.wikipedia.org/wiki/Fusion_power#Magnetic_Mirror
https://en.wikipedia.org/wiki/Nuclear_drip_line
https://en.wikipedia.org/wiki/Table_of_nuclides
https://en.wikipedia.org/wiki/Valley_of_stability
https://en.wikipedia.org/wiki/Beta-decay_stable_isobars
https://en.wikipedia.org/wiki/Nuclear_binding_energy
https://en.wikipedia.org/wiki/Neutron_magnetic_moment
https://en.wikipedia.org/wiki/Positron_emission
https://en.wikipedia.org/wiki/Nuclear_fission_product
https://en.wikipedia.org/wiki/Radioactive_waste
https://en.wikipedia.org/wiki/Compact_toroid
Fusion power is a proposed form of power generation that would generate electricity by using heat from nuclear fusion reactions. In a fusion process, two lighter atomic nuclei combine to form a heavier nucleus, while releasing energy. Devices designed to harness this energy are known as fusion reactors.
Plasma behavior[edit]
Plasma is an ionized gas that conducts electricity.[10] In bulk, it is modeled using magnetohydrodynamics, which is a combination of the Navier–Stokes equations governing fluids and Maxwell's equations governing how magnetic and electric fields behave.[11] Fusion exploits several plasma properties, including:
- Beam fusion: A beam of high energy particles fired at another beam or target can initiate fusion. This was used in the 1970s and 1980s to study the cross sections of fusion reactions.[3]However beam systems cannot be used for power because keeping a beam coherent takes more energy than comes from fusion.
- Muon-catalyzed fusion: This approach replaces electrons in diatomic molecules of isotopes of hydrogen with muons - more massive particles with the same electric charge. Their greater mass compresses the nuclei enough such that the strong interaction can cause fusion.[40] As of 2007 producing muons required more energy than can be obtained from muon-catalyzed fusion.[41]
- Radio frequency heating: a radio wave causes the plasma to oscillate (i.e., microwave oven). This is also known as electron cyclotron resonance heating or dielectric heating.[45]
- Flux loop: a loop of wire is inserted into the magnetic field. As the field passes through the loop, a current is made. The current measures the total magnetic flux through that loop. This has been used on the National Compact Stellarator Experiment,[49] the polywell,[50] and the LDX machines. A Langmuir probe, a metal object placed in a plasma, can be employed. A potential is applied to it, giving it a voltage against the surrounding plasma. The metal collects charged particles, drawing a current. As the voltage changes, the current changes. This makes an IV Curve. The IV-curve can be used to determine the local plasma density, potential and temperature.[51]
- Steam turbines can be driven by heat transferred into a working fluid that turns into steam, driving electric generators.[58]
- Neutron blankets: These neutrons can regenerate spent fission fuel.[59] Tritium can be produced using a breeder blanket comprised of liquid lithium or a helium cooled pebble bed made of lithium-bearing ceramic pebbles.[60]
- Direct conversion: The kinetic energy of a particle can be converted into voltage.[18] It was first suggested by Richard F. Post in conjunction with magnetic mirrors, in the late 1960s. It has been proposed for Field-Reversed Configurations as well as Dense Plasma Focus devices. The process converts a large fraction of the random energy of the fusion products into directed motion. The particles are then collected on electrodes at various large electrical potentials. This method has demonstrated an experimental efficiency of 48 percent.[61]
Magnetic confinement[edit]
Magnetic Mirror[edit]
Magnetic mirror effect. If a particle follows the field line and enters a region of higher field strength, the particles can be reflected. Several devices apply this effect. The most famous was the magnetic mirror machines, a series of devices built at LLNL from the 1960s to the 1980s.[62] Other examples include magnetic bottles and Biconic cusp.[63] Because the mirror machines were straight, they had some advantages over ring-shaped designs. The mirrors were easier to construct and maintain and direct conversion energy capture was easier to implement.[9] Poor confinement led this approach to be abandoned, except in the polywell design.[64]
Magnetic Loops[edit]
Magnetic loops bend the field lines back on themselves, either in circles or more commonly in nested toroidal surfaces. The most highly developed systems of this type are the tokamak, the stellarator, and the reversed field pinch. Compact toroids, especially the field-reversed configuration and the spheromak, attempt to combine the advantages of toroidal magnetic surfaces with those of a simply connected (non-toroidal) machine, resulting in a mechanically simpler and smaller confinement area.
Inertial confinement[edit]
Inertial confinement is the use of rapid implosion to heat and confine plasma. A shell surrounding the fuel is imploded using a direct laser blast (direct drive), a secondary x-ray blast (indirect drive), or heavy beams. The fuel must be compressed to about 30 times solid density with energetic beams. Direct drive can in principle be efficient, but insufficient uniformity has prevented success.[65]:19-20 Indirect drive uses beams to heat a shell, driving the shell to radiate x-rays, which then implode the pellet. The beams are commonly laser beams, but ion and electron beams have been investigated.[65]:182-193
Electrostatic confinement[edit]
Electrostatic confinement fusion devices use electrostatic fields. The best known is the fusor. This device has a cathode inside an anode wire cage. Positive ions fly towards the negative inner cage, and are heated by the electric field in the process. If they miss the inner cage they can collide and fuse. Ions typically hit the cathode, however, creating prohibitory high conduction losses. Fusion rates in fusors are low because of competing physical effects, such as energy loss in the form of light radiation.[66] Designs have been proposed to avoid the problems associated with the cage, by generating the field using a non-neutral cloud. These include a plasma oscillating device,[67] a magnetically-shielded-grid,[68] a penning trap, the polywell,[69] and the F1 cathode driver concept.[70]
https://en.wikipedia.org/wiki/Fusion_power#Magnetic_Mirror
https://nikiyaantonbettey.blogspot.com/2021/09/09-18-2021-0414-physics-notes-drafting.html
https://en.wikipedia.org/wiki/Torus
https://en.wikipedia.org/wiki/Fusion_power#Magnetic_Mirror
https://en.wikipedia.org/wiki/Simply_connected_space
https://en.wikipedia.org/wiki/X-ray
https://en.wikipedia.org/wiki/Inertial_electrostatic_confinement
https://en.wikipedia.org/wiki/Penning_trap
https://en.wikipedia.org/wiki/Polywell
https://en.wikipedia.org/wiki/Lockheed_Martin_Compact_Fusion_Reactor
https://en.wikipedia.org/wiki/Deuterium–tritium_fusion
https://en.wikipedia.org/wiki/Magnetized_target_fusion
The reaction vessel will be a fast-rotating cylinder of liquid metal (lead, incorporating lithium to harvest the tritium formed through neutron activation) which is formed into a sphere by the action of synchronised pistons driven by steam. Magnetized fusion fuel as plasma is injected into the sphere as it contracts, producing sufficient temperature and pressure for the fusion reaction to take place. The liquid metal is circulated through heat exchangers to provide steam.
https://en.wikipedia.org/wiki/Magnetized_target_fusion
https://en.wikipedia.org/wiki/Heat_exchanger
https://en.wikipedia.org/wiki/Field-reversed_configuration
https://en.wikipedia.org/wiki/Superconductivity
https://en.wikipedia.org/wiki/Magneto-inertial_fusion
https://en.wikipedia.org/wiki/Neutronium
https://en.wikipedia.org/wiki/Preon
https://en.wikipedia.org/wiki/Meson_bomb
https://en.wikipedia.org/wiki/Pressuron
https://en.wikipedia.org/wiki/Vertical_pressure_variation
https://en.wikipedia.org/wiki/Hypsometric_equation
https://en.wikipedia.org/wiki/Seabed#Depth
https://en.wikipedia.org/wiki/Marine_sediment
https://en.wikipedia.org/wiki/Trihydrogen_cation
https://en.wikipedia.org/wiki/Three-center_two-electron_bond
https://en.wikipedia.org/wiki/Dark_matter
https://en.wikipedia.org/wiki/Dark_energy
https://en.wikipedia.org/wiki/Lambda-CDM_model
https://en.wikipedia.org/wiki/Inhomogeneous_cosmology
https://en.wikipedia.org/wiki/Prussian_blue
https://en.wikipedia.org/wiki/Hydride
https://en.wikipedia.org/wiki/Hydrogen_ion
https://en.wikipedia.org/wiki/Weakly_interacting_massive_particles
https://en.wikipedia.org/wiki/Inflation_(cosmology)
https://en.wikipedia.org/wiki/Cosmic_microwave_background
https://en.wikipedia.org/wiki/Nitroglycerin
https://en.wikipedia.org/wiki/Glycerol
https://en.wikipedia.org/wiki/Propylene_oxide
https://en.wikipedia.org/wiki/Oxetane
https://en.wikipedia.org/wiki/Diol_cyclization
cyclicization ringulants
https://en.wikipedia.org/wiki/Magnetic_dipole–dipole_interaction
https://en.wikipedia.org/wiki/J-coupling
https://en.wikipedia.org/wiki/Electron_electric_dipole_moment
https://en.wikipedia.org/wiki/Zero-point_energy
https://en.wikipedia.org/wiki/Van_der_Waals_force
https://en.wikipedia.org/wiki/Zero_field_splitting
https://en.wikipedia.org/wiki/Quadrupole
https://en.wikipedia.org/wiki/Quadrupole#Gravitational_quadrupole
https://en.wikipedia.org/wiki/Trace_(linear_algebra)
https://en.wikipedia.org/wiki/Overtone_band
https://en.wikipedia.org/wiki/Near-infrared_spectroscopy
https://en.wikipedia.org/wiki/Doppler_effect
https://en.wikipedia.org/wiki/Inflation_(cosmology)
https://en.wikipedia.org/wiki/Quintessence_(physics)
https://en.wikipedia.org/wiki/Lambda-CDM_model
https://en.wikipedia.org/wiki/Heliosphere#Heliopause
https://en.wikipedia.org/wiki/Entropic_gravity
https://en.wikipedia.org/wiki/Fundamental_interaction
https://en.wikipedia.org/wiki/List_of_particles
https://en.wikipedia.org/wiki/Chameleon_particle
https://en.wikipedia.org/wiki/Axion
https://en.wikipedia.org/wiki/Graviphoton
https://en.wikipedia.org/wiki/Particle_physics
https://en.wikipedia.org/wiki/Condensed_matter_physics
https://en.wikipedia.org/wiki/Particle
https://en.wikipedia.org/wiki/Preon
https://en.wikipedia.org/wiki/Weak_hypercharge
https://en.wikipedia.org/wiki/X_(charge)
https://en.wikipedia.org/wiki/SO(10)
https://en.wikipedia.org/wiki/Grand_Unified_Theory
https://en.wikipedia.org/wiki/Supersymmetry_breaking
https://en.wikipedia.org/wiki/Stochastic_differential_equation
https://en.wikipedia.org/wiki/Gauge_theory
https://en.wikipedia.org/wiki/Eigenvalues_and_eigenvectors
https://en.wikipedia.org/wiki/Zero_element#Additive_identities
https://en.wikipedia.org/wiki/Category:Isotopes_of_neutronium
https://en.wikipedia.org/wiki/Category:Neutron
https://en.wikipedia.org/wiki/Isotopes_of_hydrogen#Hydrogen-1_(Protium)
https://en.wikipedia.org/wiki/Hydrogen
https://en.wikipedia.org/wiki/Proton
https://en.wikipedia.org/wiki/Extended_periodic_table
https://en.wikipedia.org/wiki/Periodic_table
https://en.wikipedia.org/wiki/Alternative_periodic_tables
https://en.wikipedia.org/wiki/Subatomic_particle
https://en.wikipedia.org/wiki/Hypernucleus
https://en.wikipedia.org/wiki/Category:Exotic_matter
https://en.wikipedia.org/wiki/Spinor_condensate
https://en.wikipedia.org/wiki/Spiral
https://en.wikipedia.org/wiki/Circle
https://en.wikipedia.org/wiki/Triangle
https://en.wikipedia.org/wiki/Square
https://en.wikipedia.org/wiki/Point_(geometry)
https://en.wikipedia.org/wiki/Point
https://en.wikipedia.org/wiki/Line
https://en.wikipedia.org/wiki/Tractrix
https://en.wikipedia.org/wiki/Logarithmic_spiral
https://en.wikipedia.org/wiki/Loop_(graph_theory)
https://en.wikipedia.org/wiki/Loop_(topology)
https://en.wikipedia.org/wiki/Free_loop
https://en.wikipedia.org/wiki/Category:Knot_theory
https://en.wikipedia.org/wiki/Infinity
https://en.wikipedia.org/wiki/Mathematical_fallacy
https://en.wikipedia.org/wiki/Infinite_divisibility
https://en.wikipedia.org/wiki/Division_by_zero
https://en.wikipedia.org/wiki/Indeterminate_form#Indeterminate_form_0/0
https://en.wikipedia.org/wiki/Undefined_(mathematics)
https://en.wikipedia.org/wiki/Zero_to_the_power_of_zero
https://en.wikipedia.org/wiki/Category:0_(number)
https://en.wikipedia.org/wiki/Nilpotent
https://en.wikipedia.org/wiki/Zero_of_a_function
https://en.wikipedia.org/wiki/Algebraically_closed_field#Other_properties
https://en.wikipedia.org/wiki/Category:Field_(mathematics)
https://en.wikipedia.org/wiki/Ground_field
https://en.wikipedia.org/wiki/Galois_cohomology
https://en.wikipedia.org/wiki/Profinite_group
https://en.wikipedia.org/wiki/Hilbert%27s_Theorem_90
https://en.wikipedia.org/wiki/Principal_homogeneous_space
https://en.wikipedia.org/wiki/Differentiable_manifold#Definition
https://en.wikipedia.org/wiki/Chain_rule
https://en.wikipedia.org/wiki/Malliavin_calculus
https://en.wikipedia.org/wiki/Stochastic_process
https://en.wikipedia.org/wiki/Gaussian_process
https://en.wikipedia.org/wiki/Gaussian_process_approximations
https://en.wikipedia.org/wiki/Linear_combination
https://en.wikipedia.org/wiki/Euclidean_space
https://en.wikipedia.org/wiki/Dot_product
https://en.wikipedia.org/wiki/Scalar_multiplication
https://en.wikipedia.org/wiki/Empty_set
https://en.wikipedia.org/wiki/Axiom_of_extensionality
https://en.wikipedia.org/wiki/Axiom_of_extensionality#In_set_theory_with_ur-elements
https://en.wikipedia.org/wiki/Axiom_of_regularity
https://en.wikipedia.org/wiki/Axiom_of_pairing
https://en.wikipedia.org/wiki/Gödel%27s_incompleteness_theorems#Second_incompleteness_theorem
https://en.wikipedia.org/wiki/Axiom_schema_of_replacement
https://en.wikipedia.org/wiki/Axiom_schema_of_replacement
https://en.wikipedia.org/wiki/Zermelo_set_theory
https://en.wikipedia.org/wiki/Type_theory
https://en.wikipedia.org/wiki/Ordinal_number
https://en.wikipedia.org/wiki/Limit_ordinal
https://en.wikipedia.org/wiki/Isolated_point
https://en.wikipedia.org/wiki/Topological_space
https://en.wikipedia.org/wiki/Kuratowski_closure_axioms
https://en.wikipedia.org/wiki/Unary_operation
https://en.wikipedia.org/wiki/Arity
https://en.wikipedia.org/wiki/Valency_(linguistics)
https://en.wikipedia.org/wiki/Lambda_calculus
https://en.wikipedia.org/wiki/Absolute_value
https://en.wikipedia.org/wiki/Floor_and_ceiling_functions
https://en.wikipedia.org/wiki/Arity
https://en.wikipedia.org/wiki/Earnshaw%27s_theorem#Proofs_for_magnetic_dipoles
https://en.wikipedia.org/wiki/Electrostatic_levitation
https://en.wikipedia.org/wiki/Magnetic_levitation
https://en.wikipedia.org/wiki/Magnetic_pressure
https://en.wikipedia.org/wiki/Dipole_magnet
https://en.wikipedia.org/wiki/Permeability_(electromagnetism)
https://en.wikipedia.org/wiki/Nonlinear_optics
In a nonlinear medium, the permeability can depend on the strength of the magnetic field. (space)
https://en.wikipedia.org/wiki/Permeability_(electromagnetism)
https://en.wikipedia.org/wiki/Vacuum_permeability
https://en.wikipedia.org/wiki/Centimetre–gram–second_system_of_units
https://en.wikipedia.org/wiki/Magnetostatics
https://en.wikipedia.org/wiki/Servomechanism
https://en.wikipedia.org/wiki/Eddy_current
https://en.wikipedia.org/wiki/Anisotropy
https://en.wikipedia.org/wiki/Magnetic_levitation
https://en.wikipedia.org/wiki/Dimensionless_quantity
https://en.wikipedia.org/wiki/Linear_stability
https://en.wikipedia.org/wiki/Electromagnetic_suspension
https://en.wikipedia.org/wiki/Zippe-type_centrifuge
https://en.wikipedia.org/wiki/Maglev
https://en.wikipedia.org/wiki/Drag_(physics)
https://en.wikipedia.org/wiki/Electrodynamic_suspension
https://en.wikipedia.org/wiki/Lenz%27s_law
https://en.wikipedia.org/wiki/Gyroscope
https://en.wikipedia.org/wiki/Linear_induction_motor
https://en.wikipedia.org/wiki/Pyrolytic_carbon
https://en.wikipedia.org/wiki/Bismuth
https://en.wikipedia.org/wiki/Vacuum_permeability
https://en.wikipedia.org/wiki/Ampère%27s_force_law
https://en.wikipedia.org/wiki/Biot–Savart_law
https://en.wikipedia.org/wiki/Line_integral
https://en.wikipedia.org/wiki/Parametric_equation
https://en.wikipedia.org/wiki/Linear_form
https://en.wikipedia.org/wiki/Flux
https://en.wikipedia.org/wiki/Transport_phenomena
UNIT OPERATIONS
In chemical engineering and related fields, a unit operation is a basic step in a process. Unit operations involve a physical change or chemical transformation such as separation, crystallization, evaporation, filtration, polymerization, isomerization, and other reactions. For example, in milk processing, the following unit operations are involved: homogenization, pasteurization, and packaging. These unit operations are connected to create the overall process. A process may require many unit operations to obtain the desired product from the starting materials, or feedstocks.
Chemical Engineering[edit]
Chemical engineering unit operations consist of five classes:
- Fluid flow processes, including fluids transportation, filtration, and solids fluidization.
- Heat transfer processes, including evaporation and heat exchange.
- Mass transfer processes, including gas absorption, distillation, extraction, adsorption, and drying.
- Thermodynamic processes, including gas liquefaction, and refrigeration.
- Mechanical processes, including solids transportation, crushing and pulverization, and screening and sieving.
Chemical engineering unit operations also fall in the following categories which involve elements from more than one class:
- Combination (mixing)
- Separation (distillation, crystallization)
- Reaction (chemical reaction)
Furthermore, there are some unit operations which combine even these categories, such as reactive distillation and stirred tank reactors. A "pure" unit operation is a physical transport process, while a mixed chemical/physical process requires modeling both the physical transport, such as diffusion, and the chemical reaction. This is usually necessary for designing catalytic reactions, and is considered a separate discipline, termed chemical reaction engineering.
Chemical engineering unit operations and chemical engineering unit processing form the main principles of all kinds of chemical industries and are the foundation of designs of chemical plants, factories, and equipment used.
https://en.wikipedia.org/wiki/Unit_operation
In physics, transport phenomena are all irreversible processes of statistical nature stemming from the random continuous motion of molecules, mostly observed in fluids. Every aspect of transport phenomena is grounded in two primary concepts : the conservation laws, and the constitutive equations. The conservation laws, which in the context of transport phenomena are formulated as continuity equations, describe how the quantity being studied must be conserved. The constitutive equations describe how the quantity in question responds to various stimuli via transport. Prominent examples include Fourier's law of heat conduction and the Navier–Stokes equations, which describe, respectively, the response of heat flux to temperature gradients and the relationship between fluid flux and the forces applied to the fluid. These equations also demonstrate the deep connection between transport phenomena and thermodynamics, a connection that explains why transport phenomena are irreversible. Almost all of these physical phenomena ultimately involve systems seeking their lowest energy state in keeping with the principle of minimum energy. As they approach this state, they tend to achieve true thermodynamic equilibrium, at which point there are no longer any driving forces in the system and transport ceases. The various aspects of such equilibrium are directly connected to a specific transport: heat transfer is the system's attempt to achieve thermal equilibrium with its environment, just as mass and momentum transport move the system towards chemical and mechanical equilibrium.
Examples of transport processes include heat conduction (energy transfer), fluid flow (momentum transfer), molecular diffusion (mass transfer), radiation and electric charge transfer in semiconductors.[3][4][5][6]
Transport phenomena have wide application. For example, in solid state physics, the motion and interaction of electrons, holes and phonons are studied under "transport phenomena". Another example is in biomedical engineering, where some transport phenomena of interest are thermoregulation, perfusion, and microfluidics. In chemical engineering, transport phenomena are studied in reactor design, analysis of molecular or diffusive transport mechanisms, and metallurgy.
The transport of mass, energy, and momentum can be affected by the presence of external sources:
- An odor dissipates more slowly (and may intensify) when the source of the odor remains present.
- The rate of cooling of a solid that is conducting heat depends on whether a heat source is applied.
- The gravitational force acting on a rain drop counteracts the resistance or drag imparted by the surrounding air.
Diffusion[edit]
There are some notable similarities in equations for momentum, energy, and mass transfer[7] which can all be transported by diffusion, as illustrated by the following examples:
- Mass: the spreading and dissipation of odors in air is an example of mass diffusion.
- Energy: the conduction of heat in a solid material is an example of heat diffusion.
- Momentum: the drag experienced by a rain drop as it falls in the atmosphere is an example of momentum diffusion (the rain drop loses momentum to the surrounding air through viscous stresses and decelerates).
The molecular transfer equations of Newton's law for fluid momentum, Fourier's law for heat, and Fick's law for mass are very similar. One can convert from one transport coefficient to another in order to compare all three different transport phenomena.[8]
| Transported quantity | Physical phenomenon | Equation |
|---|---|---|
| Momentum | Viscosity (Newtonian fluid) | |
| Energy | Heat conduction (Fourier's law) | |
| Mass | Molecular diffusion (Fick's law) |
(Definitions of these formulas are given below).
A great deal of effort has been devoted in the literature to developing analogies among these three transport processes for turbulent transfer so as to allow prediction of one from any of the others. The Reynolds analogy assumes that the turbulent diffusivities are all equal and that the molecular diffusivities of momentum (μ/ρ) and mass (DAB) are negligible compared to the turbulent diffusivities. When liquids are present and/or drag is present, the analogy is not valid. Other analogies, such as von Karman's and Prandtl's, usually result in poor relations.
The most successful and most widely used analogy is the Chilton and Colburn J-factor analogy.[9] This analogy is based on experimental data for gases and liquids in both the laminar and turbulent regimes. Although it is based on experimental data, it can be shown to satisfy the exact solution derived from laminar flow over a flat plate. All of this information is used to predict transfer of mass.
Onsager reciprocal relations[edit]
In fluid systems described in terms of temperature, matter density, and pressure, it is known that temperature differences lead to heat flows from the warmer to the colder parts of the system; similarly, pressure differences will lead to matter flow from high-pressure to low-pressure regions (a "reciprocal relation"). What is remarkable is the observation that, when both pressure and temperature vary, temperature differences at constant pressure can cause matter flow (as in convection) and pressure differences at constant temperature can cause heat flow. Perhaps surprisingly, the heat flow per unit of pressure difference and the density (matter) flow per unit of temperature difference are equal.
This equality was shown to be necessary by Lars Onsager using statistical mechanics as a consequence of the time reversibility of microscopic dynamics. The theory developed by Onsager is much more general than this example and capable of treating more than two thermodynamic forces at once.[10]
Momentum transfer[edit]
In momentum transfer, the fluid is treated as a continuous distribution of matter. The study of momentum transfer, or fluid mechanics can be divided into two branches: fluid statics (fluids at rest), and fluid dynamics (fluids in motion). When a fluid is flowing in the x-direction parallel to a solid surface, the fluid has x-directed momentum, and its concentration is υxρ. By random diffusion of molecules there is an exchange of molecules in the z-direction. Hence the x-directed momentum has been transferred in the z-direction from the faster- to the slower-moving layer. The equation for momentum transfer is Newton's law of viscosity written as follows:
where τzx is the flux of x-directed momentum in the z-direction, ν is μ/ρ, the momentum diffusivity, z is the distance of transport or diffusion, ρ is the density, and μ is the dynamic viscosity. Newton's law of viscosity is the simplest relationship between the flux of momentum and the velocity gradient.
Mass transfer[edit]
When a system contains two or more components whose concentration vary from point to point, there is a natural tendency for mass to be transferred, minimizing any concentration difference within the system. Mass Transfer in a system is governed by Fick's First Law: 'Diffusion flux from higher concentration to lower concentration is proportional to the gradient of the concentration of the substance and the diffusivity of the substance in the medium.' Mass transfer can take place due to different driving forces. Some of them are:[11]
- Mass can be transferred by the action of a pressure gradient (pressure diffusion)
- Forced diffusion occurs because of the action of some external force
- Diffusion can be caused by temperature gradients (thermal diffusion)
- Diffusion can be caused by differences in chemical potential
This can be compared to Fick's Law of Diffusion, for a species A in a binary mixture consisting of A and B:
where D is the diffusivity constant.
Energy transfer[edit]
All processes in engineering involve the transfer of energy. Some examples are the heating and cooling of process streams, phase changes, distillations, etc. The basic principle is the first law of thermodynamics which is expressed as follows for a static system:
The net flux of energy through a system equals the conductivity times the rate of change of temperature with respect to position.
For other systems that involve either turbulent flow, complex geometries or difficult boundary conditions another equation would be easier to use:
where A is the surface area, : is the temperature driving force, Q is the heat flow per unit time, and h is the heat transfer coefficient.
Within heat transfer, two types of convection can occur:
- Forced convection can occur in both laminar and turbulent flow. In the situation of laminar flow in circular tubes, several dimensionless numbers are used such as Nusselt number, Reynolds number, and Prandtl number. The commonly used equation is .
- Natural or free convection is a function of Grashof and Prandtl numbers. The complexities of free convection heat transfer make it necessary to mainly use empirical relations from experimental data.[11]
Heat transfer is analyzed in packed beds, nuclear reactors and heat exchangers.
Applications[edit]
Pollution[edit]
The study of transport processes is relevant for understanding the release and distribution of pollutants into the environment. In particular, accurate modeling can inform mitigation strategies. Examples include the control of surface water pollution from urban runoff, and policies intended to reduce the copper content of vehicle brake pads in the U.S.[12][13]
See also[edit]
https://en.wikipedia.org/wiki/Reversible_process_(thermodynamics)
https://en.wikipedia.org/wiki/Trihydrogen_cation
https://en.wikipedia.org/wiki/Hydride
https://en.wikipedia.org/wiki/Positronium_hydride
https://en.wikipedia.org/wiki/Exotic_atom#exotic_molecules
https://en.wikipedia.org/wiki/Isotopologue
https://en.wikipedia.org/wiki/Ionosphere
https://en.wikipedia.org/wiki/Triatomic_molecule
https://en.wikipedia.org/wiki/Three-center_two-electron_bond
https://en.wikipedia.org/wiki/Polyhedral_skeletal_electron_pair_theory
https://en.wikipedia.org/wiki/Isotopomers
https://en.wikipedia.org/wiki/Pseudohalogen
https://en.wikipedia.org/wiki/Nuclear_transmutation
https://en.wikipedia.org/wiki/Dark_matter
https://en.wikipedia.org/wiki/Lambda-CDM_model
https://en.wikipedia.org/wiki/Dark_energy
https://en.wikipedia.org/wiki/Dark_fluid
https://en.wikipedia.org/wiki/Cosmic_microwave_background
https://en.wikipedia.org/wiki/Cosmic_neutrino_background
https://en.wikipedia.org/wiki/Annihilation
https://en.wikipedia.org/wiki/Nuclear_fission
https://en.wikipedia.org/wiki/Exponential_decay
https://en.wikipedia.org/wiki/Weak_interaction
https://en.wikipedia.org/wiki/Vacuum
https://en.wikipedia.org/wiki/Mirror_matter
https://en.wikipedia.org/wiki/Fusion_power#Magnetic_Mirror
https://en.wikipedia.org/wiki/Fictitious_force
Outer space is an even higher-quality vacuum, with the equivalent of just a few hydrogen atoms per cubic meter on average in intergalactic space.[5]
https://en.wikipedia.org/wiki/Vacuum
https://en.wikipedia.org/wiki/Magnetohydrodynamics
Nuclides with even numbers of both have a total spin of zero and are therefore NMR-inactive.
https://en.wikipedia.org/wiki/Nuclear_magnetic_resonance
https://en.wikipedia.org/wiki/Degaussing
https://en.wikipedia.org/wiki/Ferromagnetism
https://en.wikipedia.org/wiki/Magnetic_field
https://en.wikipedia.org/wiki/Nuclear_magnetic_resonance
https://en.wikipedia.org/wiki/Angular_momentum
https://en.wikipedia.org/wiki/Nuclide
https://en.wikipedia.org/wiki/Larmor_precession
https://en.wikipedia.org/wiki/Magnetic_coupling
https://en.wikipedia.org/wiki/Magnetic_hysteresis
https://en.wikipedia.org/wiki/Magnetic_flux
https://en.wikipedia.org/wiki/Magnetized_target_fusion
https://en.wikipedia.org/wiki/Heat_exchanger
https://en.wikipedia.org/wiki/Drag_(physics)
https://en.wikipedia.org/wiki/Electrodynamic_suspension
https://en.wikipedia.org/wiki/Magnetic_levitation
https://en.wikipedia.org/wiki/Lenz%27s_law
https://en.wikipedia.org/wiki/Ampère%27s_force_law
https://en.wikipedia.org/wiki/Earnshaw%27s_theorem#Proofs_for_magnetic_dipoles
https://en.wikipedia.org/wiki/Gyroscope
https://en.wikipedia.org/wiki/Linear_induction_motor
https://en.wikipedia.org/wiki/Pyrolytic_carbon
https://en.wikipedia.org/wiki/Bismuth
https://en.wikipedia.org/wiki/Vacuum_permeability
https://en.wikipedia.org/wiki/Electrostatic_levitation
https://en.wikipedia.org/wiki/Magnetic_levitation
https://en.wikipedia.org/wiki/Magnetic_pressure
https://en.wikipedia.org/wiki/Dipole_magnet
https://en.wikipedia.org/wiki/Permeability_(electromagnetism)
https://en.wikipedia.org/wiki/Nonlinear_optics
https://en.wikipedia.org/wiki/Heat_exchanger
https://en.wikipedia.org/wiki/Drag_(physics)
https://en.wikipedia.org/wiki/Electrodynamic_suspension
https://en.wikipedia.org/wiki/Magnetic_levitation
https://en.wikipedia.org/wiki/Field-reversed_configuration
https://en.wikipedia.org/wiki/Superconductivity
https://en.wikipedia.org/wiki/Diol_cyclization
cyclicization ringulants
https://en.wikipedia.org/wiki/Magnetic_dipole–dipole_interaction
https://en.wikipedia.org/wiki/J-coupling
https://en.wikipedia.org/wiki/Electron_electric_dipole_moment
https://en.wikipedia.org/wiki/Zero-point_energy
https://en.wikipedia.org/wiki/Van_der_Waals_force
https://en.wikipedia.org/wiki/Zero_field_splitting
https://en.wikipedia.org/wiki/Quadrupole
https://en.wikipedia.org/wiki/Quadrupole#Gravitational_quadrupole
https://en.wikipedia.org/wiki/Trace_(linear_algebra)
https://en.wikipedia.org/wiki/Overtone_band
https://en.wikipedia.org/wiki/Lockheed_Martin_Compact_Fusion_Reactor
https://en.wikipedia.org/wiki/Deuterium–tritium_fusion
https://en.wikipedia.org/wiki/Magnetized_target_fusion
https://en.wikipedia.org/wiki/Magnetic_levitation
https://en.wikipedia.org/wiki/Dimensionless_quantity
https://en.wikipedia.org/wiki/Linear_stability
https://en.wikipedia.org/wiki/Heat_exchanger
https://en.wikipedia.org/wiki/Field-reversed_configuration
https://en.wikipedia.org/wiki/Superconductivity
https://en.wikipedia.org/wiki/Magneto-inertial_fusion
https://en.wikipedia.org/wiki/Neutronium
https://en.wikipedia.org/wiki/Preon
https://en.wikipedia.org/wiki/Meson_bomb
https://en.wikipedia.org/wiki/Pressuron
https://en.wikipedia.org/wiki/Vertical_pressure_variation
Magnetized Liner Inertial Fusion (MagLIF) is an emerging method of producing controlled nuclear fusion. It is part of the broad category of inertial fusion energy (IFE) systems, which drives the inward movement of fusion fuel, thereby compressing it to reach densities and temperatures where fusion reactions occur. Previous IFE experiments used laser drivers to reach these conditions, whereas MagLIF uses a combination of lasers for heating and Z-pinch for compression. A variety of theoretical considerations suggest such a system will reach the required conditions for fusion with a machine of significantly less complexity than the pure-laser approach. There are currently at least two facilities testing feasibility of the MagLIF concept, the Z-machine at Sandia Labs in the US and Primary Test Stand (PTS) located in Mianyang, China.[1]
Description[edit]
MagLIF is a method of generating energy by using a 100 nanosecond pulse of electricity to create an intense Z-pinch magnetic field that inwardly crushes a fuel filled cylindrical metal liner (a hohlraum) through which the electric pulse runs. Just before the cylinder implodes, a laser is used to preheat fusion fuel (such as deuterium-tritium) that is held within the cylinder and contained by a magnetic field. Sandia National Labs is currently exploring the potential for this method to generate energy by utilizing the Z machine.
MagLIF has characteristics of both Inertial confinement fusion (due to the usage of a laser and pulsed compression) and magnetic confinement (due to the utilization of a powerful magnetic field to inhibit thermal conduction and contain the plasma). In results published in 2012, a LASNEX based computer simulation of a 70 megaampere facility showed the prospect of a spectacular energy return of 1000 times the expended energy. A 60 MA facility would produce a 100x yield. The currently available facility at Sandia, Z machine, is capable of 27 MA and may be capable of producing slightly more than breakeven energy while helping to validate the computer simulations.[2] The Z-machine conducted MagLIF experiments in November 2013 with a view towards breakeven experiments using D-T fuel in 2018.[3]
Sandia Labs planned to proceed to ignition experiments after establishing the following:[4]
- That the liner will not break apart too quickly under the intense energy. This has been apparently confirmed by recent experiments. This hurdle was the biggest concern regarding MagLIF following its initial proposal.
- That laser preheating is able to correctly heat the fuel — to be confirmed by experiments starting in December 2012.
- That magnetic fields generated by a pair of coils above and below the hohlraum can serve to trap the preheated fusion fuel and importantly inhibit thermal conduction without causing the target to buckle prematurely. — to be confirmed by experiments starting in December 2012.
Following these experiments, an integrated test started in November 2013. The test yielded about 1010 high-energy neutrons.
As of November 2013, the facility at Sandia labs had the following capabilities:[3][5]
- 10 tesla magnetic field
- 2 kJ laser
- 16 MA
- D-D fuel
In 2014, the test yielded up to 2×1012 D-D neutrons under the following conditions:[6]
- 10 tesla magnetic field
- 2.5 kJ laser
- 19 MA
- D-D fuel
Experiments aiming for energy breakeven with D-T fuel were expected to occur in 2018.[7]
To achieve scientific breakeven, the facility is going through a 5-year upgrade to:
- 30 teslas
- 8 kJ laser
- 27 MA
- D-T fuel handling[3]
In 2019, after encountering significant problems related to mixing of imploding foil with fuel and helical instability of plasma,[8] the tests yielded up to 3.2×1012 neutrons under the following conditions:[9]
- 1.2 kJ laser
- 18 MA
In 2020, "the burn-averaged ion temperature doubled to 3.1 keV and the primary deuterium-deuterium neutron yield increased by more than an order of magnitude to 1.1 × 10^13 (2 kJ deuterium-tritium equivalent) through a simultaneous increase in the applied magnetic field (from 10.4 to 15.9 T), laser preheat energy (from 0.46 to 1.2 kJ), and current coupling (from 16 to 20 MA). " [10]
See also[edit]
Ni
, 58
Fe
, 56
Fe
, and 60
Ni
.[11] Even though the nickel isotope, 62
Ni
, is more stable, the iron isotope 56
Fe
is an order of magnitude more common. This is due to the fact that there is no easy way for stars to create 62
Ni
through the alpha process.
Other principles[edit]
Some other confinement principles have been investigated.
- Antimatter-initialized fusion uses small amounts of antimatter to trigger a tiny fusion explosion. This has been studied primarily in the context of making nuclear pulse propulsion, and pure fusion bombs feasible. This is not near becoming a practical power source, due to the cost of manufacturing antimatter alone.
- Pyroelectric fusion was reported in April 2005 by a team at UCLA. The scientists used a pyroelectric crystal heated from −34 to 7 °C (−29 to 45 °F), combined with a tungsten needle to produce an electric field of about 25 gigavolts per meter to ionize and accelerate deuterium nuclei into an erbium deuteride target. At the estimated energy levels,[22] the D-D fusion reaction may occur, producing helium-3 and a 2.45 MeV neutron. Although it makes a useful neutron generator, the apparatus is not intended for power generation since it requires far more energy than it produces.[23][24][25][26]
- Hybrid nuclear fusion-fission (hybrid nuclear power) is a proposed means of generating power by use of a combination of nuclear fusion and fission processes. The concept dates to the 1950s, and was briefly advocated by Hans Bethe during the 1970s, but largely remained unexplored until a revival of interest in 2009, due to the delays in the realization of pure fusion.[27]
- Project PACER, carried out at Los Alamos National Laboratory (LANL) in the mid-1970s, explored the possibility of a fusion power system that would involve exploding small hydrogen bombs (fusion bombs) inside an underground cavity. As an energy source, the system is the only fusion power system that could be demonstrated to work using existing technology. However it would also require a large, continuous supply of nuclear bombs, making the economics of such a system rather questionable.
- Bubble fusion also called sonofusion was a proposed mechanism for achieving fusion via sonic cavitation which rose to prominence in the early 2000s. Subsequent attempts at replication failed and the principal investigator, Rusi Taleyarkhan, was judged guilty of research misconduct in 2008.[28]
Few reactions meet these criteria. The following are those with the largest cross sections:[30] [31]
(1) 2
1D
+ 3
1T
→ 4
2He
( 3.52 MeV ) + n0 ( 14.06 MeV ) (2i) 2
1D
+ 2
1D
→ 3
1T
( 1.01 MeV ) + p+ ( 3.02 MeV ) 50% (2ii) → 3
2He
( 0.82 MeV ) + n0 ( 2.45 MeV ) 50% (3) 2
1D
+ 3
2He
→ 4
2He
( 3.6 MeV ) + p+ ( 14.7 MeV ) (4) 3
1T
+ 3
1T
→ 4
2He
+ 2 n0 + 11.3 MeV (5) 3
2He
+ 3
2He
→ 4
2He
+ 2 p+ + 12.9 MeV (6i) 3
2He
+ 3
1T
→ 4
2He
+ p+ + n0 + 12.1 MeV 57% (6ii) → 4
2He
( 4.8 MeV ) + 2
1D
( 9.5 MeV ) 43% (7i) 2
1D
+ 6
3Li
→ 2 4
2He
+ 22.4 MeV (7ii) → 3
2He
+ 4
2He
+ n0 + 2.56 MeV (7iii) → 7
3Li
+ p+ + 5.0 MeV (7iv) → 7
4Be
+ n0 + 3.4 MeV (8) p+ + 6
3Li
→ 4
2He
( 1.7 MeV ) + 3
2He
( 2.3 MeV ) (9) 3
2He
+ 6
3Li
→ 2 4
2He
+ p+ + 16.9 MeV (10) p+ + 11
5B
→ 3 4
2He
+ 8.7 MeV
| Nucleosynthesis |
|---|
 |
| Related topics |
For reactions with two products, the energy is divided between them in inverse proportion to their masses, as shown. In most reactions with three products, the distribution of energy varies. For reactions that can result in more than one set of products, the branching ratios are given.
Some reaction candidates can be eliminated at once. The D-6Li reaction has no advantage compared to p+-11
5B
because it is roughly as difficult to burn but produces substantially more neutrons through 2
1D
-2
1D
side reactions. There is also a p+-7
3Li
reaction, but the cross section is far too low, except possibly when Ti > 1 MeV, but at such high temperatures an endothermic, direct neutron-producing reaction also becomes very significant. Finally there is also a p+-9
4Be
reaction, which is not only difficult to burn, but 9
4Be
can be easily induced to split into two alpha particles and a neutron.
In addition to the fusion reactions, the following reactions with neutrons are important in order to "breed" tritium in "dry" fusion bombs and some proposed fusion reactors:
The latter of the two equations was unknown when the U.S. conducted the Castle Bravo fusion bomb test in 1954. Being just the second fusion bomb ever tested (and the first to use lithium), the designers of the Castle Bravo "Shrimp" had understood the usefulness of 6Li in tritium production, but had failed to recognize that 7Li fission would greatly increase the yield of the bomb. While 7Li has a small neutron cross-section for low neutron energies, it has a higher cross section above 5 MeV.[32] The 15 Mt yield was 150% greater than the predicted 6 Mt and caused unexpected exposure to fallout.
To evaluate the usefulness of these reactions, in addition to the reactants, the products, and the energy released, one needs to know something about the nuclear cross section. Any given fusion device has a maximum plasma pressure it can sustain, and an economical device would always operate near this maximum. Given this pressure, the largest fusion output is obtained when the temperature is chosen so that <σv>/T2 is a maximum. This is also the temperature at which the value of the triple product nTτ required for ignition is a minimum, since that required value is inversely proportional to <σv>/T2 (see Lawson criterion). (A plasma is "ignited" if the fusion reactions produce enough power to maintain the temperature without external heating.) This optimum temperature and the value of <σv>/T2 at that temperature is given for a few of these reactions in the following table.
| fuel | T [keV] | <σv>/T2 [m3/s/keV2] |
|---|---|---|
| 2 1D -3 1T | 13.6 | 1.24×10−24 |
| 2 1D -2 1D | 15 | 1.28×10−26 |
| 2 1D -3 2He | 58 | 2.24×10−26 |
| p+-6 3Li | 66 | 1.46×10−27 |
| p+-11 5B | 123 | 3.01×10−27 |
Note that many of the reactions form chains. For instance, a reactor fueled with 3
1T
and 3
2He
creates some 2
1D
, which is then possible to use in the 2
1D
-3
2He
reaction if the energies are "right". An elegant idea is to combine the reactions (8) and (9). The 3
2He
from reaction (8) can react with 6
3Li
in reaction (9) before completely thermalizing. This produces an energetic proton, which in turn undergoes reaction (8) before thermalizing. Detailed analysis shows that this idea would not work well,[citation needed] but it is a good example of a case where the usual assumption of a Maxwellian plasma is not appropriate.
Neutronicity, confinement requirement, and power density[edit]
Any of the reactions above can in principle be the basis of fusion power production. In addition to the temperature and cross section discussed above, we must consider the total energy of the fusion products Efus, the energy of the charged fusion products Ech, and the atomic number Z of the non-hydrogenic reactant.
Specification of the 2
1D
-2
1D
reaction entails some difficulties, though. To begin with, one must average over the two branches (2i) and (2ii). More difficult is to decide how to treat the 3
1T
and 3
2He
products. 3
1T
burns so well in a deuterium plasma that it is almost impossible to extract from the plasma. The 2
1D
-3
2He
reaction is optimized at a much higher temperature, so the burnup at the optimum 2
1D
-2
1D
temperature may be low. Therefore, it seems reasonable to assume the 3
1T
but not the 3
2He
gets burned up and adds its energy to the net reaction, which means the total reaction would be the sum of (2i), (2ii), and (1):
For calculating the power of a reactor (in which the reaction rate is determined by the D-D step), we count the 2
1D
-2
1D
fusion energy per D-D reaction as Efus = (4.03 MeV + 17.6 MeV)×50% + (3.27 MeV)×50% = 12.5 MeV and the energy in charged particles as Ech = (4.03 MeV + 3.5 MeV)×50% + (0.82 MeV)×50% = 4.2 MeV. (Note: if the tritium ion reacts with a deuteron while it still has a large kinetic energy, then the kinetic energy of the helium-4 produced may be quite different from 3.5 MeV,[33] so this calculation of energy in charged particles is only an approximation of the average.) The amount of energy per deuteron consumed is 2/5 of this, or 5.0 MeV (a specific energy of about 225 million MJ per kilogram of deuterium).
Another unique aspect of the 2
1D
-2
1D
reaction is that there is only one reactant, which must be taken into account when calculating the reaction rate.
With this choice, we tabulate parameters for four of the most important reactions
| fuel | Z | Efus [MeV] | Ech [MeV] | neutronicity |
|---|---|---|---|---|
| 2 1D -3 1T | 1 | 17.6 | 3.5 | 0.80 |
| 2 1D -2 1D | 1 | 12.5 | 4.2 | 0.66 |
| 2 1D -3 2He | 2 | 18.3 | 18.3 | ≈0.05 |
| p+-11 5B | 5 | 8.7 | 8.7 | ≈0.001 |
The last column is the neutronicity of the reaction, the fraction of the fusion energy released as neutrons. This is an important indicator of the magnitude of the problems associated with neutrons like radiation damage, biological shielding, remote handling, and safety. For the first two reactions it is calculated as (Efus-Ech)/Efus. For the last two reactions, where this calculation would give zero, the values quoted are rough estimates based on side reactions that produce neutrons in a plasma in thermal equilibrium.
Of course, the reactants should also be mixed in the optimal proportions. This is the case when each reactant ion plus its associated electrons accounts for half the pressure. Assuming that the total pressure is fixed, this means that particle density of the non-hydrogenic ion is smaller than that of the hydrogenic ion by a factor 2/(Z+1). Therefore, the rate for these reactions is reduced by the same factor, on top of any differences in the values of <σv>/T2. On the other hand, because the 2
1D
-2
1D
reaction has only one reactant, its rate is twice as high as when the fuel is divided between two different hydrogenic species, thus creating a more efficient reaction.
Thus there is a "penalty" of (2/(Z+1)) for non-hydrogenic fuels arising from the fact that they require more electrons, which take up pressure without participating in the fusion reaction. (It is usually a good assumption that the electron temperature will be nearly equal to the ion temperature. Some authors, however, discuss the possibility that the electrons could be maintained substantially colder than the ions. In such a case, known as a "hot ion mode", the "penalty" would not apply.) There is at the same time a "bonus" of a factor 2 for 2
1D
-2
1D
because each ion can react with any of the other ions, not just a fraction of them.
We can now compare these reactions in the following table.
| fuel | <σv>/T2 | penalty/bonus | inverse reactivity | Lawson criterion | power density (W/m3/kPa2) | inverse ratio of power density |
|---|---|---|---|---|---|---|
| 2 1D -3 1T | 1.24×10−24 | 1 | 1 | 1 | 34 | 1 |
| 2 1D -2 1D | 1.28×10−26 | 2 | 48 | 30 | 0.5 | 68 |
| 2 1D -3 2He | 2.24×10−26 | 2/3 | 83 | 16 | 0.43 | 80 |
| p+-6 3Li | 1.46×10−27 | 1/2 | 1700 | 0.005 | 6800 | |
| p+-11 5B | 3.01×10−27 | 1/3 | 1240 | 500 | 0.014 | 2500 |
The maximum value of <σv>/T2 is taken from a previous table. The "penalty/bonus" factor is that related to a non-hydrogenic reactant or a single-species reaction. The values in the column "inverse reactivity" are found by dividing 1.24×10−24 by the product of the second and third columns. It indicates the factor by which the other reactions occur more slowly than the 2
1D
-3
1T
reaction under comparable conditions. The column "Lawson criterion" weights these results with Ech and gives an indication of how much more difficult it is to achieve ignition with these reactions, relative to the difficulty for the 2
1D
-3
1T
reaction. The next-to-last column is labeled "power density" and weights the practical reactivity by Efus. The final column indicates how much lower the fusion power density of the other reactions is compared to the 2
1D
-3
1T
reaction and can be considered a measure of the economic potential.
Bremsstrahlung losses in quasineutral, isotropic plasmas[edit]
The ions undergoing fusion in many systems will essentially never occur alone but will be mixed with electrons that in aggregate neutralize the ions' bulk electrical charge and form a plasma. The electrons will generally have a temperature comparable to or greater than that of the ions, so they will collide with the ions and emit x-ray radiation of 10–30 keV energy, a process known as Bremsstrahlung.
The huge size of the Sun and stars means that the x-rays produced in this process will not escape and will deposit their energy back into the plasma. They are said to be opaque to x-rays. But any terrestrial fusion reactor will be optically thin for x-rays of this energy range. X-rays are difficult to reflect but they are effectively absorbed (and converted into heat) in less than mm thickness of stainless steel (which is part of a reactor's shield). This means the bremsstrahlung process is carrying energy out of the plasma, cooling it.
The ratio of fusion power produced to x-ray radiation lost to walls is an important figure of merit. This ratio is generally maximized at a much higher temperature than that which maximizes the power density (see the previous subsection). The following table shows estimates of the optimum temperature and the power ratio at that temperature for several reactions:
| fuel | Ti (keV) | Pfusion/PBremsstrahlung |
|---|---|---|
| 2 1D -3 1T | 50 | 140 |
| 2 1D -2 1D | 500 | 2.9 |
| 2 1D -3 2He | 100 | 5.3 |
| 3 2He -3 2He | 1000 | 0.72 |
| p+-6 3Li | 800 | 0.21 |
| p+-11 5B | 300 | 0.57 |
The actual ratios of fusion to Bremsstrahlung power will likely be significantly lower for several reasons. For one, the calculation assumes that the energy of the fusion products is transmitted completely to the fuel ions, which then lose energy to the electrons by collisions, which in turn lose energy by Bremsstrahlung. However, because the fusion products move much faster than the fuel ions, they will give up a significant fraction of their energy directly to the electrons. Secondly, the ions in the plasma are assumed to be purely fuel ions. In practice, there will be a significant proportion of impurity ions, which will then lower the ratio. In particular, the fusion products themselves must remain in the plasma until they have given up their energy, and will remain for some time after that in any proposed confinement scheme. Finally, all channels of energy loss other than Bremsstrahlung have been neglected. The last two factors are related. On theoretical and experimental grounds, particle and energy confinement seem to be closely related. In a confinement scheme that does a good job of retaining energy, fusion products will build up. If the fusion products are efficiently ejected, then energy confinement will be poor, too.
The temperatures maximizing the fusion power compared to the Bremsstrahlung are in every case higher than the temperature that maximizes the power density and minimizes the required value of the fusion triple product. This will not change the optimum operating point for 2
1D
-3
1T
very much because the Bremsstrahlung fraction is low, but it will push the other fuels into regimes where the power density relative to 2
1D
-3
1T
is even lower and the required confinement even more difficult to achieve. For 2
1D
-2
1D
and 2
1D
-3
2He
, Bremsstrahlung losses will be a serious, possibly prohibitive problem. For 3
2He
-3
2He
, p+-6
3Li
and p+-11
5B
the Bremsstrahlung losses appear to make a fusion reactor using these fuels with a quasineutral, isotropic plasma impossible. Some ways out of this dilemma have been considered but rejected.[34][35] This limitation does not apply to non-neutral and anisotropic plasmas; however, these have their own challenges to contend with.
Mathematical description of cross section[edit]
Fusion under classical physics[edit]
In a classical picture, nuclei can be understood as hard spheres that repel each other through the Coulomb force but fuse once the two spheres come close enough for contact. Estimating the radius of an atomic nuclei as about one femtometer, the energy needed for fusion of two hydrogen is:
This would imply that for the core of the sun, which has a Boltzmann distribution with a temperature of around 1.4 keV, the probability hydrogen would reach the threshold is , that is, fusion would never occur. However, fusion in the sun does occur due to quantum mechanics.
Parameterization of cross section[edit]
The probability that fusion occurs is greatly increased compared to the classical picture, thanks to the smearing of the effective radius as the DeBroglie wavelength as well as quantum tunnelling through the potential barrier. To determine the rate of fusion reactions, the value of most interest is the cross section, which describes the probability that particles will fuse by giving a characteristic area of interaction. An estimation of the fusion cross-sectional area is often broken into three pieces:
Where is the geometric cross section, T is the barrier transparency and R is the reaction characteristics of the reaction.
is of the order of the square of the de-Broglie wavelength where is the reduced mass of the system and is the center of mass energy of the system.
T can be approximated by the Gamow transparency, which has the form: where is the Gamow factor and comes from estimating the quantum tunneling probability through the potential barrier.
R contains all the nuclear physics of the specific reaction and takes very different values depending on the nature of the interaction. However, for most reactions, the variation of is small compared to the variation from the Gamow factor and so is approximated by a function called the Astrophysical S-factor, , which is weakly varying in energy. Putting these dependencies together, one approximation for the fusion cross section as a function of energy takes the form:
More detailed forms of the cross-section can be derived through nuclear physics-based models and R-matrix theory.
In mathematics, the gravitational potential is also known as the Newtonian potential and is fundamental in the study of potential theory. It may also be used for solving the electrostatic and magnetostatic fields generated by uniformly charged or polarized ellipsoidal bodies.[1]
Plot of a two-dimensional slice of the gravitational potential in and around a uniform spherical body. The inflection points of the cross-section are at the surface of the body.https://en.wikipedia.org/wiki/Gravitational_potential
The axion (/ˈæksiɒn/) is a hypothetical elementary particle postulated by the Peccei–Quinn theory in 1977 to resolve the strong CP problem in quantum chromodynamics (QCD). If axions exist and have low mass within a specific range, they are of interest as a possible component of cold dark matter.
https://en.wikipedia.org/wiki/Axion
Because dark matter has not yet been observed directly, if it exists, it must barely interact with ordinary baryonic matter and radiation, except through gravity. Most dark matter is thought to be non-baryonic in nature; it may be composed of some as-yet undiscovered subatomic particles.[b] The primary candidate for dark matter is some new kind of elementary particle that has not yet been discovered, in particular, weakly interacting massive particles(WIMPs).[14] Many experiments to directly detect and study dark matter particles are being actively undertaken, but none have yet succeeded.[15] Dark matter is classified as "cold", "warm", or "hot" according to its velocity (more precisely, its free streaming length). Current models favor a cold dark matterscenario, in which structures emerge by gradual accumulation of particles.
https://en.wikipedia.org/wiki/Dark_matter
The axion (/ˈæksiɒn/) is a hypothetical elementary particle postulated by the Peccei–Quinn theory in 1977 to resolve the strong CP problem in quantum chromodynamics (QCD). If axions exist and have low mass within a specific range, they are of interest as a possible component of cold dark matter.
https://en.wikipedia.org/wiki/Axion
Because dark matter has not yet been observed directly, if it exists, it must barely interact with ordinary baryonic matter and radiation, except through gravity. Most dark matter is thought to be non-baryonic in nature; it may be composed of some as-yet undiscovered subatomic particles.[b] The primary candidate for dark matter is some new kind of elementary particle that has not yet been discovered, in particular, weakly interacting massive particles(WIMPs).[14] Many experiments to directly detect and study dark matter particles are being actively undertaken, but none have yet succeeded.[15] Dark matter is classified as "cold", "warm", or "hot" according to its velocity (more precisely, its free streaming length). Current models favor a cold dark matterscenario, in which structures emerge by gradual accumulation of particles.
https://en.wikipedia.org/wiki/Dark_matter
Small molecule hyperfine structure[edit]
A typical simple example of the hyperfine structure due to the interactions discussed above is in the rotational transitions of hydrogen cyanide (1H12C14N) in its ground vibrational state. Here, the electric quadrupole interaction is due to the 14N-nucleus, the hyperfine nuclear spin-spin splitting is from the magnetic coupling between nitrogen, 14N (IN = 1), and hydrogen, 1H (IH = 1⁄2), and a hydrogen spin-rotation interaction due to the 1H-nucleus. These contributing interactions to the hyperfine structure in the molecule are listed here in descending order of influence. Sub-doppler techniques have been used to discern the hyperfine structure in HCN rotational transitions.[11]
The dipole selection rules for HCN hyperfine structure transitions are , , where J is the rotational quantum number and F is the total rotational quantum number inclusive of nuclear spin (), respectively. The lowest transition () splits into a hyperfine triplet. Using the selection rules, the hyperfine pattern of transition and higher dipole transitions is in the form of a hyperfine sextet. However, one of these components () carries only 0.6% of the rotational transition intensity in the case of . This contribution drops for increasing J. So, from upwards the hyperfine pattern consists of three very closely spaced stronger hyperfine components (, ) together with two widely spaced components; one on the low frequency side and one on the high frequency side relative to the central hyperfine triplet. Each of these outliers carry ~ (J is the upper rotational quantum number of the allowed dipole transition) the intensity of the entire transition. For consecutively higher-J transitions, there are small but significant changes in the relative intensities and positions of each individual hyperfine component.[12]
Measurements[edit]
Hyperfine interactions can be measured, among other ways, in atomic and molecular spectra and in electron paramagnetic resonance spectra of free radicals and transition-metal ions.
Applications[edit]
Astrophysics[edit]
As the hyperfine splitting is very small, the transition frequencies are usually not located in the optical, but are in the range of radio- or microwave (also called sub-millimeter) frequencies.
Hyperfine structure gives the 21 cm line observed in H I regions in interstellar medium.
Carl Sagan and Frank Drake considered the hyperfine transition of hydrogen to be a sufficiently universal phenomenon so as to be used as a base unit of time and length on the Pioneer plaque and later Voyager Golden Record.
In submillimeter astronomy, heterodyne receivers are widely used in detecting electromagnetic signals from celestial objects such as star-forming core or young stellar objects. The separations among neighboring components in a hyperfine spectrum of an observed rotational transition are usually small enough to fit within the receiver's IF band. Since the optical depth varies with frequency, strength ratios among the hyperfine components differ from that of their intrinsic (or optically thin) intensities (these are so-called hyperfine anomalies, often observed in the rotational transitions of HCN[12]). Thus, a more accurate determination of the optical depth is possible. From this we can derive the object's physical parameters.[13]
Nuclear spectroscopy[edit]
In nuclear spectroscopy methods, the nucleus is used to probe the local structure in materials. The methods mainly base on hyperfine interactions with the surrounding atoms and ions. Important methods are nuclear magnetic resonance, Mössbauer spectroscopy, and perturbed angular correlation.
Nuclear technology[edit]
The atomic vapor laser isotope separation (AVLIS) process uses the hyperfine splitting between optical transitions in uranium-235 and uranium-238 to selectively photo-ionize only the uranium-235 atoms and then separate the ionized particles from the non-ionized ones. Precisely tuned dye lasers are used as the sources of the necessary exact wavelength radiation.
Use in defining the SI second and meter[edit]
The hyperfine structure transition can be used to make a microwave notch filter with very high stability, repeatability and Q factor, which can thus be used as a basis for very precise atomic clocks. The term transition frequency denotes the frequency of radiation corresponding to the transition between the two hyperfine levels of the atom, and is equal to f = ΔE/h, where ΔE is difference in energy between the levels and h is the Planck constant. Typically, the transition frequency of a particular isotope of caesium or rubidium atoms is used as a basis for these clocks.
Due to the accuracy of hyperfine structure transition-based atomic clocks, they are now used as the basis for the definition of the second. One second is now defined to be exactly 9192631770cycles of the hyperfine structure transition frequency of caesium-133 atoms.
On October 21, 1983, the 17th CGPM defined the metre as the length of the path travelled by light in a vacuum during a time interval of 1299,792,458 of a second.[14][15]
Precision tests of quantum electrodynamics[edit]
The hyperfine splitting in hydrogen and in muonium have been used to measure the value of the fine structure constant α. Comparison with measurements of α in other physical systems provides a stringent test of QED.
Qubit in ion-trap quantum computing[edit]
The hyperfine states of a trapped ion are commonly used for storing qubits in ion-trap quantum computing. They have the advantage of having very long lifetimes, experimentally exceeding ~10 minutes (compared to ~1 s for metastable electronic levels).
The frequency associated with the states' energy separation is in the microwave region, making it possible to drive hyperfine transitions using microwave radiation. However, at present no emitter is available that can be focused to address a particular ion from a sequence. Instead, a pair of laser pulses can be used to drive the transition, by having their frequency difference (detuning) equal to the required transition's frequency. This is essentially a stimulated Raman transition. In addition, near-field gradients have been exploited to individually address two ions separated by approximately 4.3 micrometers directly with microwave radiation.[16]
See also[edit]
https://en.wikipedia.org/wiki/Hyperfine_structure
In linear algebra, a pseudoscalar is a quantity that behaves like a scalar, except that it changes sign under a parity inversion[1][2] while a true scalar does not.
Any scalar product between a pseudovector and an ordinary vector is a pseudoscalar. The prototypical example of a pseudoscalar is the scalar triple product, which can be written as the scalar product between one of the vectors in the triple product and the cross product between the two other vectors, where the latter is a pseudovector. A pseudoscalar, when multiplied by an ordinary vector, becomes a pseudovector (axial vector); a similar construction creates the pseudotensor.
Mathematically, a pseudoscalar is an element of the top exterior power of a vector space, or the top power of a Clifford algebra; see pseudoscalar (Clifford algebra). More generally, it is an element of the canonical bundle of a differentiable manifold.
In physics[edit]
In physics, a pseudoscalar denotes a physical quantity analogous to a scalar. Both are physical quantities which assume a single value which is invariant under proper rotations. However, under the parity transformation, pseudoscalars flip their signs while scalars do not. As reflections through a plane are the combination of a rotation with the parity transformation, pseudoscalars also change signs under reflections.
https://en.wikipedia.org/wiki/Pseudoscalar
In geometry and physics, spinors /spɪnər/ are elements of a complex vector space that can be associated with Euclidean space.[b] Like geometric vectors and more general tensors, spinors transform linearly when the Euclidean space is subjected to a slight (infinitesimal) rotation.[c] However, when a sequence of such small rotations is composed (integrated) to form an overall final rotation, the resulting spinor transformation depends on which sequence of small rotations was used. Unlike vectors and tensors, a spinor transforms to its negative when the space is continuously rotated through a complete turn from 0° to 360° (see picture). This property characterizes spinors: spinors can be viewed as the "square roots" of vectors (although this is inaccurate and may be misleading; they are better viewed as "square roots" of sections of vector bundles – in the case of the exterior algebra bundle of the cotangent bundle, they thus become "square roots" of differential forms).
It is also possible to associate a substantially similar notion of spinor to Minkowski space, in which case the Lorentz transformations of special relativity play the role of rotations. Spinors were introduced in geometry by Élie Cartan in 1913.[1][d] In the 1920s physicists discovered that spinors are essential to describe the intrinsic angular momentum, or "spin", of the electron and other subatomic particles.[e]
Spinors are characterized by the specific way in which they behave under rotations. They change in different ways depending not just on the overall final rotation, but the details of how that rotation was achieved (by a continuous path in the rotation group). There are two topologically distinguishable classes (homotopy classes) of paths through rotations that result in the same overall rotation, as illustrated by the belt trick puzzle. These two inequivalent classes yield spinor transformations of opposite sign. The spin group is the group of all rotations keeping track of the class.[f] It doubly covers the rotation group, since each rotation can be obtained in two inequivalent ways as the endpoint of a path. The space of spinors by definition is equipped with a (complex) linear representation of the spin group, meaning that elements of the spin group act as linear transformations on the space of spinors, in a way that genuinely depends on the homotopy class.[g] In mathematical terms, spinors are described by a double-valued projective representation of the rotation group SO(3).
Although spinors can be defined purely as elements of a representation space of the spin group (or its Lie algebra of infinitesimal rotations), they are typically defined as elements of a vector space that carries a linear representation of the Clifford algebra. The Clifford algebra is an associative algebra that can be constructed from Euclidean space and its inner product in a basis-independent way. Both the spin group and its Lie algebra are embedded inside the Clifford algebra in a natural way, and in applications the Clifford algebra is often the easiest to work with.[h] A Clifford space operates on a spinor space, and the elements of a spinor space are spinors.[3] After choosing an orthonormal basis of Euclidean space, a representation of the Clifford algebra is generated by gamma matrices, matrices that satisfy a set of canonical anti-commutation relations. The spinors are the column vectors on which these matrices act. In three Euclidean dimensions, for instance, the Pauli spin matrices are a set of gamma matrices,[i] and the two-component complex column vectors on which these matrices act are spinors. However, the particular matrix representation of the Clifford algebra, hence what precisely constitutes a "column vector" (or spinor), involves the choice of basis and gamma matrices in an essential way. As a representation of the spin group, this realization of spinors as (complex[j]) column vectors will either be irreducible if the dimension is odd, or it will decompose into a pair of so-called "half-spin" or Weyl representations if the dimension is even.[k]
https://en.wikipedia.org/wiki/Spinor
https://en.wikipedia.org/wiki/S-factor
Parameterization of cross section[edit]
The probability that fusion occurs is greatly increased compared to the classical picture, thanks to the smearing of the effective radius as the DeBroglie wavelength as well as quantum tunnelling through the potential barrier. To determine the rate of fusion reactions, the value of most interest is the cross section, which describes the probability that particles will fuse by giving a characteristic area of interaction. An estimation of the fusion cross-sectional area is often broken into three pieces:
Where is the geometric cross section, T is the barrier transparency and R is the reaction characteristics of the reaction.
is of the order of the square of the de-Broglie wavelength where is the reduced mass of the system and is the center of mass energy of the system.
T can be approximated by the Gamow transparency, which has the form: where is the Gamow factor and comes from estimating the quantum tunneling probability through the potential barrier.
R contains all the nuclear physics of the specific reaction and takes very different values depending on the nature of the interaction. However, for most reactions, the variation of is small compared to the variation from the Gamow factor and so is approximated by a function called the Astrophysical S-factor, , which is weakly varying in energy. Putting these dependencies together, one approximation for the fusion cross section as a function of energy takes the form:
More detailed forms of the cross-section can be derived through nuclear physics-based models and R-matrix theory.
Formulas of fusion cross sections[edit]
The Naval Research Lab's plasma physics formulary[36] gives the total cross section in barns as a function of the energy (in keV) of the incident particle towards a target ion at rest fit by the formula:
with the following coefficient values:
NRL Formulary Cross Section Coefficients DT(1) DD(2i) DD(2ii) DHe3(3) TT(4) The3(6) A1 45.95 46.097 47.88 89.27 38.39 123.1 A2 50200 372 482 25900 448 11250 A3 1.368e-2 4.36e-4 3.08e-4 3.98e-3 1.02e-3 0 A4 1.076 1.22 1.177 1.297 2.09 0 A5 409 0 0 647 0 0
Bosch-Hale[37] also reports a R-matrix calculated cross sections fitting observation data with Padé rational approximating coefficients. With energy in units of keV and cross sections in units of millibarn, the factor has the form:
, with the coefficient values:
Bosch-Hale coefficients for the fusion cross section DT(1) DD(2ii) DHe3(3) THe4 31.3970 68.7508 31.3970 34.3827 A1 5.5576e4 5.7501e6 5.3701e4 6.927e4 A2 2.1054e2 2.5226e3 3.3027e2 7.454e8 A3 -3.2638e-2 4.5566e1 -1.2706e-1 2.050e6 A4 1.4987e-6 0 2.9327e-5 5.2002e4 A5 1.8181e-10 0 -2.5151e-9 0 B1 0 -3.1995e-3 0 6.38e1 B2 0 -8.5530e-6 0 -9.95e-1 B3 0 5.9014e-8 0 6.981e-5 B4 0 0 0 1.728e-4 Applicable Energy Range [keV] 0.5-5000 0.3-900 0.5-4900 0.5-550 2.0 2.2 2.5 1.9
where
https://en.wikipedia.org/wiki/Nuclear_fusion#Criteria_and_candidates_for_terrestrial_reactions
Obtaining the correct abundance of dark matter today via thermal production requires a self-annihilation cross section of , which is roughly what is expected for a new particle in the 100 GeV mass range that interacts via the electroweak force.
WIMP-like particles are predicted by R-parity-conserving supersymmetry, a popular type of extension to the Standard Model of particle physics, although none of the large number of new particles in supersymmetry have been observed.[6] WIMP-like particles are also predicted by universal extra dimension and little Higgs theories.
Model parity candidate SUSY R-parity lightest supersymmetric particle (LSP) UED KK-parity lightest Kaluza-Klein particle (LKP) little Higgs T-parity lightest T-odd particle (LTP)
The main theoretical characteristics of a WIMP are:
- Interactions only through the weak nuclear force and gravity, or possibly other interactions with cross-sections no higher than the weak scale;[7]
- Large mass compared to standard particles (WIMPs with sub-GeV masses may be considered to be light dark matter).
Because of their lack of electromagnetic interaction with normal matter, WIMPs would be invisible through normal electromagnetic observations. Because of their large mass, they would be relatively slow moving and therefore "cold".[8] Their relatively low velocities would be insufficient to overcome the mutual gravitational attraction, and as a result, WIMPs would tend to clump together.[9] WIMPs are considered one of the main candidates for cold dark matter, the others being massive compact halo objects (MACHOs) and axions. (These names were deliberately chosen for contrast, with MACHOs named later than WIMPs.[10]) Also, in contrast to MACHOs, there are no known stable particles within the Standard Model of particle physics that have all the properties of WIMPs. The particles that have little interaction with normal matter, such as neutrinos, are all very light, and hence would be fast moving, or "hot".
https://en.wikipedia.org/wiki/Weakly_interacting_massive_particles
https://en.wikipedia.org/wiki/Preon
https://en.wikipedia.org/wiki/Pressuron
https://en.wikipedia.org/wiki/Spinor
Hyperfine Structure
Qubit in ion-trap quantum computing[edit]
The hyperfine states of a trapped ion are commonly used for storing qubits in ion-trap quantum computing. They have the advantage of having very long lifetimes, experimentally exceeding ~10 minutes (compared to ~1 s for metastable electronic levels).
The frequency associated with the states' energy separation is in the microwave region, making it possible to drive hyperfine transitions using microwave radiation. However, at present no emitter is available that can be focused to address a particular ion from a sequence. Instead, a pair of laser pulses can be used to drive the transition, by having their frequency difference (detuning) equal to the required transition's frequency. This is essentially a stimulated Raman transition. In addition, near-field gradients have been exploited to individually address two ions separated by approximately 4.3 micrometers directly with microwave radiation.[16]
See also[edit]
https://en.wikipedia.org/wiki/Hyperfine_structure
Multipole magnets[edit]
Modern systems often use multipole magnets, such as quadrupole and sextupole magnets, to focus the beam down, as magnets give a more powerful deflection effect than earlier electrostatic systems at high beam kinetic energies. The multipole magnets refocus the beam after each deflection section, as deflection sections have a defocusing effect that can be countered with a convergent magnet 'lens'.
This can be shown schematically as a sequence of divergent and convergent lenses. The quadrupoles are often laid out in what are called FODO patterns (where F focusses vertically and defocusses horizontally, and D focusses horizontally and defocusses vertically and O is a space or deflection magnet). Following the beam particles in their trajectories through the focusing arrangement, an oscillating pattern would be seen.
Mathematical modeling[edit]
The action upon a set of charged particles by a set of linear magnets (i.e. only dipoles, quadrupoles and the field-free drift regions between them) can be expressed as matrices which can be multiplied together to give their net effect, using ray transfer matrix analysis.[7] Higher-order terms such as sextupoles, octupoles etc. may be treated by a variety of methods, depending on the phenomena of interest.
See also[edit]
- Electron gun – uses cylindrical symmetric fields such as provided by a Wehnelt cylinder to focus an electron beam
- Maglev – has also been a suggested use of strong focusing
- Quadrupole magnet
- Sextupole magnet
- Nicholas Christofilos – the scientist who first conceived strong focusing
https://en.wikipedia.org/wiki/Strong_focusing
https://en.wikipedia.org/wiki/Massive_gravity#The_Boulware-Deser_ghost
https://en.wikipedia.org/wiki/Dynamic_nuclear_polarization
https://en.wikipedia.org/wiki/Electron_paramagnetic_resonance
https://en.wikipedia.org/wiki/Superdiamagnetism
The Meissner effect (or Meissner–Ochsenfeld effect) is the expulsion of a magnetic field from a superconductor during its transition to the superconducting state when it is cooled below the critical temperature. This expulsion will repel a nearby magnet.
https://en.wikipedia.org/wiki/Meissner_effect
An electron gun (also called electron emitter) is an electrical component in some vacuum tubes that produces a narrow, collimated electron beam that has a precise kinetic energy. The largest use is in cathode ray tubes (CRTs), used in nearly all television sets, computer displays and oscilloscopes that are not flat-panel displays. They are also used in field emission displays (FEDs), which are essentially flat-panel displays made out of rows of extremely small cathode ray tubes. They are also used in microwave linear beam vacuum tubes such as klystrons, inductive output tubes, travelling wave tubes, and gyrotrons, as well as in scientific instruments such as electron microscopes and particle accelerators. Electron guns may be classified by the type of electric field generation (DC or RF), by emission mechanism (thermionic, photocathode, cold emission, plasmas source), by focusing (pure electrostatic or with magnetic fields), or by the number of electrodes.
https://en.wikipedia.org/wiki/Electron_gun
https://en.wikipedia.org/wiki/Hyperloop
https://en.wikipedia.org/wiki/Electrodynamic_suspension#Levitation_melting
Aerodynamic levitation as a scientific tool[edit]
These systems allow spherical samples to be levitated by passing gas up through a diverging conical nozzle. Combining this with >200W continuous CO2laser heating allows sample temperatures in excess of 3000 degrees Celsius to be achieved.
When heating materials to these extremely high temperatures levitation in general provides two key advantages over traditional furnaces. First, contamination that would otherwise occur from a solid container is eliminated. Second, the sample can be undercooled, i.e. cooled below its normal freezing temperature without actually freezing.
These systems allow spherical samples to be levitated by passing gas up through a diverging conical nozzle. Combining this with >200W continuous CO2laser heating allows sample temperatures in excess of 3000 degrees Celsius to be achieved.
When heating materials to these extremely high temperatures levitation in general provides two key advantages over traditional furnaces. First, contamination that would otherwise occur from a solid container is eliminated. Second, the sample can be undercooled, i.e. cooled below its normal freezing temperature without actually freezing.
Undercooling of liquid samples[edit]
Undercooling, or supercooling, is the cooling of a liquid below its equilibrium freezing temperature while it remains a liquid. This can occur wherever crystal nucleation is suppressed. In levitated samples, heterogeneous nucleation is suppressed due to lack of contact with a solid surface. Levitation techniques typically allow samples to be cooled several hundred degrees Celsius below their equilibrium freezing temperatures.
Undercooling, or supercooling, is the cooling of a liquid below its equilibrium freezing temperature while it remains a liquid. This can occur wherever crystal nucleation is suppressed. In levitated samples, heterogeneous nucleation is suppressed due to lack of contact with a solid surface. Levitation techniques typically allow samples to be cooled several hundred degrees Celsius below their equilibrium freezing temperatures.
Glass produced by aerodynamic levitation[edit]
Since crystal nucleation is suppressed by levitation, and since it is not limited by sample conductivity (unlike electromagnetic levitation), aerodynamic levitation can be used to make glassy materials, from high temperature melts that cannot be made by standard methods. Several silica-free, aluminium oxide based glasses have been made.[2][3][4]
Since crystal nucleation is suppressed by levitation, and since it is not limited by sample conductivity (unlike electromagnetic levitation), aerodynamic levitation can be used to make glassy materials, from high temperature melts that cannot be made by standard methods. Several silica-free, aluminium oxide based glasses have been made.[2][3][4]
Physical property measurements[edit]
In the last few years a range of in situ measurement techniques have also been developed. The following measurements can be made with varying precision:
electrical conductivity, viscosity,[5] density, surface tension,[6] specific heat capacity,
In situ aerodynamic levitation has also been combined with:
X-ray synchrotron radiation, neutron scattering, NMR spectroscopy
In the last few years a range of in situ measurement techniques have also been developed. The following measurements can be made with varying precision:
electrical conductivity, viscosity,[5] density, surface tension,[6] specific heat capacity,
In situ aerodynamic levitation has also been combined with:
X-ray synchrotron radiation, neutron scattering, NMR spectroscopy
See also[edit]
https://en.wikipedia.org/wiki/Aerodynamic_levitation
Electrostatic levitation is the process of using an electric field to levitate a charged object and counteract the effects of gravity. It was used, for instance, in Robert Millikan's oil drop experiment and is used to suspend the gyroscopes in Gravity Probe B during launch.
Due to Earnshaw's theorem, no static arrangement of classical electrostatic fields can be used to stably levitate a point charge. There is an equilibrium point where the two fields cancel, but it is an unstable equilibrium. By using feedback techniques it is possible to adjust the charges to achieve a quasi static levitation.
https://en.wikipedia.org/wiki/Electrostatic_levitation
Electrostatic levitation is the process of using an electric field to levitate a charged object and counteract the effects of gravity. It was used, for instance, in Robert Millikan's oil drop experiment and is used to suspend the gyroscopes in Gravity Probe B during launch.
Due to Earnshaw's theorem, no static arrangement of classical electrostatic fields can be used to stably levitate a point charge. There is an equilibrium point where the two fields cancel, but it is an unstable equilibrium. By using feedback techniques it is possible to adjust the charges to achieve a quasi static levitation.
https://en.wikipedia.org/wiki/Electrostatic_levitation
Optical levitation[edit]
In order to levitate the particle in air, the downward force of gravity must be countered by the forces stemming from photon momentum transfer. Typically photon radiation pressure of a focused laser beam of enough intensity counters the downward force of gravity while also preventing lateral (side to side) and vertical instabilities to allow for a stable optical trap capable of holding small particles in suspension.
Micrometer sized (from several to 50 micrometers in diameter) transparent dielectric spheres such as fused silica spheres, oil or water droplets, are used in this type of experiment. The laser radiation can be fixed in wavelength such as that of an argon ion laser or that of a tunable dye laser. Laser power required is of the order of 1 Watt focused to a spot size of several tens of micrometers. Phenomena related to morphology-dependent resonances in a spherical optical cavity have been studied by several research groups.
For a shiny object, such as a metallic micro-sphere, stable optical levitation has not been achieved. Optical levitation of a macroscopic object is also theoretically possible,[23] and can be enhanced with nano-structuring.[24]
Materials that have been successfully levitated include Black liquor, aluminum oxide, tungsten, and nickel.[25]
https://en.wikipedia.org/wiki/Optical_tweezers#Optical_levitation
A cyclotron is a type of particle accelerator invented by Ernest O. Lawrence in 1929–1930 at the University of California, Berkeley,[1][2] and patented in 1932.[3][4] A cyclotron accelerates charged particles outwards from the center of a flat cylindrical vacuum chamber along a spiral path.[5][6] The particles are held to a spiral trajectory by a static magnetic field and accelerated by a rapidly varying (radio frequency) electric field. Lawrence was awarded the 1939 Nobel Prize in Physics for this invention.[6][7]
Cyclotrons were the most powerful particle accelerator technology until the 1950s when they were superseded by the synchrotron, and are still used to produce particle beams in physics and nuclear medicine. The largest single-magnet cyclotron was the 4.67 m (184 in) synchrocyclotronbuilt between 1940 and 1946 by Lawrence at the University of California, Berkeley,[1][6] which could accelerate protons to 730 mega electron volts (MeV). The largest cyclotron of its kind is the 17.1 m (56 ft) multimagnet TRIUMF accelerator at the University of British Columbia in Vancouver, British Columbia, which can produce 520 MeV protons.
Close to 1500 cyclotrons are used in nuclear medicine worldwide for the production of radionuclides.[8]
https://en.wikipedia.org/wiki/Cyclotron
https://en.wikipedia.org/wiki/Launch_loop
https://en.wikipedia.org/wiki/Synchronous_motor
In order to levitate the particle in air, the downward force of gravity must be countered by the forces stemming from photon momentum transfer. Typically photon radiation pressure of a focused laser beam of enough intensity counters the downward force of gravity while also preventing lateral (side to side) and vertical instabilities to allow for a stable optical trap capable of holding small particles in suspension.
Micrometer sized (from several to 50 micrometers in diameter) transparent dielectric spheres such as fused silica spheres, oil or water droplets, are used in this type of experiment. The laser radiation can be fixed in wavelength such as that of an argon ion laser or that of a tunable dye laser. Laser power required is of the order of 1 Watt focused to a spot size of several tens of micrometers. Phenomena related to morphology-dependent resonances in a spherical optical cavity have been studied by several research groups.
For a shiny object, such as a metallic micro-sphere, stable optical levitation has not been achieved. Optical levitation of a macroscopic object is also theoretically possible,[23] and can be enhanced with nano-structuring.[24]
Materials that have been successfully levitated include Black liquor, aluminum oxide, tungsten, and nickel.[25]
https://en.wikipedia.org/wiki/Optical_tweezers#Optical_levitation
A cyclotron is a type of particle accelerator invented by Ernest O. Lawrence in 1929–1930 at the University of California, Berkeley,[1][2] and patented in 1932.[3][4] A cyclotron accelerates charged particles outwards from the center of a flat cylindrical vacuum chamber along a spiral path.[5][6] The particles are held to a spiral trajectory by a static magnetic field and accelerated by a rapidly varying (radio frequency) electric field. Lawrence was awarded the 1939 Nobel Prize in Physics for this invention.[6][7]
Cyclotrons were the most powerful particle accelerator technology until the 1950s when they were superseded by the synchrotron, and are still used to produce particle beams in physics and nuclear medicine. The largest single-magnet cyclotron was the 4.67 m (184 in) synchrocyclotronbuilt between 1940 and 1946 by Lawrence at the University of California, Berkeley,[1][6] which could accelerate protons to 730 mega electron volts (MeV). The largest cyclotron of its kind is the 17.1 m (56 ft) multimagnet TRIUMF accelerator at the University of British Columbia in Vancouver, British Columbia, which can produce 520 MeV protons.
Close to 1500 cyclotrons are used in nuclear medicine worldwide for the production of radionuclides.[8]
https://en.wikipedia.org/wiki/Cyclotron
https://en.wikipedia.org/wiki/Launch_loop
https://en.wikipedia.org/wiki/Synchronous_motor
Saturday, September 18, 2021
09-18-2021-0747 - Levitron
Levitron is a brand of levitating toys and gifts in science and educational markets marketed by Creative Gifts Inc. and Fascination Toys & Gifts.[1]The Levitron top device is a commercial toy under this brand that displays the phenomenon known as spin-stabilized magnetic levitation. This method, with moving permanent magnets, is quite distinct from other versions which use changing electromagnetic fields, levitating various items such as a rotating world globe, model space shuttle or VW Beetle, and picture frame.[2] 750,000 units of the top were sold from 1994 through 1999.[1]
https://en.wikipedia.org/wiki/Levitron
Levitron is a brand of levitating toys and gifts in science and educational markets marketed by Creative Gifts Inc. and Fascination Toys & Gifts.[1]The Levitron top device is a commercial toy under this brand that displays the phenomenon known as spin-stabilized magnetic levitation. This method, with moving permanent magnets, is quite distinct from other versions which use changing electromagnetic fields, levitating various items such as a rotating world globe, model space shuttle or VW Beetle, and picture frame.[2] 750,000 units of the top were sold from 1994 through 1999.[1]
https://en.wikipedia.org/wiki/Levitron
Saturday, September 18, 2021
09-18-2021-0414 - Psuedoforce Notes Drafting
Silver catalysis[edit]
Recently, the discovery of a general Ag(I)-catalyzed azide–alkyne cycloaddition reaction (Ag-AAC) leading to 1,4-triazoles is reported. Mechanistic features are similar to the generally accepted mechanism of the copper(I)-catalyzed process. Silver(I)-salts alone are not sufficient to promote the cycloaddition. However the ligated Ag(I) source has proven to be exceptional for AgAAC reaction.[24][25] Curiously, pre-formed silver acetylides do not react with azides; however, silver acetylides do react with azides under catalysis with copper(I).[26]
https://en.wikipedia.org/wiki/Azide-alkyne_Huisgen_cycloaddition
Recently, the discovery of a general Ag(I)-catalyzed azide–alkyne cycloaddition reaction (Ag-AAC) leading to 1,4-triazoles is reported. Mechanistic features are similar to the generally accepted mechanism of the copper(I)-catalyzed process. Silver(I)-salts alone are not sufficient to promote the cycloaddition. However the ligated Ag(I) source has proven to be exceptional for AgAAC reaction.[24][25] Curiously, pre-formed silver acetylides do not react with azides; however, silver acetylides do react with azides under catalysis with copper(I).[26]
https://en.wikipedia.org/wiki/Azide-alkyne_Huisgen_cycloaddition
Saturday, September 18, 2021
09-18-2021-0818 - Thiol-yne reaction
Saturday, September 18, 2021
09-18-2021-0919 - Maglev
https://en.wikipedia.org/wiki/Linear_induction_motorA linear induction motor (LIM) is an alternating current (AC), asynchronous linear motor that works by the same general principles as other induction motorsbut is typically designed to directly produce motion in a straight line. Characteristically, linear induction motors have a finite primary or secondary length, which generates end-effects, whereas a conventional induction motor is arranged in an endless loop.[1]
Despite their name, not all linear induction motors produce linear motion; some linear induction motors are employed for generating rotations of large diameters where the use of a continuous primary would be very expensive.
As with rotary motors, linear motors frequently run on a three-phase power supply and can support very high speeds. However, there are end-effects that reduce the motor's force, and it is often not possible to fit a gearbox to trade off force and speed. Linear induction motors are thus frequently less energy efficient than normal rotary motors for any given required force output.
LIMs, unlike their rotary counterparts, can give a levitation effect. They are therefore often used where contactless force is required, where low maintenance is desirable, or where the duty cycle is low. Their practical uses include magnetic levitation, linear propulsion, and linear actuators. They have also been used for pumping liquid metals.[2]
https://en.wikipedia.org/wiki/Linear_induction_motor
https://en.wikipedia.org/wiki/Linear_motor
https://en.wikipedia.org/wiki/Regenerative_brake
https://en.wikipedia.org/wiki/Vactrain
https://en.wikipedia.org/wiki/Linear_motor
https://en.wikipedia.org/wiki/Atmospheric_railway
https://en.wikipedia.org/wiki/StarTram
https://en.wikipedia.org/wiki/Category:Magnetic_propulsion_devices
https://en.wikipedia.org/wiki/Category:Electrodynamics
https://en.wikipedia.org/wiki/Halbach_array
https://en.wikipedia.org/wiki/Earnshaw%27s_theorem
https://en.wikipedia.org/wiki/Drag_(physics)
https://en.wikipedia.org/wiki/Linear_stage
https://en.wikipedia.org/wiki/Electromagnetic_Aircraft_Launch_System
https://en.wikipedia.org/wiki/Aircraft_catapult#Steam_catapult
https://en.wikipedia.org/wiki/Spacecraft_propulsion
https://en.wikipedia.org/wiki/Escape_velocity
https://en.wikipedia.org/wiki/Electric_generator
https://en.wikipedia.org/wiki/Flywheel
https://en.wikipedia.org/wiki/Spindle_(textiles)
https://en.wikipedia.org/wiki/Flywheel_energy_storage
https://en.wikipedia.org/wiki/Rotational_energy
https://en.wikipedia.org/wiki/Rotational_speed
https://en.wikipedia.org/wiki/Inductor
https://en.wikipedia.org/wiki/Accumulator_(energy)
https://en.wikipedia.org/wiki/Reciprocating_engine
https://en.wikipedia.org/wiki/Reaction_wheel
https://en.wikipedia.org/wiki/Gyroscope
https://en.wikipedia.org/wiki/Homopolar_generator
https://en.wikipedia.org/wiki/Electrolysis
https://en.wikipedia.org/wiki/Angular_momentum
https://en.wikipedia.org/wiki/Spectrum_(functional_analysis)#Point_spectrum
https://en.wikipedia.org/wiki/Synchrotron
https://en.wikipedia.org/wiki/Coilgun
https://en.wikipedia.org/wiki/Carl_Friedrich_Gauss
https://en.wikipedia.org/wiki/Self-interacting_dark_matter
https://en.wikipedia.org/wiki/Scalar_field_dark_matter
https://en.wikipedia.org/wiki/Minimal_Supersymmetric_Standard_Model
https://en.wikipedia.org/wiki/Neutralino
https://en.wikipedia.org/wiki/Fuzzy_cold_dark_matter
https://en.wikipedia.org/wiki/Cosmic_microwave_background
https://en.wikipedia.org/wiki/Superfluidity
https://en.wikipedia.org/wiki/Supercritical_fluid
https://en.wikipedia.org/wiki/Supercritical_flow
https://en.wikipedia.org/wiki/Sonic_black_hole
https://en.wikipedia.org/wiki/Optical_black_hole
https://en.wikipedia.org/wiki/Analog_models_of_gravity
https://en.wikipedia.org/wiki/Transformation_optics
https://en.wikipedia.org/wiki/Black_hole
https://en.wikipedia.org/wiki/Cold_dark_matter
https://en.wikipedia.org/wiki/Primordial_black_hole
https://en.wikipedia.org/wiki/Neutrino_oscillation
https://en.wikipedia.org/wiki/Rotating_black_hole
https://en.wikipedia.org/wiki/Spin-flip
https://en.wikipedia.org/wiki/Black_hole_bomb
https://en.wikipedia.org/wiki/Neutron_reflector
https://en.wikipedia.org/wiki/Mirror_matter
https://en.wikipedia.org/wiki/Photofission
A linear induction motor (LIM) is an alternating current (AC), asynchronous linear motor that works by the same general principles as other induction motorsbut is typically designed to directly produce motion in a straight line. Characteristically, linear induction motors have a finite primary or secondary length, which generates end-effects, whereas a conventional induction motor is arranged in an endless loop.[1]
Despite their name, not all linear induction motors produce linear motion; some linear induction motors are employed for generating rotations of large diameters where the use of a continuous primary would be very expensive.
As with rotary motors, linear motors frequently run on a three-phase power supply and can support very high speeds. However, there are end-effects that reduce the motor's force, and it is often not possible to fit a gearbox to trade off force and speed. Linear induction motors are thus frequently less energy efficient than normal rotary motors for any given required force output.
LIMs, unlike their rotary counterparts, can give a levitation effect. They are therefore often used where contactless force is required, where low maintenance is desirable, or where the duty cycle is low. Their practical uses include magnetic levitation, linear propulsion, and linear actuators. They have also been used for pumping liquid metals.[2]
https://en.wikipedia.org/wiki/Linear_induction_motor
https://en.wikipedia.org/wiki/Linear_motor
https://en.wikipedia.org/wiki/Regenerative_brake
https://en.wikipedia.org/wiki/Vactrain
https://en.wikipedia.org/wiki/Linear_motor
https://en.wikipedia.org/wiki/Atmospheric_railway
https://en.wikipedia.org/wiki/StarTram
https://en.wikipedia.org/wiki/Category:Magnetic_propulsion_devices
https://en.wikipedia.org/wiki/Category:Electrodynamics
https://en.wikipedia.org/wiki/Halbach_array
https://en.wikipedia.org/wiki/Earnshaw%27s_theorem
https://en.wikipedia.org/wiki/Drag_(physics)
https://en.wikipedia.org/wiki/Linear_stage
https://en.wikipedia.org/wiki/Electromagnetic_Aircraft_Launch_System
https://en.wikipedia.org/wiki/Aircraft_catapult#Steam_catapult
https://en.wikipedia.org/wiki/Spacecraft_propulsion
https://en.wikipedia.org/wiki/Escape_velocity
https://en.wikipedia.org/wiki/Electric_generator
https://en.wikipedia.org/wiki/Flywheel
https://en.wikipedia.org/wiki/Spindle_(textiles)
https://en.wikipedia.org/wiki/Flywheel_energy_storage
https://en.wikipedia.org/wiki/Rotational_energy
https://en.wikipedia.org/wiki/Rotational_speed
https://en.wikipedia.org/wiki/Inductor
https://en.wikipedia.org/wiki/Accumulator_(energy)
https://en.wikipedia.org/wiki/Reciprocating_engine
https://en.wikipedia.org/wiki/Reaction_wheel
https://en.wikipedia.org/wiki/Gyroscope
https://en.wikipedia.org/wiki/Homopolar_generator
https://en.wikipedia.org/wiki/Electrolysis
https://en.wikipedia.org/wiki/Angular_momentum
https://en.wikipedia.org/wiki/Spectrum_(functional_analysis)#Point_spectrum
https://en.wikipedia.org/wiki/Synchrotron
https://en.wikipedia.org/wiki/Coilgun
https://en.wikipedia.org/wiki/Carl_Friedrich_Gauss
https://en.wikipedia.org/wiki/Self-interacting_dark_matter
https://en.wikipedia.org/wiki/Scalar_field_dark_matter
https://en.wikipedia.org/wiki/Minimal_Supersymmetric_Standard_Model
https://en.wikipedia.org/wiki/Neutralino
https://en.wikipedia.org/wiki/Fuzzy_cold_dark_matter
https://en.wikipedia.org/wiki/Cosmic_microwave_background
https://en.wikipedia.org/wiki/Superfluidity
https://en.wikipedia.org/wiki/Supercritical_fluid
https://en.wikipedia.org/wiki/Supercritical_flow
https://en.wikipedia.org/wiki/Sonic_black_hole
https://en.wikipedia.org/wiki/Optical_black_hole
https://en.wikipedia.org/wiki/Analog_models_of_gravity
https://en.wikipedia.org/wiki/Transformation_optics
https://en.wikipedia.org/wiki/Black_hole
https://en.wikipedia.org/wiki/Cold_dark_matter
https://en.wikipedia.org/wiki/Primordial_black_hole
https://en.wikipedia.org/wiki/Neutrino_oscillation
https://en.wikipedia.org/wiki/Rotating_black_hole
https://en.wikipedia.org/wiki/Spin-flip
https://en.wikipedia.org/wiki/Black_hole_bomb
https://en.wikipedia.org/wiki/Neutron_reflector
https://en.wikipedia.org/wiki/Mirror_matter
https://en.wikipedia.org/wiki/Photofission
Inertial confinement[edit]
Inertial confinement is the use of rapid implosion to heat and confine plasma. A shell surrounding the fuel is imploded using a direct laser blast (direct drive), a secondary x-ray blast (indirect drive), or heavy beams. The fuel must be compressed to about 30 times solid density with energetic beams. Direct drive can in principle be efficient, but insufficient uniformity has prevented success.[65]:19-20 Indirect drive uses beams to heat a shell, driving the shell to radiate x-rays, which then implode the pellet. The beams are commonly laser beams, but ion and electron beams have been investigated.[65]:182-193
Inertial confinement is the use of rapid implosion to heat and confine plasma. A shell surrounding the fuel is imploded using a direct laser blast (direct drive), a secondary x-ray blast (indirect drive), or heavy beams. The fuel must be compressed to about 30 times solid density with energetic beams. Direct drive can in principle be efficient, but insufficient uniformity has prevented success.[65]:19-20 Indirect drive uses beams to heat a shell, driving the shell to radiate x-rays, which then implode the pellet. The beams are commonly laser beams, but ion and electron beams have been investigated.[65]:182-193
Electrostatic confinement[edit]
Electrostatic confinement fusion devices use electrostatic fields. The best known is the fusor. This device has a cathode inside an anode wire cage. Positive ions fly towards the negative inner cage, and are heated by the electric field in the process. If they miss the inner cage they can collide and fuse. Ions typically hit the cathode, however, creating prohibitory high conduction losses. Fusion rates in fusors are low because of competing physical effects, such as energy loss in the form of light radiation.[66] Designs have been proposed to avoid the problems associated with the cage, by generating the field using a non-neutral cloud. These include a plasma oscillating device,[67] a magnetically-shielded-grid,[68] a penning trap, the polywell,[69] and the F1 cathode driver concept.[70]
https://en.wikipedia.org/wiki/Fusion_power#Magnetic_Mirror
Electrostatic confinement fusion devices use electrostatic fields. The best known is the fusor. This device has a cathode inside an anode wire cage. Positive ions fly towards the negative inner cage, and are heated by the electric field in the process. If they miss the inner cage they can collide and fuse. Ions typically hit the cathode, however, creating prohibitory high conduction losses. Fusion rates in fusors are low because of competing physical effects, such as energy loss in the form of light radiation.[66] Designs have been proposed to avoid the problems associated with the cage, by generating the field using a non-neutral cloud. These include a plasma oscillating device,[67] a magnetically-shielded-grid,[68] a penning trap, the polywell,[69] and the F1 cathode driver concept.[70]
https://en.wikipedia.org/wiki/Fusion_power#Magnetic_Mirror
Heating[edit]
- Electrostatic heating: an electric field can do work on charged ions or electrons, heating them.[43]
- Neutral beam injection: hydrogen is ionized and accelerated by an electric field to form a charged beam that is shone through a source of neutral hydrogen gas towards the plasma which itself is ionized and contained by a magnetic field. Some of the intermediate hydrogen gas is accelerated towards the plasma by collisions with the charged beam while remaining neutral: this neutral beam is thus unaffected by the magnetic field and so reaches the plasma. Once inside the plasma the neutral beam transmits energy to the plasma by collisions which ionize it and allow it to be contained by the magnetic field, thereby both heating and refueling the reactor in one operation. The remainder of the charged beam is diverted by magnetic fields onto cooled beam dumps.[44]
- Radio frequency heating: a radio wave causes the plasma to oscillate (i.e., microwave oven). This is also known as electron cyclotron resonance heating or dielectric heating.[45]
- Magnetic reconnection: when plasma gets dense, its electromagnetic properties can change, which can lead to magnetic reconnection. Reconnection helps fusion because it instantly dumps energy into a plasma, heating it quickly. Up to 45% of the magnetic field energy can heat the ions.[46][47]
- Magnetic oscillations: varying electrical currents can be supplied to magnetic coils that heat plasma confined within a magnetic wall.[48]
- Antiproton annihilation: antiprotons injected into a mass of fusion fuel can induce thermonuclear reactions. This possibility as a method of spacecraft propulsion, known as antimatter-catalyzed nuclear pulse propulsion, was investigated at Pennsylvania State University in connection with the proposed AIMStar project.[citation needed]
https://en.wikipedia.org/wiki/Fusion_power
Alternating current (AC) is an electric current which periodically reverses direction and changes its magnitude continuously with time in contrast to direct current(DC) which flows only in one direction. Alternating current is the form in which electric power is delivered to businesses and residences, and it is the form of electrical energy that consumers typically use when they plug kitchen appliances, televisions, fans and electric lamps into a wall socket. A common source of DC power is a battery cell in a flashlight. The abbreviations AC and DC are often used to mean simply alternating and direct, as when they modify current or voltage.[1][2]
The usual waveform of alternating current in most electric power circuits is a sine wave, whose positive half-period corresponds with positive direction of the current and vice versa. In certain applications, like guitar amplifiers, different waveforms are used, such as triangular waves or square waves. Audio and radiosignals carried on electrical wires are also examples of alternating current. These types of alternating current carry information such as sound (audio) or images (video) sometimes carried by modulation of an AC carrier signal. These currents typically alternate at higher frequencies than those used in power transmission.
https://en.wikipedia.org/wiki/Alternating_current
The perturbed γ-γ angular correlation, PAC for short or PAC-Spectroscopy, is a method of nuclear solid-state physics with which magnetic and electric fields in crystal structures can be measured. In doing so, electrical field gradients and the Larmor frequency in magnetic fields as well as dynamic effects are determined. With this very sensitive method, which requires only about 10-1000 billion atoms of a radioactive isotope per measurement, material properties in the local structure, phase transitions, magnetism and diffusion can be investigated. The PAC method is related to nuclear magnetic resonance and the Mössbauer effect, but shows no signal attenuation at very high temperatures. Today only the time-differential perturbed angular correlation (TDPAC) is used.
https://en.wikipedia.org/wiki/Perturbed_angular_correlation
https://en.wikipedia.org/wiki/Analog
https://en.wikipedia.org/wiki/Superheterodyne_receiver
https://en.wikipedia.org/wiki/Gyroscope
https://en.wikipedia.org/wiki/Accumulator_(energy)
https://en.wikipedia.org/wiki/Nuclear_fusion#Criteria_and_candidates_for_terrestrial_reactions
https://www.osti.gov/servlets/purl/5545055
https://www.science.gov/topicpages/a/accelerated+beam+experiments
https://www.mdpi.com/1996-1073/3/5/1014/htm
- Electrostatic heating: an electric field can do work on charged ions or electrons, heating them.[43]
- Neutral beam injection: hydrogen is ionized and accelerated by an electric field to form a charged beam that is shone through a source of neutral hydrogen gas towards the plasma which itself is ionized and contained by a magnetic field. Some of the intermediate hydrogen gas is accelerated towards the plasma by collisions with the charged beam while remaining neutral: this neutral beam is thus unaffected by the magnetic field and so reaches the plasma. Once inside the plasma the neutral beam transmits energy to the plasma by collisions which ionize it and allow it to be contained by the magnetic field, thereby both heating and refueling the reactor in one operation. The remainder of the charged beam is diverted by magnetic fields onto cooled beam dumps.[44]
- Radio frequency heating: a radio wave causes the plasma to oscillate (i.e., microwave oven). This is also known as electron cyclotron resonance heating or dielectric heating.[45]
- Magnetic reconnection: when plasma gets dense, its electromagnetic properties can change, which can lead to magnetic reconnection. Reconnection helps fusion because it instantly dumps energy into a plasma, heating it quickly. Up to 45% of the magnetic field energy can heat the ions.[46][47]
- Magnetic oscillations: varying electrical currents can be supplied to magnetic coils that heat plasma confined within a magnetic wall.[48]
- Antiproton annihilation: antiprotons injected into a mass of fusion fuel can induce thermonuclear reactions. This possibility as a method of spacecraft propulsion, known as antimatter-catalyzed nuclear pulse propulsion, was investigated at Pennsylvania State University in connection with the proposed AIMStar project.[citation needed]
Alternating current (AC) is an electric current which periodically reverses direction and changes its magnitude continuously with time in contrast to direct current(DC) which flows only in one direction. Alternating current is the form in which electric power is delivered to businesses and residences, and it is the form of electrical energy that consumers typically use when they plug kitchen appliances, televisions, fans and electric lamps into a wall socket. A common source of DC power is a battery cell in a flashlight. The abbreviations AC and DC are often used to mean simply alternating and direct, as when they modify current or voltage.[1][2]
The usual waveform of alternating current in most electric power circuits is a sine wave, whose positive half-period corresponds with positive direction of the current and vice versa. In certain applications, like guitar amplifiers, different waveforms are used, such as triangular waves or square waves. Audio and radiosignals carried on electrical wires are also examples of alternating current. These types of alternating current carry information such as sound (audio) or images (video) sometimes carried by modulation of an AC carrier signal. These currents typically alternate at higher frequencies than those used in power transmission.
https://en.wikipedia.org/wiki/Alternating_current
The perturbed γ-γ angular correlation, PAC for short or PAC-Spectroscopy, is a method of nuclear solid-state physics with which magnetic and electric fields in crystal structures can be measured. In doing so, electrical field gradients and the Larmor frequency in magnetic fields as well as dynamic effects are determined. With this very sensitive method, which requires only about 10-1000 billion atoms of a radioactive isotope per measurement, material properties in the local structure, phase transitions, magnetism and diffusion can be investigated. The PAC method is related to nuclear magnetic resonance and the Mössbauer effect, but shows no signal attenuation at very high temperatures. Today only the time-differential perturbed angular correlation (TDPAC) is used.
https://en.wikipedia.org/wiki/Perturbed_angular_correlation
https://en.wikipedia.org/wiki/Analog
https://en.wikipedia.org/wiki/Superheterodyne_receiver
https://en.wikipedia.org/wiki/Gyroscope
https://en.wikipedia.org/wiki/Accumulator_(energy)
https://en.wikipedia.org/wiki/Nuclear_fusion#Criteria_and_candidates_for_terrestrial_reactions
https://www.osti.gov/servlets/purl/5545055
https://www.science.gov/topicpages/a/accelerated+beam+experiments
https://www.mdpi.com/1996-1073/3/5/1014/htm
































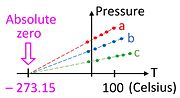












































No comments:
Post a Comment