Nikiya Anton Bettey 2021: 09-21-2021-1235 - Ground Earth Ground Plane

Tuesday, September 21, 2021

09-21-2021-1235 - Ground Earth Ground Plane

In electrical engineering, ground or earth is a reference point in an electrical circuit from which voltages are measured, a common return path for electric current, or a direct physical connection to the earth.

Electrical circuits may be connected to ground for several reasons. Exposed conductive parts of electrical equipment are connected to ground, so that failures of internal insulation which create dangerous voltages on the parts which could be a shock hazard will trigger protective mechanisms in the circuit such as fuses or circuit breakers which turn off the power. In electric power distribution systems, a protective earth (PE) conductor is an essential part of the safety provided by the earthing system.

Connection to ground also limits the build-up of static electricity when handling flammable products or electrostatic-sensitive devices. In some telegraph and power transmission circuits, the ground itself can be used as one conductor of the circuit, saving the cost of installing a separate return conductor (see single-wire earth return and earth-return telegraph).

For measurement purposes, the Earth serves as a (reasonably) constant potential reference against which other potentials can be measured. An electrical ground system should have an appropriate current-carrying capability to serve as an adequate zero-voltage reference level. In electronic circuit theory, a "ground" is usually idealized as an infinite source or sink for charge, which can absorb an unlimited amount of current without changing its potential. Where a real ground connection has a significant resistance, the approximation of zero potential is no longer valid. Stray voltages or earth potential rise effects will occur, which may create noise in signals or produce an electric shock hazard if large enough.

The use of the term ground (or earth) is so common in electrical and electronics applications that circuits in portable electronic devices such as cell phones and media players as well as circuits in vehicles may be spoken of as having a "ground" or chassis ground connection without any actual connection to the Earth, despite "common" being a more appropriate term for such a connection. This is usually a large conductor attached to one side of the power supply (such as the "ground plane" on a printed circuit board) which serves as the common return path for current from many different components in the circuit.

https://en.wikipedia.org/wiki/Ground_(electricity)

In electrical engineering, a ground plane is an electrically conductive surface, usually connected to electrical ground. The term has two different meanings in separate areas of electrical engineering. In antenna theory, a ground plane is a conducting surface large in comparison to the wavelength, such as the Earth, which is connected to the transmitter's ground wire and serves as a reflecting surface for radio waves. In printed circuit boards, a ground plane is a large area of copper foil on the board which is connected to the power supply ground terminal and serves as a return path for current from different components on the board.

https://en.wikipedia.org/wiki/Ground_plane

An earthing system (UK and IEC) or grounding system (US) connects specific parts of an electric power systemwith the ground, typically the Earth's conductive surface, for safety and functional purposes.^[1] The choice of earthing system can affect the safety and electromagnetic compatibility of the installation. Regulations for earthing systems vary considerably among countries, though most follow the recommendations of the International Electrotechnical Commission. Regulations may identify special cases for earthing in mines, in patient care areas, or in hazardous areas of industrial plants.

In addition to electric power systems, other systems may require grounding for safety or function. Tall structures may have lightning rods as part of a system to protect them from lightning strikes. Telegraph lines may use the Earth as one conductor of a circuit, saving the cost of installation of a return wire over a long circuit. Radio antennas may require particular grounding for operation, as well as to control static electricity and provide lightning protection.

https://en.wikipedia.org/wiki/Earthing_system

In electromagnetism, current sources and sinks are analysis formalisms which distinguish points, areas, or volumes through which electric current enters or exits a system. While current sources or sinks are abstract elements used for analysis, generally they have physical counterparts in real-world applications; e.g. the anode or cathode in a battery. In all cases, each of the opposing terms (source or sink) may refer to the same object, depending on the perspective of the observer and the sign convention being used; there is no intrinsic difference between a source and a sink.

In some cases, the term current source refers to a boundary where charge flows from locations where it is not measured to locations where it is measured. In a similar fashion, a current sink may refer to the boundary where charge flows from locations where it is measured to locations where it is not measured. By analogy to the flow of water, a current source would be like a mountain spring - water flows from its source (a hidden location underground) to the surface where it is easily observed. Using the same analogy, a current sink would be like water flowing down a drain - water travels from where it is observed to where it is not observed.

A source or a sink is defined by which compartment is viewable by the observer.

A source is:
- A flow of positive charges from the "invisible" to the "visible" compartment (i.e. toward the eye), or…
- A flow of negative charges from the "visible" to the "invisible" (away from the eye).
A sink is:
- A flow of positive charges "away from the eye", or…
- A flow of negative charges "toward the eye".

In biology, the schematic barrier in the figure could represent a cell membrane, and as a result, the two compartments could represent the inside and the outside of the cell. Generally speaking the point of observation would be outside the cell. Thus the cell would be termed a sink with respect to any flow of positive charges into it, and the cell would act as a source for any positive charges flowing out of it. Note that when considering the flow of negative charges, the definitions are reversed.

https://en.wikipedia.org/wiki/Current_sources_and_sinks

Local field potentials (LFP) are transient electrical signals generated in nervous and other tissues by the summed and synchronous electrical activity of the individual cells (e.g. neurons) in that tissue. LFP are "extracellular" signals, meaning that they are generated by transient imbalances in ion concentrations in the spaces outside the cells, that result from cellular electrical activity. LFP are 'local' because they are recorded by an electrode placed nearby the generating cells. As a result of the Inverse-square law, such electrodes can only 'see' potentials in spatially limited radius. They are 'potentials' because they are generated by the voltage that results from charge separation in the extracellular space. They are 'field' because those extracellular charge separations essentially create a local electric field. LFP are typically recorded with a high-impedance microelectrode placed in the midst of the population of cells generating it. They can be recorded, for example, via a microelectrode placed in the brain of a human^[1] or animal subject, or in an in vitro brain thin slice.

https://en.wikipedia.org/wiki/Local_field_potential

A current mirror is a circuit designed to copy a current through one active device by controlling the current in another active device of a circuit, keeping the output current constant regardless of loading. The current being "copied" can be, and sometimes is, a varying signal current. Conceptually, an ideal current mirror is simply an ideal inverting current amplifier that reverses the current direction as well. Or it can consist of a current-controlled current source (CCCS). The current mirror is used to provide bias currents and active loads to circuits. It can also be used to model a more realistic current source (since ideal current sources don't exist).

The circuit topology covered here is one that appears in many monolithic ICs. It is a Widlar mirror without an emitter degeneration resistor in the follower (output) transistor. This topology can only be done in an IC, as the matching has to be extremely close and cannot be achieved with discretes.

Another topology is the Wilson current mirror. The Wilson mirror solves the Early effect voltage problem in this design.

Mirror characteristics[edit]

There are three main specifications that characterize a current mirror. The first is the transfer ratio (in the case of a current amplifier) or the output current magnitude (in the case of a constant current source CCS). The second is its AC output resistance, which determines how much the output current varies with the voltage applied to the mirror. The third specification is the minimum voltage drop across the output part of the mirror necessary to make it work properly. This minimum voltage is dictated by the need to keep the output transistor of the mirror in active mode. The range of voltages where the mirror works is called the compliance range and the voltage marking the boundary between good and bad behavior is called the compliance voltage. There are also a number of secondary performance issues with mirrors, for example, temperature stability.

Practical approximations[edit]

For small-signal analysis the current mirror can be approximated by its equivalent Norton impedance.

In large-signal hand analysis, a current mirror is usually and simply approximated by an ideal current source. However, an ideal current source is unrealistic in several respects:

it has infinite AC impedance, while a practical mirror has finite impedance
it provides the same current regardless of voltage, that is, there are no compliance range requirements
it has no frequency limitations, while a real mirror has limitations due to the parasitic capacitances of the transistors
the ideal source has no sensitivity to real-world effects like noise, power-supply voltage variations and component tolerances.

Circuit realizations of current mirrors[edit]

Basic idea[edit]

A bipolar transistor can be used as the simplest current-to-current converter but its transfer ratio would highly depend on temperature variations, β tolerances, etc. To eliminate these undesired disturbances, a current mirror is composed of two cascaded current-to-voltage and voltage-to-current converters placed at the same conditions and having reverse characteristics. It is not obligatory for them to be linear; the only requirement is their characteristics to be mirrorlike (for example, in the BJT current mirror below, they are logarithmic and exponential). Usually, two identical converters are used but the characteristic of the first one is reversed by applying a negative feedback. Thus a current mirror consists of two cascaded equal converters (the first - reversed and the second - direct).

Figure 1: A current mirror implemented with npn bipolar transistors using a resistor to set the reference current I_REF; V_CC = supply voltage

Basic BJT current mirror[edit]

If a voltage is applied to the BJT base-emitter junction as an input quantity and the collector current is taken as an output quantity, the transistor will act as an exponential voltage-to-current converter. By applying a negative feedback (simply joining the base and collector) the transistor can be "reversed" and it will begin acting as the opposite logarithmic current-to-voltage converter; now it will adjust the "output" base-emitter voltage so as to pass the applied "input" collector current.

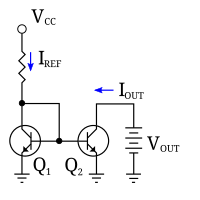
The simplest bipolar current mirror (shown in Figure 1) implements this idea. It consists of two cascaded transistor stages acting accordingly as a reversed and direct voltage-to-current converters. The emitter of transistor Q₁ is connected to ground. Its collector-base voltage is zero as shown. Consequently, the voltage drop across Q₁ is V_BE, that is, this voltage is set by the diode law and Q₁ is said to be diode connected. (See also Ebers-Moll model.) It is important to have Q₁ in the circuit instead of a simple diode, because Q₁ sets V_BE for transistor Q₂. If Q₁ and Q₂ are matched, that is, have substantially the same device properties, and if the mirror output voltage is chosen so the collector-base voltage of Q₂ is also zero, then the V_BE-value set by Q₁ results in an emitter current in the matched Q₂ that is the same as the emitter current in Q₁^{[citation needed]}. Because Q₁ and Q₂ are matched, their β₀-values also agree, making the mirror output current the same as the collector current of Q₁.

The current delivered by the mirror for arbitrary collector-base reverse bias, V_CB, of the output transistor is given by:

I_{\text{C}}=I_{\text{S}}\left(e^{\frac {V_{\text{BE}}}{V_{\text{T}}}}-1\right)\left(1+{\frac {V_{\text{CE}}}{V_{\text{A}}}}\right),

where I_S is the reverse saturation current or scale current; V_T, the thermal voltage; and V_A, the Early voltage. This current is related to the reference current I_refwhen the output transistor V_CB = 0 V by:

I_{\text{ref}}=I_{C}\left(1+{\frac {2}{\beta _{0}}}\right),

as found using Kirchhoff's current law at the collector node of Q₁:

I_{\text{ref}}=I_{C}+I_{B1}+I_{B2}\ .

The reference current supplies the collector current to Q₁ and the base currents to both transistors — when both transistors have zero base-collector bias, the two base currents are equal, I_B1 = I_B2 = I_B.

I_{\text{ref}}=I_{C}+I_{B}+I_{B}=I_{C}+2I_{B}=I_{C}\left(1+{\frac {2}{\beta _{0}}}\right),

Parameter β₀ is the transistor β-value for V_CB = 0 V.

Output resistance[edit]

If V_BC is greater than zero in output transistor Q₂, the collector current in Q₂ will be somewhat larger than for Q₁ due to the Early effect. In other words, the mirror has a finite output (or Norton) resistance given by the r_o of the output transistor, namely:

R_{N}=r_{o}={\frac {V_{A}+V_{CE}}{I_{C}}}\ ,

where V_A is the Early voltage; and V_CE, the collector-to-emitter voltage of output transistor.

Compliance voltage[edit]

To keep the output transistor active, V_CB ≥ 0 V. That means the lowest output voltage that results in correct mirror behavior, the compliance voltage, is V_OUT = V_CV = V_BE under bias conditions with the output transistor at the output current level I_C and with V_CB = 0 V or, inverting the I-V relation above:

V_{CV}=V_{T}\ln \left({\frac {I_{C}}{I_{S}}}+1\right),

where V_T is the thermal voltage; and I_S, the reverse saturation current or scale current.

Extensions and complications[edit]

When Q₂ has V_CB > 0 V, the transistors no longer are matched. In particular, their β-values differ due to the Early effect, with

{\begin{aligned}\beta _{1}&=\beta _{0}\\\beta _{2}&=\beta _{0}\left(1+{\frac {V_{CB}}{V_{A}}}\right),\end{aligned}}

where V_A is the Early voltage and β₀ is the transistor β for V_CB = 0 V. Besides the difference due to the Early effect, the transistor β-values will differ because the β₀-values depend on current, and the two transistors now carry different currents (see Gummel-Poon model).

Further, Q₂ may get substantially hotter than Q₁ due to the associated higher power dissipation. To maintain matching, the temperature of the transistors must be nearly the same. In integrated circuits and transistor arrays where both transistors are on the same die, this is easy to achieve. But if the two transistors are widely separated, the precision of the current mirror is compromised.

Additional matched transistors can be connected to the same base and will supply the same collector current. In other words, the right half of the circuit can be duplicated several times with various resistor values replacing R₂ on each. Note, however, that each additional right-half transistor "steals" a bit of collector current from Q₁ due to the non-zero base currents of the right-half transistors. This will result in a small reduction in the programmed current.

For the simple mirror shown in the diagram, typical values of $\beta$ will yield a current match of 1% or better.

Figure 2: An n-channel MOSFET current mirror with a resistor to set the reference current I_REF; V_DD is the supply voltage

Basic MOSFET current mirror[edit]

The basic current mirror can also be implemented using MOSFET transistors, as shown in Figure 2. Transistor M₁ is operating in the saturation or active mode, and so is M₂. In this setup, the output current I_OUT is directly related to I_REF, as discussed next.

The drain current of a MOSFET I_D is a function of both the gate-source voltage and the drain-to-gate voltage of the MOSFET given by I_D = f (V_GS, V_DG), a relationship derived from the functionality of the MOSFET device. In the case of transistor M₁ of the mirror, I_D = I_REF. Reference current I_REF is a known current, and can be provided by a resistor as shown, or by a "threshold-referenced" or "self-biased" current source to ensure that it is constant, independent of voltage supply variations.^[1]

Using V_DG = 0 for transistor M₁, the drain current in M₁ is I_D = f(V_GS, V_DG=0), so we find: f(V_GS, 0) = I_REF, implicitly determining the value of V_GS. Thus I_REF sets the value of V_GS. The circuit in the diagram forces the same V_GS to apply to transistor M₂. If M₂ is also biased with zero V_DG and provided transistors M₁ and M₂ have good matching of their properties, such as channel length, width, threshold voltage, etc., the relationship I_OUT = f(V_GS, V_DG = 0) applies, thus setting I_OUT = I_REF; that is, the output current is the same as the reference current when V_DG = 0 for the output transistor, and both transistors are matched.

The drain-to-source voltage can be expressed as V_DS = V_DG + V_GS. With this substitution, the Shichman–Hodges model provides an approximate form for function f(V_GS, V_DG):^[2]

{\begin{aligned}I_{d}&=f(V_{GS},V_{DG})\\&={\frac {1}{2}}K_{p}\left({\frac {W}{L}}\right)\left(V_{GS}-V_{th}\right)^{2}\left(1+\lambda V_{DS}\right)\\&={\frac {1}{2}}K_{p}\left[{\frac {W}{L}}\right]\left[V_{GS}-V_{th}\right]^{2}\left[1+\lambda (V_{DG}+V_{GS})\right],\\\end{aligned}}

where $K_{p}$ is a technology-related constant associated with the transistor, W/L is the width to length ratio of the transistor, $V_{GS}$ is the gate-source voltage, $V_{th}$ is the threshold voltage, λ is the channel length modulation constant, and $V_{DS}$ is the drain-source voltage.

Output resistance[edit]

Because of channel-length modulation, the mirror has a finite output (or Norton) resistance given by the r_o of the output transistor, namely (see channel length modulation):

R_{N}=r_{o}={\frac {1}{I_{D}}}\left({\frac {1}{\lambda }}r+V_{DS}\right)={\frac {1}{I_{D}}}\left(V_{E}L+V_{DS}\right),

where λ = channel-length modulation parameter and V_DS = drain-to-source bias.

Compliance voltage[edit]

To keep the output transistor resistance high, V_DG ≥ 0 V.^{[nb 1]} (see Baker).^[3] That means the lowest output voltage that results in correct mirror behavior, the compliance voltage, is V_OUT = V_CV = V_GS for the output transistor at the output current level with V_DG = 0 V, or using the inverse of the f-function, f⁻¹:

V_{CV}=V_{GS}({\text{for}}\ I_{D}\ {\text{at}}\ V_{DG}=0V)=f^{-1}(I_{D})\ {\text{with}}\ V_{DG}=0\ .

For the Shichman–Hodges model, f⁻¹ is approximately a square-root function.

Extensions and reservations[edit]

A useful feature of this mirror is the linear dependence of f upon device width W, a proportionality approximately satisfied even for models more accurate than the Shichman–Hodges model. Thus, by adjusting the ratio of widths of the two transistors, multiples of the reference current can be generated.

The Shichman–Hodges model^[4] is accurate only for rather dated^[when?] technology, although it often is used simply for convenience even today. Any quantitative design based upon new^[when?] technology uses computer models for the devices that account for the changed current-voltage characteristics. Among the differences that must be accounted for in an accurate design is the failure of the square law in V_gs for voltage dependence and the very poor modeling of V_dsdrain voltage dependence provided by λV_ds. Another failure of the equations that proves very significant is the inaccurate dependence upon the channel length L. A significant source of L-dependence stems from λ, as noted by Gray and Meyer, who also note that λ usually must be taken from experimental data.^[5]

Due to the wide variation of V_th even within a particular device number discrete versions are problematic. Although the variation can be somewhat compensated for by using a Source degenerate resistor its value becomes so large that the output resistance suffers (i.e. reduces). This variation relegates the MOSFET version to the IC/monolithic arena.

Feedback-assisted current mirror[edit]

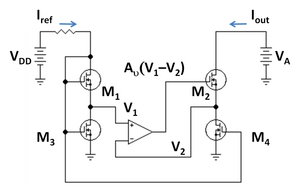
Figure 3: Gain-boosted current mirror with op-amp feedback to increase output resistance

MOSFET version of gain-boosted current mirror; M₁and M₂ are in active mode, while M₃ and M₄ are in Ohmic mode and act like resistors. The operational amplifier provides feedback that maintains a high output resistance.

Figure 3 shows a mirror using negative feedback to increase output resistance. Because of the op amp, these circuits are sometimes called gain-boosted current mirrors. Because they have relatively low compliance voltages, they also are called wide-swing current mirrors. A variety of circuits based upon this idea are in use,^[6]^[7]^[8] particularly for MOSFET mirrors because MOSFETs have rather low intrinsic output resistance values. A MOSFET version of Figure 3 is shown in Figure 4, where MOSFETs M₃ and M₄operate in Ohmic mode to play the same role as emitter resistors R_E in Figure 3, and MOSFETs M₁ and M₂operate in active mode in the same roles as mirror transistors Q₁ and Q₂ in Figure 3. An explanation follows of how the circuit in Figure 3 works.

The operational amplifier is fed the difference in voltages V₁ − V₂ at the top of the two emitter-leg resistors of value R_E. This difference is amplified by the op amp and fed to the base of output transistor Q₂. If the collector base reverse bias on Q₂ is increased by increasing the applied voltage V_A, the current in Q₂increases, increasing V₂ and decreasing the difference V₁ − V₂ entering the op amp. Consequently, the base voltage of Q₂ is decreased, and V_BE of Q₂ decreases, counteracting the increase in output current.

If the op-amp gain A_v is large, only a very small difference V₁ − V₂ is sufficient to generate the needed base voltage V_B for Q₂, namely

V_{1}-V_{2}={\frac {V_{B}}{A_{v}}}.

Consequently, the currents in the two leg resistors are held nearly the same, and the output current of the mirror is very nearly the same as the collector current I_C1 in Q₁, which in turn is set by the reference current as

I_{\text{ref}}=I_{C1}\left(1+{\frac {1}{\beta _{1}}}\right),

where β₁ for transistor Q₁ and β₂ for Q₂ differ due to the Early effect if the reverse bias across the collector-base of Q₂ is non-zero.

Output resistance[edit]

Figure 5: Small-signal circuit to determine output resistance of mirror; transistor Q₂ is replaced with its hybrid-pi model; a test current I_X at the output generates a voltage V_X, and the output resistance is R_out = V_X / I_X.

An idealized treatment of output resistance is given in the footnote.^{[nb 2]} A small-signal analysis for an op amp with finite gain A_v but otherwise ideal is based upon Figure 5 (β, r_O and r_π refer to Q₂). To arrive at Figure 5, notice that the positive input of the op amp in Figure 3 is at AC ground, so the voltage input to the op amp is simply the AC emitter voltage V_e applied to its negative input, resulting in a voltage output of −A_vV_e. Using Ohm's law across the input resistance r_π determines the small-signal base current I_b as:

I_{b}={\frac {V_{e}}{\frac {r_{\pi }}{A_{v}+1}}}\ .

Combining this result with Ohm's law for $R_E$ , $V_{e}$ can be eliminated, to find:^{[nb 3]}

I_{b}=I_{X}{\frac {R_{E}}{R_{E}+{\frac {r_{\pi }}{A_{v}+1}}}}.

Kirchhoff's voltage law from the test source I_X to the ground of R_E provides:

V_{X}=(I_{X}+\beta I_{b})r_{O}+(I_{X}-I_{b})R_{E}.

Substituting for I_b and collecting terms the output resistance R_out is found to be:

R_{\text{out}}={\frac {V_{X}}{I_{X}}}=r_{O}\left(1+\beta {\frac {R_{E}}{R_{E}+{\frac {r_{\pi }}{A_{v}+1}}}}\right)+R_{E}\|{\frac {r_{\pi }}{A_{v}+1}}.

For a large gain A_v ≫ r_π / R_E the maximum output resistance obtained with this circuit is

R_{\text{out}}=(\beta +1)r_{O},

a substantial improvement over the basic mirror where R_out = r_O.

The small-signal analysis of the MOSFET circuit of Figure 4 is obtained from the bipolar analysis by setting β = g_m r_π in the formula for R_out and then letting r_π → ∞. The result is

R_{\text{out}}=r_{O}\left[1+g_{m}R_{E}(A_{v}+1)\right]+R_{E}.

This time, R_E is the resistance of the source-leg MOSFETs M₃, M₄. Unlike Figure 3, however, as A_v is increased (holding R_E fixed in value), R_out continues to increase, and does not approach a limiting value at large A_v.

Compliance voltage[edit]

For Figure 3, a large op amp gain achieves the maximum R_out with only a small R_E. A low value for R_E means V₂ also is small, allowing a low compliance voltage for this mirror, only a voltage V₂ larger than the compliance voltage of the simple bipolar mirror. For this reason this type of mirror also is called a wide-swing current mirror, because it allows the output voltage to swing low compared to other types of mirror that achieve a large R_out only at the expense of large compliance voltages.

With the MOSFET circuit of Figure 4, like the circuit in Figure 3, the larger the op amp gain A_v, the smaller R_E can be made at a given R_out, and the lower the compliance voltage of the mirror.

Other current mirrors[edit]

There are many sophisticated current mirrors that have higher output resistances than the basic mirror (more closely approach an ideal mirror with current output independent of output voltage) and produce currents less sensitive to temperature and device parameter variations and to circuit voltage fluctuations. These multi-transistor mirror circuits are used both with bipolar and MOS transistors. These circuits include:

the Widlar current source
the Wilson current mirror used as a current source
Cascoded current sources

Notes[edit]

^ Keeping the output resistance high means more than keeping the MOSFET in active mode, because the output resistance of real MOSFETs only begins to increase on entry into the active region, then rising to become close to maximum value only when V_DG ≥ 0 V.
^ An idealized version of the argument in the text, valid for infinite op amp gain, is as follows. If the op amp is replaced by a nullor, voltage V₂ = V₁, so the currents in the leg resistors are held at the same value. That means the emitter currents of the transistors are the same. If the V_CB of Q₂ increases, so does the output transistor β because of the Early effect: β = β₀(1 + V_CB / V_A). Consequently the base current to Q₂ given by I_B = I_E / (β + 1) decreases and the output current I_out = I_E / (1 + 1 / β) increases slightly because β increases slightly. Doing the math,
${\begin{aligned}{\frac {1}{R_{\text{out}}}}&={\frac {\partial I_{\text{out}}}{\partial V_{CB}}}=I_{E}\cdot {\frac {\partial }{\partial V_{CB}}}\left({\frac {\beta }{\beta +1}}\right)=I_{E}\cdot {\frac {1}{(\beta +1)^{2}}}\cdot {\frac {\partial \beta }{\partial V_{CB}}}\\&={\frac {\beta I_{E}}{\beta +1}}\cdot {\frac {1}{\beta }}\cdot {\frac {\beta _{0}}{V_{A}}}\cdot {\frac {1}{\beta +1}}\\&=I_{\text{out}}\cdot {\frac {1}{1+{\frac {V_{CB}}{V_{A}}}}}\cdot {\frac {1}{V_{A}}}\cdot {\frac {1}{\beta +1}}\\&={\frac {1}{(\beta +1)r_{0}}},\end{aligned}}$
where the transistor output resistance is given by r_O = (V_A + V_CB) / I_out. That is, the ideal mirror resistance for the circuit using an ideal op amp nullor is R_out = (β + 1c)r_O, in agreement with the value given later in the text when the gain → ∞.
^ As A_v → ∞, V_e → 0 and I_b → I_X.

Nikiya Anton Bettey 2021

Blog Archive

Tuesday, September 21, 2021

09-21-2021-1235 - Ground Earth Ground Plane

Mirror characteristics[edit]

Practical approximations[edit]

Circuit realizations of current mirrors[edit]

Basic idea[edit]

Basic BJT current mirror[edit]

Output resistance[edit]

Compliance voltage[edit]

Extensions and complications[edit]

Basic MOSFET current mirror[edit]

Output resistance[edit]

Compliance voltage[edit]

Extensions and reservations[edit]

Feedback-assisted current mirror[edit]

Output resistance[edit]

Compliance voltage[edit]

Other current mirrors[edit]

Notes[edit]

See also[edit]

No comments:

Post a Comment