Phonons can scatter through several mechanisms as they travel through the material. These scattering mechanisms are: Umklapp phonon-phonon scattering, phonon-impurity scattering, phonon-electron scattering, and phonon-boundary scattering. Each scattering mechanism can be characterised by a relaxation rate 1/ which is the inverse of the corresponding relaxation time.
All scattering processes can be taken into account using Matthiessen's rule. Then the combined relaxation time can be written as:
The parameters , , , are due to Umklapp scattering, mass-difference impurity scattering, boundary scattering and phonon-electron scattering, respectively.
Phonon-phonon scattering[edit]
For phonon-phonon scattering, effects by normal processes (processes which conserve the phonon wave vector - N processes) are ignored in favor of Umklapp processes (U processes). Since normal processes vary linearly with and umklapp processes vary with , Umklapp scattering dominates at high frequency.[1] is given by:
where is the Gruneisen anharmonicity parameter, μ is the shear modulus, V0 is the volume per atom and is the Debye frequency.[2]
Three-phonon and four-phonon process[edit]
Thermal transport in non-metal solids was usually considered to be governed by the three-phonon scattering process,[3] and the role of four-phonon and higher-order scattering processes was believed to be negligible. Recent studies have shown that the four-phonon scattering can be important for nearly all materials at high temperature [4] and for certain materials at room temperature.[5][6] The predicted significance of four-phonon scattering in boron arsenide was confirmed by experiments.[7]
Mass-difference impurity scattering[edit]
Mass-difference impurity scattering is given by:
where is a measure of the impurity scattering strength. Note that is dependent of the dispersion curves.
Boundary scattering[edit]
Boundary scattering is particularly important for low-dimensional nanostructures and its relaxation time is given by:
where is the characteristic length of the system and , which is related to the roughness of the surface, represents the fraction of specularly scattered phonons. The parameter is not easily calculated for an arbitrary surface. For a surface characterized by a root-mean-square roughness , a wavelength-dependent value for the parameter can be calculated using
in the case of plane waves at normal incidence.[8] The value corresponds to a perfectly smooth surface such that boundary scattering is purely specular. The relaxation time is in this case infinite, implying that boundary scattering does not contribute to the thermal resistance. Conversely, the value corresponds to a very rough surface, in which case boundary scattering is purely diffusive and the relaxation rate is given by:
This equation is also known as Casimir limit.[9]
Phonon-electron scattering[edit]
Phonon-electron scattering can also contribute when the material is heavily doped. The corresponding relaxation time is given as:
The parameter is conduction electrons concentration, ε is deformation potential, ρ is mass density and m* is effective electron mass.[2] It is usually assumed that contribution to thermal conductivity by phonon-electron scattering is negligible.
See also[edit]
References[edit]
- ^ Mingo, N (2003). "Calculation of nanowire thermal conductivity using complete phonon dispersion relations". Physical Review B. 68 (11): 113308. arXiv:cond-mat/0308587. Bibcode:2003PhRvB..68k3308M. doi:10.1103/PhysRevB.68.113308.
- ^ a b Zou, Jie; Balandin, Alexander (2001). "Phonon heat conduction in a semiconductor nanowire" (PDF). Journal of Applied Physics. 89 (5): 2932. Bibcode:2001JAP....89.2932Z. doi:10.1063/1.1345515. Archived from the original (PDF) on 2010-06-18.
- ^ Ziman, J.M. (1960). Electrons and Phonons: The Theory of transport phenomena in solids. Oxford Classic Texts in the Physical Sciences. Oxford University Press.
- ^ Feng, Tianli; Ruan, Xiulin (2016). "Quantum mechanical prediction of four-phonon scattering rates and reduced thermal conductivity of solids". Physical Review B. 93(4): 045202. arXiv:1510.00706. Bibcode:2016PhRvB..96p5202F. doi:10.1103/PhysRevB.93.045202.
- ^ Feng, Tianli; Lindsay, Lucas; Ruan, Xiulin (2017). "Four-phonon scattering significantly reduces intrinsic thermal conductivity of solids". Physical Review B. 96 (16): 161201. Bibcode:2017PhRvB..96p1201F. doi:10.1103/PhysRevB.96.161201.
- ^ Kundu, Ashis (2021). "Ultrahigh Thermal Conductivity of θ-Phase Tantalum Nitride". Phys. Rev. Lett. 126 (11): 115901. doi:10.1103/PhysRevLett.126.115901.
- ^ Kang, Joon Sang; Li, Man; Wu, Huan; Nguyen, Huuduy; Hu, Yongjie (2018). "Experimental observation of high thermal conductivity in boron arsenide". Science. 361 (6402): 575–578. Bibcode:2018Sci...361..575K. doi:10.1126/science.aat5522. PMID 29976798.
- ^ Ziman, John M. (2001). Electrons and Phonons: The Theory of Transport Phenomena in Solids. Oxford University Press. pp. 459. doi:10.1093/acprof:oso/9780198507796.003.0011.
- ^ Casimir, H.B.G (1938). "Note on the Conduction of Heat in Crystals". Physica. 5 (6): 495–500. Bibcode:1938Phy.....5..495C. doi:10.1016/S0031-8914(38)80162-2.
https://en.wikipedia.org/wiki/Phonon_scattering
In crystalline materials, Umklapp scattering (also U-process or Umklapp process) is a scattering process that results in a wave vector (usually written k) which falls outside the first Brillouin zone. If a material is periodic, it has a Brillouin zone, and any point outside the first Brillouin zone can also be expressed as a point inside the zone. So, the wave vector is then mathematically transformed to a point inside the first Brillouin zone. This transformation allows for scattering processes which would otherwise violate the conservation of momentum: two wave vectors pointing to the right can combine to create a wave vector that points to the left. This non-conservation is why crystal momentum is not a true momentum.
Examples include electron-lattice potential scattering or an anharmonic phonon-phonon (or electron-phonon) scattering process, reflecting an electronic state or creating a phonon with a momentum k-vector outside the first Brillouin zone. Umklapp scattering is one process limiting the thermal conductivity in crystalline materials, the others being phonon scattering on crystal defects and at the surface of the sample.
Figure 1 schematically shows the possible scattering processes of two incoming phonons with wave-vectors (k-vectors) k1 and k2 (red) creating one outgoing phonon with a wave vector k3 (blue). As long as the sum of k1 and k2stay inside the first Brillouin zone (grey squares), k3 is the sum of the former two, thus conserving phonon momentum. This process is called normal scattering (N-process).
With increasing phonon momentum and thus larger wave vectors k1 and k2, their sum might point outside the first Brillouin zone (k'3). As shown in Figure 2, k-vectors outside the first Brillouin zone are physically equivalent to vectors inside it and can be mathematically transformed into each other by the addition of a reciprocal lattice vector G. These processes are called Umklapp scattering and change the total phonon momentum.
Umklapp scattering is the dominant process for electrical resistivity at low temperatures for low defect crystals[1] (as opposed to phonon-electron scattering, which dominates at high temperatures, and high-defect lattices which lead to scattering at any temperature.)
Umklapp scattering is the dominant process for thermal resistivity at high temperatures for low defect crystals.[citation needed] The thermal conductivity for an insulating crystal where the U-processes are dominant has 1/T dependence.
The name derives from the German word umklappen (to turn over). Rudolf Peierls, in his autobiography Bird of Passage states he was the originator of this phrase and coined it during his 1929 crystal lattice studies under the tutelage of Wolfgang Pauli. Peierls wrote, "…I used the German term Umklapp (flip-over) and this rather ugly word has remained in use…."[2]
See also[edit]
https://en.wikipedia.org/wiki/Umklapp_scattering
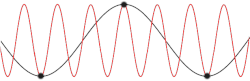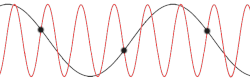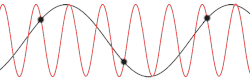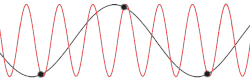
The Nyquist–Shannon sampling theorem is a theorem in the field of signal processing which serves as a fundamental bridge between continuous-time signals and discrete-time signals. It establishes a sufficient condition for a sample rate that permits a discrete sequence of samples to capture all the information from a continuous-time signal of finite bandwidth.
Strictly speaking, the theorem only applies to a class of mathematical functions having a Fourier transform that is zero outside of a finite region of frequencies. Intuitively we expect that when one reduces a continuous function to a discrete sequence and interpolates back to a continuous function, the fidelity of the result depends on the density (or sample rate) of the original samples. The sampling theorem introduces the concept of a sample rate that is sufficient for perfect fidelity for the class of functions that are band-limited to a given bandwidth, such that no actual information is lost in the sampling process. It expresses the sufficient sample rate in terms of the bandwidth for the class of functions. The theorem also leads to a formula for perfectly reconstructing the original continuous-time function from the samples.
Perfect reconstruction may still be possible when the sample-rate criterion is not satisfied, provided other constraints on the signal are known (see § Sampling of non-baseband signals below and compressed sensing). In some cases (when the sample-rate criterion is not satisfied), utilizing additional constraints allows for approximate reconstructions. The fidelity of these reconstructions can be verified and quantified utilizing Bochner's theorem.[1]
The name Nyquist–Shannon sampling theorem honours Harry Nyquist and Claude Shannon, but the theorem was also previously discovered by E. T. Whittaker(published in 1915) and Shannon cited Whittaker's paper in his work. The theorem is thus also known by the names Whittaker–Shannon sampling theorem, Whittaker–Shannon, and Whittaker–Nyquist–Shannon, and may also be referred to as the cardinal theorem of interpolation.
https://en.wikipedia.org/wiki/Nyquist–Shannon_sampling_theorem
Subcategories
This category has the following 3 subcategories, out of 3 total.
A
R
S
Pages in category "Scattering, absorption and radiative transfer (optics)"
The following 94 pages are in this category, out of 94 total. This list may not reflect recent changes (learn more).



























No comments:
Post a Comment