In differential geometry, an osculating curve is a plane curve from a given family that has the highest possible order of contact with another curve. That is, if F is a family of smooth curves, C is a smooth curve (not in general belonging to F), and p is a point on C, then an osculating curve from F at p is a curve from F that passes through p and has as many of its derivatives at p equal to the derivatives of C as possible.[1][2]
The term derives from the Latinate root "osculate", to kiss, because the two curves contact one another in a more intimate way than simple tangency.[3]
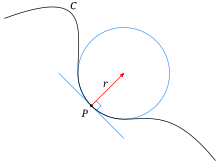
A curve C containing a point P where the radius of curvature equals r, together with the tangent line and the osculating circle touching C at P
https://en.wikipedia.org/wiki/Osculating_curve
No comments:
Post a Comment